If αandβ are the solution of acosθ+bsinθ=c, then Solution in Punjabi
sinα+sinβ=2bca2+b2
sinα+sinβ=c2−a2a2+b2
sinα+sinβ=2acb2+c2
sinα+sinβ=ca2+b2
The correct Answer is:A, B
Step by step video & image solution for If alpha and beta are the solution of a cos theta + b sin theta = c, then by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise (Passage Based Questions)13videos
TRIGONOMETRIC EQUATIONS AND INEQUATIONS
- BOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Trigonometric Equations and Inequations Exercise 3 :Passage Based Questions1videos
TRIGONOMETRIC EQUATIONS AND INEQUATIONS
- BOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise (Single Option Correct Type Questions)60videos
TRIGONOMETRIC EQUATIONS AND INEQUATIONS
- BOOK - ARIHANT MATHSCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Three Dimensional Coordinate System Exercise 12 : Question Asked in Previous Years Exam2videos
THREE DIMENSIONAL COORDINATE SYSTEM
- BOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)18videos
TRIGONOMETRIC FUNCTIONS AND IDENTITIES
Similar Questions
If number of solution and sum of solution of the equation 3sin2x−7sinx+2=0,x∈[0,2π] are respectively N and S and fn(θ)=sinnθ+cosnθ. On the basis of above information , answer the following questions.
Value of N is06:16View SolutionIf an angle and a side of a right angle triangle is known , then rest of the sides and angles can be found as follows
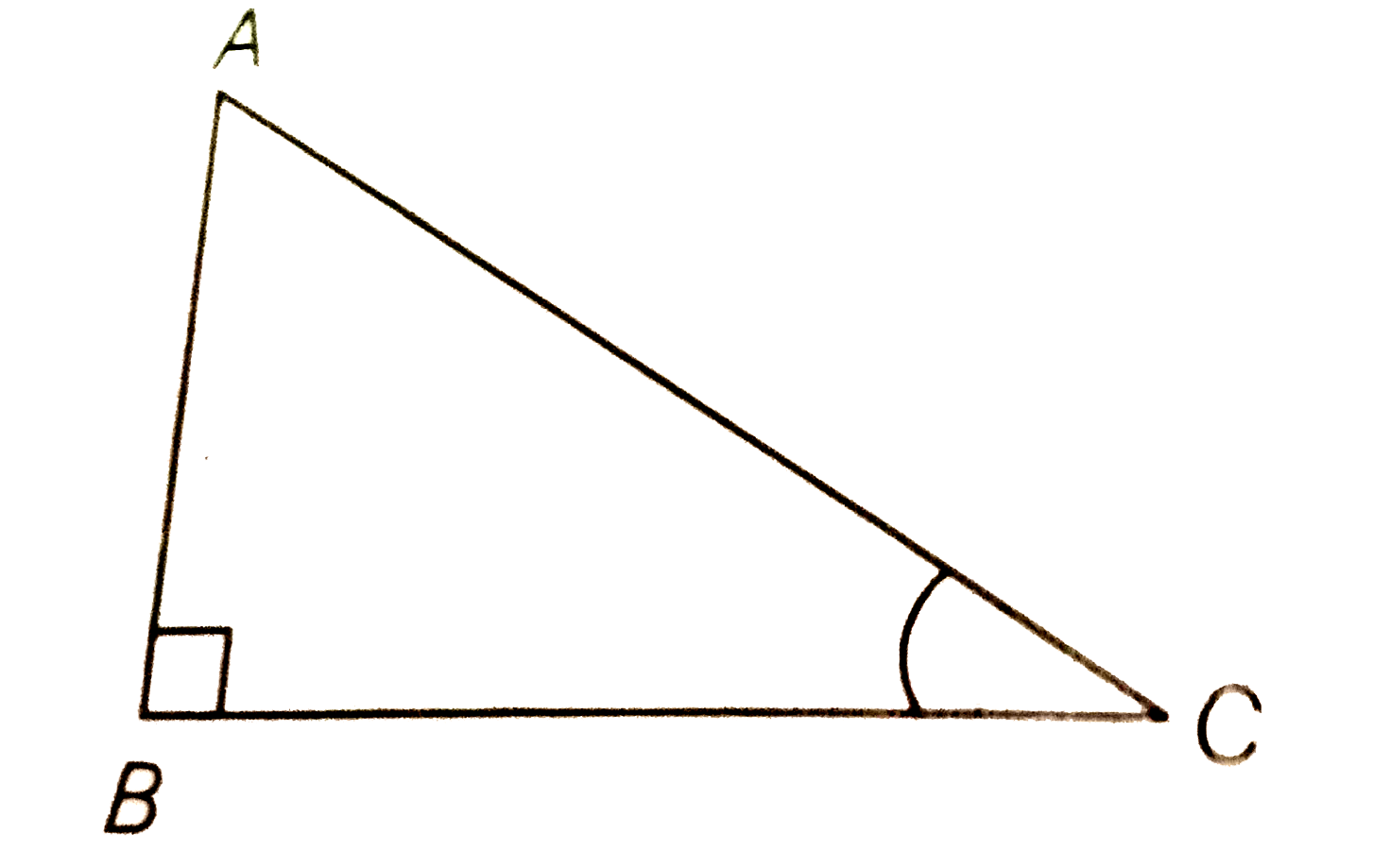
In ΔABC(figure ), if ∠B=90∘,∠C=θandBC=x, then AB=xtanθandAC=xsecθ .
Now, consider in isosceles triangle PQR (Figure 2),
Where PQ=PR and 20N=√3
On the basis of the above information answer the question
The angle of triangle PQR are05:59View SolutionShow that sinx−sinycosx+cosy=tan(x−y2)
03:07View SolutionConsider the line L1:x−12=y−1=z+31,L2:x−41=y+31=z+32 find the angle between them.
02:05View SolutionLet αandβ be non-zero real numbers such that 2(cosβ−cosα)+cosαcosβ=1. Then which of the following is/are true ?
03:41View SolutionLet sin(θ−α)sin(θ−β)=abandcos(θ−α)cos(θ−β)=cdthenac+bdad+bc= (a)cos(α−β) (b) sin(α−β) sin(α+β) (d) none of these
08:40View SolutionIf alpha and beta are the solutions of a cos theta+b sin theta=c, show that sin alpha+sin beta=(2bc)/(a^(2)+b^(2)) and sin alpha sin beta=(c^(2)-a^(2))/(a^(2)+b^(2))
02:20View SolutionUnder rotation of axes through theta , x cosalpha + ysinalpha=P changes to Xcos beta + Y sin beta=P then . (a) cos beta = cos (alpha - theta) (b) cos alpha= cos( beta - theta) (c) sin beta = sin (alpha - theta) (d) sin alpha = sin ( beta - theta)
04:33View SolutionIf alpha,beta are roots of a cos theta+b sin theta=c then value of sin alpha+sin beta&sin alpha sin beta is
04:02View Solutionalpha& beta are solutions of a cos theta+b sin theta=c(cos alpha!=cos beta)&(sin alpha!=sin beta) Then tan((alpha+beta)/(2))=?
06:46View SolutionLet sin(θ−α)sin(θ−β)=abandcos(θ−α)cos(θ−β)=cdthenac+bdad+bc= (a)cos(α−β) (b) sin(α−β) sin(α+β) (d) none of these
07:37View SolutionUnder rotation of axes through θ , xcosα+ysinα=P changes to Xcosβ+Ysinβ=P then . (a) cosβ=cos(α−θ) (b) cosα=cos(β−θ) (c) sinβ=sin(α−θ) (d) sinα=sin(β−θ)
05:24View SolutionIf cosα+cosβ=a,sinα+sinβ=bandα−β=2θ. Then cos3θcosθ=
View SolutionIf α,β are solutions of acosθ+bsinθ=c where a, b, c∈R and a2+b2>0,cosα≠cosβ,sinα≠sinβ then prove that
cosαcosβ=c2−b2a2+b203:53View SolutionLet sin(θ−α)sin(θ−β)=ab and cos(θ−α)cos(θ−β)=cd then ac+bdad+bc=
04:06View SolutionIf sin(θ+α)=aandsin(θ+β)=b,thencos2(α−β)−4abcos(α−β)=
07:29View Solution

