If α,β,γ are the zeros of the polynomial f(x)=ax3+bx2+cx+d then (α)2+(β)2+(γ)2 = ………….Solution in Telugu
b2+4ac2
b2−2aca2
b+2aca2
none
Step by step video solution for If alpha, beta, gamma are the zeros of the polynomial f(x) = ax^(3) + bx^(2) + cx + d then (alpha)^2 + (beta)^2 + (gamma)^2 = …………. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- POLYNOMIALSBOOK - BEYOND PUBLICATIONCHAPTER - POLYNOMIALSEXERCISE - EXERCISE
56 Videos
- PAIR OF LINEAR EQUATIONS IN TWO VARIABLESBOOK - BEYOND PUBLICATIONCHAPTER - PAIR OF LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - EXERCISE
174 Videos
- PROBABILITYBOOK - BEYOND PUBLICATIONCHAPTER - PROBABILITYEXERCISE - EXERCISE
136 Videos
Similar Questions
If p(x) = 2x5−6x4+3x−8, find
coefficient of x
View SolutionBy comparing the ratios a1a2,b1b2,c1c2, find out whether the represented by the following pairs of linear equations intersect at a point, are parallel or are coincident. 6x-3y+10=0 , 2x-y+9=0.
View SolutionCards marked with numbers 13,14,15…………,60 are placed in a box and mixed throughly. One card is drawn at random from the box. Find the probability that number on the card drawn is
divisible by 5.
View SolutionIf alpha,beta,gamma are the roots of the equation ax^(3)+bx^(2)+cx+d=0 then find the value of sum(alpha^(2)(beta+gamma))
View Solutionयदि α,β,γ बहुपद f(x)=ax3+bx2+cx+d के मूल है तब 1α+1β+1γ=
View Solutionयदि α,β,γ बहुपद f(x)=ax3+bx2+cx+d के मूल है तब α2+β2+γ2=???
View Solutionयदि α,β,γ बहुपद f(x)=ax3+bx2+cx+d के शून्यक हो , तो 1α+1β+1γ=
View Solutionयदि α,β,γ बहुपद f(x)=ax3+bx2+cx+d के शून्यक हो, तो α2+β2+γ2=
View Solutionयदि बहुपद cx2+ax2+bx+a के शून्यक α,β,γ हो तो αβγ का मान होगा
View Solutionयदि बहुपद dx3+cx2+bx+a के शून्यक α,β,γहों तो αβγका मान होगा
View Solutionरिक्त स्थान की पूर्ति करें -
यदि α,β,γ बहुपद ax3+bx2+cx+d के शून्यक हों, तो αβ+βγ+αγ= …….. .
View Solutionयदि बहुपद p(x)=x3+bx2+cx+d के शून्यक αβ और γ हों ताकि α+β+γ=−4,αβ+βγ+αγ=3 और αβγ=5,तो p(x) ज्ञात कीजिए ।
View SolutionIf α,β,γ are the zeroes of the cubic polynomial ax3+bx2+cx+d and (a≠0) , then αβγ = ……….
View SolutionIf α,β,γ are the zeros of the polynomial f(x)=ax3+bx2+cx+d then 1α+1β+1γ = ………….
View SolutionIf α,β,γ are the zeros of the polynomial f(x)=ax3+bx2+cx+d then (α)2+(β)2+(γ)2 = ………….
View SolutionMatch the following :
If α,β,γ are zeroes of a cubic polynomial
ax3+bx2+cx+d(a≠0), then………
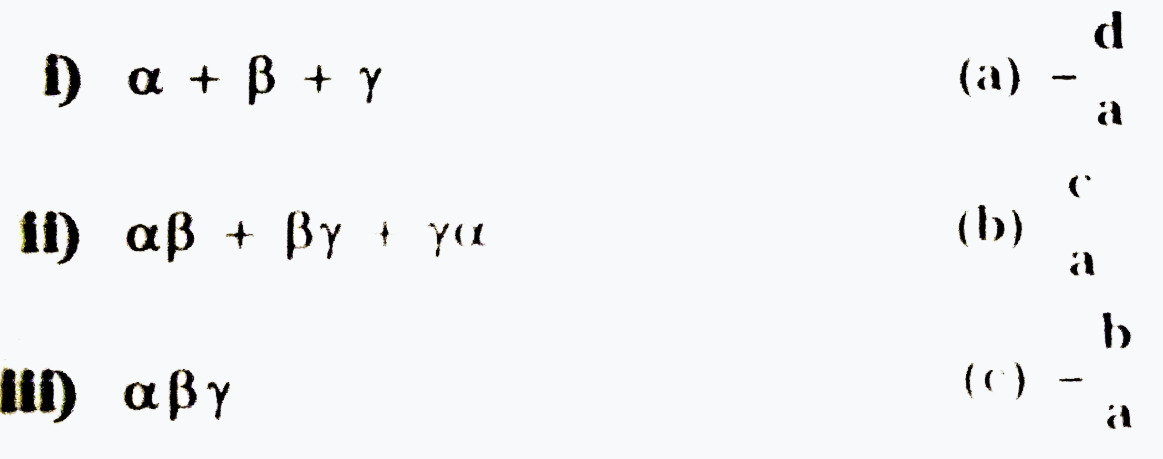
View Solution
