If AOB is a diameter of the circle and AC=BC, then ∠CAB is equal to
A
30∘
B
45∘
C
60∘
D
90∘
Video Solution
Text Solution
The correct Answer is:B
We know that, diameter subtends a right angle to the circle.
∴∠BCA=90∘...(i)
Given,AC=BC
⇒∠ABC=∠CAB...(ii)
[angles opposite to equal sides are equal]
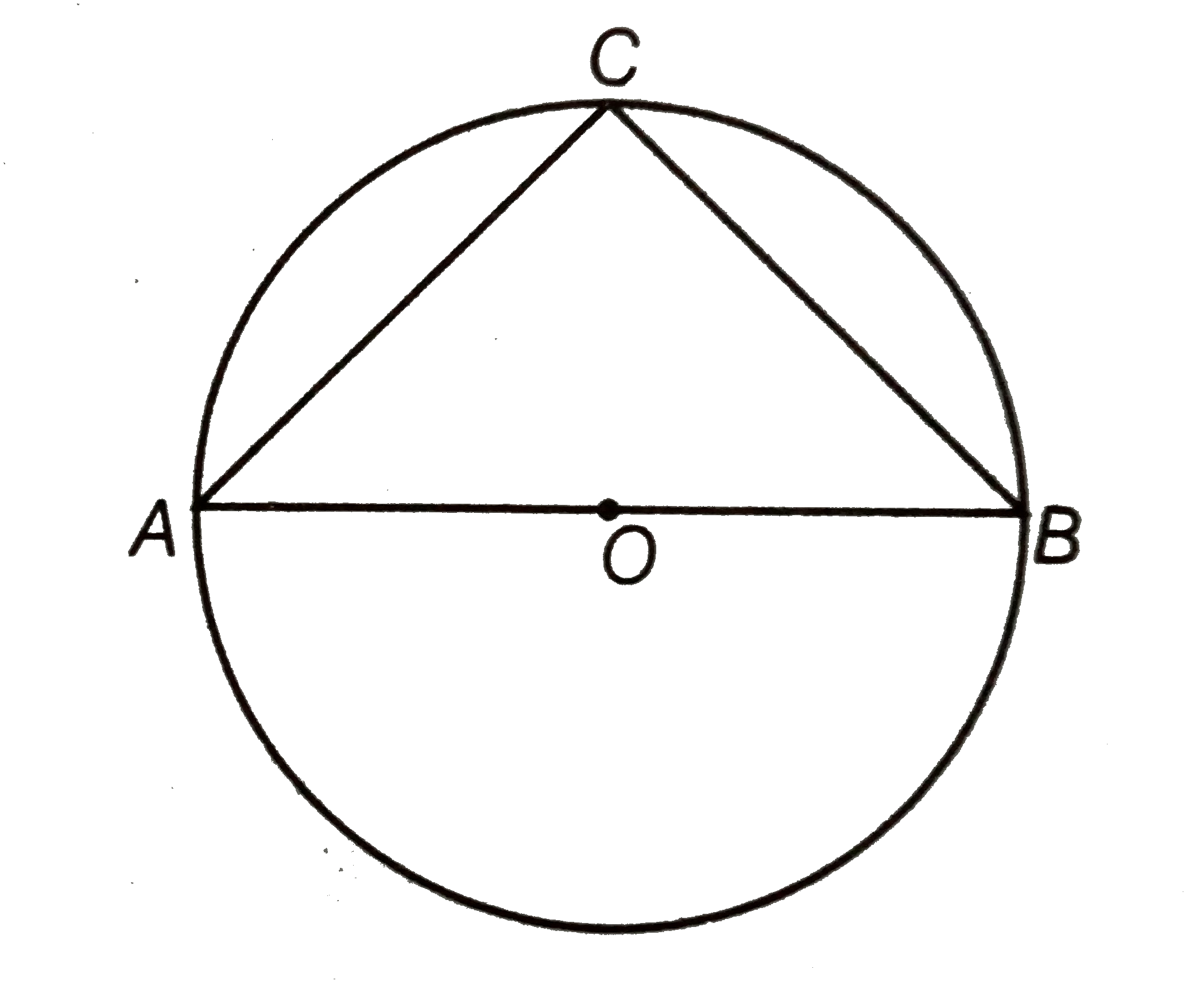
In ΔABC,∠CAB+∠ABC+∠BCA=180∘
[by angle sum property of a triangle]
⇒∠CAB+∠CAB+∠90∘=180∘[form Eqs. (i) and (ii)]
⇒2∠CAB=180∘−90∘
⇒∠CAB=90∘2
∴∠CAB=45∘
|
Topper's Solved these Questions
- CIRCLESBOOK - NCERT EXEMPLARCHAPTER - CIRCLESEXERCISE - Exercise 10.4
2 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLARCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Areas Of Parallelograms And Triangles
34 Videos
- CONSTRUCTIONSBOOK - NCERT EXEMPLARCHAPTER - CONSTRUCTIONSEXERCISE - Long Answer Type Questions
5 Videos
Similar Questions
Knowledge Check
In figure, BC is a diameter of the circle and ∠BAO=60∘. Then,∠ADCis equal to
 A60∘B45∘C50∘D120∘
A60∘B45∘C50∘D120∘AB is a diameter of the circle with centre O and chord CD is equal to radius OC (fig). AC and BD proudced meet at P. Prove that ∠CPD=60∘.
 A60∘B30∘C90∘D160∘
A60∘B30∘C90∘D160∘If the bisector of the angles A and B of a quadrilateral ABCD meet at O, then ∠AOB is equal to:
A∠C+∠DB12(∠C+∠D)C12∠C+13∠DD13∠C+12∠D
In the given figure, AOB is a diameter of a circle and CD||AB. If ∠BAD=30∘, then ∠CAD = ?
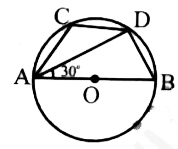
View SolutionIn the adjoining figure, AOB is the diameter of the circle. If ∠ABP=45∘, then find the value of ∠PQB.

View SolutionIn the adjoining figure, ABCD is a cyclic quadrilateral and AB is the diameter of the circle. If ∠APC=120∘, then find the value of ∠CAB.
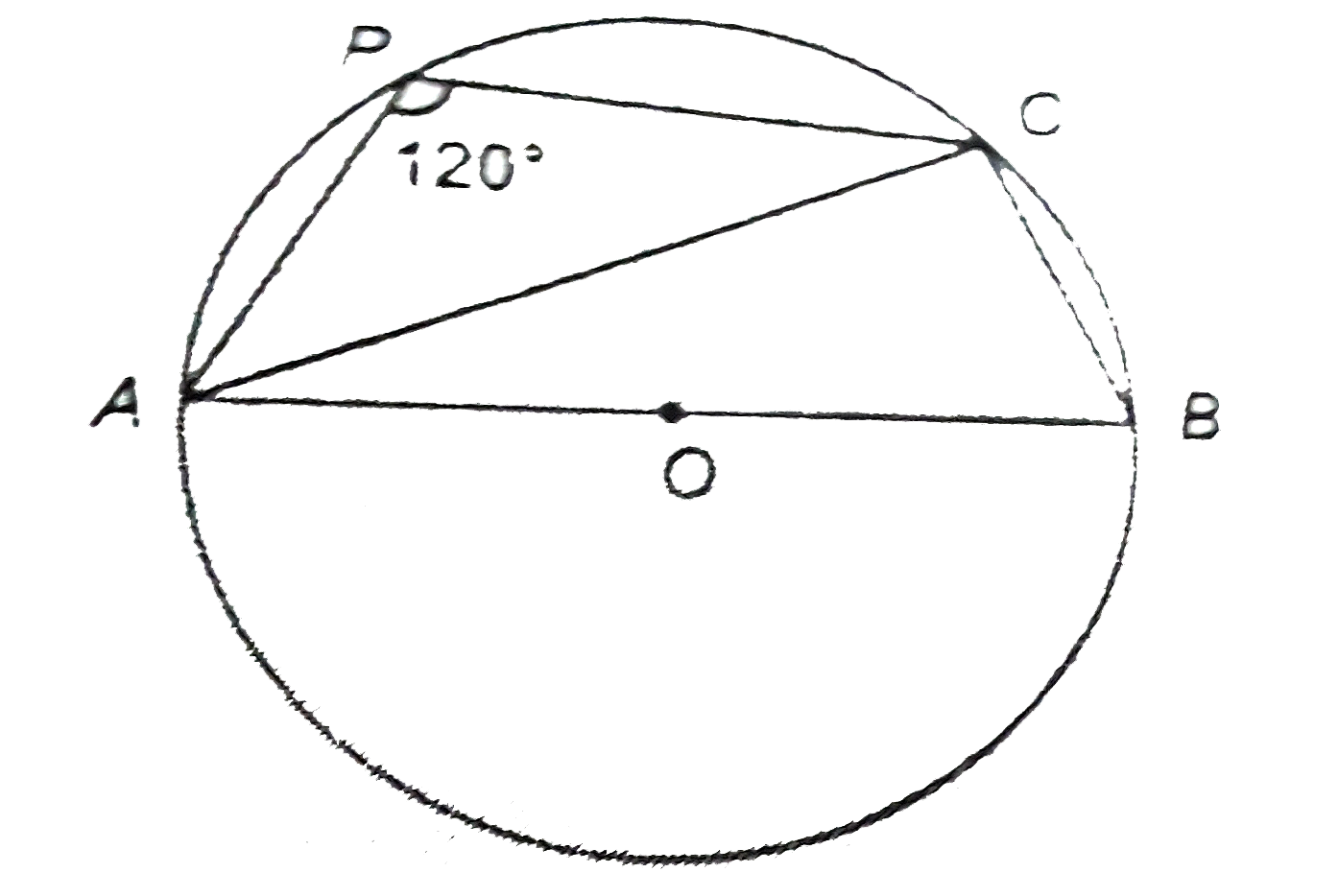
View SolutionIf AOB is a diameter of a circle and C is a point on the circle, then AC2+BC2=AB2.
View SolutionIn a circle with centre O, AOC is a diameter of the circle, BD is a chord and OB and CD are joined. If ∠AOB =130∘, then ∠BDC is equal to
View Solution