If APB and CQD are two parallel lines, then the bisectors of the angles APQ,BPQ,CQP and PQD form
a square
a rhombus
a rectangle
any other parallelogram
The correct Answer is:C
To solve the problem, we need to analyze the given information about the parallel lines and the angle bisectors. Here’s a step-by-step solution:
Step 1: Understand the Configuration
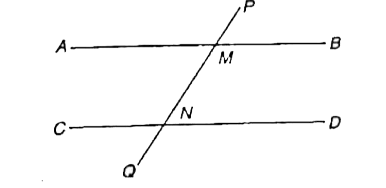
We have two parallel lines, APB and CQD. The angles formed by these lines with a transversal line PQ create angles APQ, BPQ, CQP, and PQD.
Hint: Visualize the parallel lines and the transversal to understand the angles formed.
Step 2: Identify the Angle Bisectors
Let’s denote the angle bisectors of the angles:
- The bisector of angle APQ as MP
- The bisector of angle BPQ as NP
- The bisector of angle CQP as MQ
- The bisector of angle PQD as NQ
Hint: Remember that the angle bisector divides the angle into two equal parts.
Step 3: Establish Relationships Between Angles
Since MP is the bisector of angle APQ, we have:
- Angle 1 = Angle 5 (where angle 1 is part of angle APQ and angle 5 is formed by the bisector)
Similarly, we can establish:
- Angle 2 = Angle 6
- Angle 3 = Angle 7
- Angle 4 = Angle 8
Hint: Use the property of angle bisectors to write down equalities for the angles.
Step 4: Use the Properties of Parallel Lines
Since APB and CQD are parallel, we can use the Alternate Interior Angles Theorem:
- Angle APQ (angle 1 + angle 5) = Angle DQK (angle 4 + angle 8)
This means:
- Angle 1 + Angle 5 = Angle 4 + Angle 8
Using the relationships established in Step 3, we can say:
- Angle 1 = Angle 4 and Angle 2 = Angle 3
Hint: Recall that alternate angles are equal when two lines are parallel.
Step 5: Establish Parallelism of Bisectors
From the equal angles, we can conclude:
- Since angle 1 = angle 4, the lines MP and NQ are parallel.
- Since angle 2 = angle 3, the lines MP and MQ are also parallel.
Hint: Use the properties of angles to determine the relationships between the lines.
Step 6: Determine the Shape Formed
Since we have established that:
- MP is parallel to NQ
- MQ is parallel to PN
This indicates that the figure formed by the bisectors is a parallelogram.
Step 7: Check for Right Angles
To determine if the parallelogram is a rectangle, we need to check if any angles are right angles. Since the angles formed by the transversal with the parallel lines sum up to 180 degrees, we can conclude that:
- Angle 3 + Angle 4 = 90 degrees.
Thus, the angles formed by the bisectors are right angles, confirming that the parallelogram is a rectangle.
Hint: Remember that a rectangle is a special type of parallelogram where all angles are right angles.
Conclusion
Therefore, the bisectors of the angles APQ, BPQ, CQP, and PQD form a rectangle.
Final Answer: The bisectors form a rectangle.
We have two parallel lines, APB and CQD. The angles formed by these lines with a transversal line PQ create angles APQ, BPQ, CQP, and PQD.
Let’s denote the angle bisectors of the angles:
- The bisector of angle APQ as MP
- The bisector of angle BPQ as NP
- The bisector of angle CQP as MQ
- The bisector of angle PQD as NQ
Since MP is the bisector of angle APQ, we have:
- Angle 1 = Angle 5 (where angle 1 is part of angle APQ and angle 5 is formed by the bisector)
- Angle 2 = Angle 6
- Angle 3 = Angle 7
- Angle 4 = Angle 8
Since APB and CQD are parallel, we can use the Alternate Interior Angles Theorem:
- Angle APQ (angle 1 + angle 5) = Angle DQK (angle 4 + angle 8)
- Angle 1 + Angle 5 = Angle 4 + Angle 8
- Angle 1 = Angle 4 and Angle 2 = Angle 3
From the equal angles, we can conclude:
- Since angle 1 = angle 4, the lines MP and NQ are parallel.
- Since angle 2 = angle 3, the lines MP and MQ are also parallel.
Since we have established that:
- MP is parallel to NQ
- MQ is parallel to PN
To determine if the parallelogram is a rectangle, we need to check if any angles are right angles. Since the angles formed by the transversal with the parallel lines sum up to 180 degrees, we can conclude that:
- Angle 3 + Angle 4 = 90 degrees.
Therefore, the bisectors of the angles APQ, BPQ, CQP, and PQD form a rectangle.
Topper's Solved these Questions
Similar Questions
AB and CD are two parallel lines and a transversal 'l' intersects these lines at X and Y respectively Prove that the bisectors of interior angles from a parallelogram whose each angle is 90∘.
View SolutionA transversal intersects two parallel lines. Prove that the bisectors of any pair of corresponding angles so formed are parallel.
View Solution
Knowledge Check
If APB and CQD are two parallel lines then the bisectors of ∠APQ,∠BPQ,∠CQPand∠PQD enclose a
AsquareBrhombusCrectangleDkiteIf a transversal line cuts two parallel lines then bisector of internal angle formed a
ArectangleBsquareCrhombusDparallelogramThe quadrilateral formed by the bisectors of the angles of a parallelogram is a
 ATrapeziumBKiteCRectangleDNone
ATrapeziumBKiteCRectangleDNone
Family of lines continued and angle bisector
View SolutionShow that the bisectors of angles of a parallelogram form a rectangle.
View SolutionAB and CD are two parallel lines and a transversal PO intersects AB and CD at M and N respectively. The bisector of the interior angles form a quadrilateral :
View SolutionTwo parallel lines AB and CD are intersected by a transversal EF at M and N respectively.
The lines MP and NP are the bisectors of interior angles ∠BMN and ∠DNM on the same side of the transversal. Then ∠MPN is equal to :
View SolutionAB and CD are two parallel lines. PQ cuts AB and CD at E and F respectively. EL is the bisector of ∠BEF. If ∠LEB=35∘ ,then ∠CFQ will be :
View Solution
