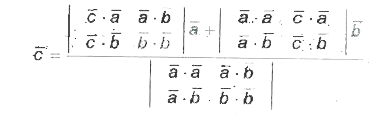
If $¯ a, ¯ b, ¯ c$ are non-coplanar unit vectors each including the angle of measure $30^{\circ}$ with the other, then find the volume of tetrahedron whose co-terminal edges are $¯ a, ¯ b, ¯ c$ .

Video Solution

Text Solution

Verified by Experts

$¯ a, ¯ b ¯ c$ are three non - coplanar unit vectors each including the angle of measure $30^{\circ}$ .
$∴ ¯ a \cdot ¯ a = ¯ b \cdot ¯ b = ¯ c \cdot ¯ c = 1$
$¯ a \cdot ¯ b = ¯ b \cdot ¯ a = 1 \times 1 \times {cos 30}^{\circ} = \frac{\sqrt{3}}{2}$
$¯ a \cdot ¯ c = ¯ c \cdot ¯ a = 1 \times 1 \times {cos 30}^{\circ} = \frac{\sqrt{3}}{2}$
$¯ b \cdot ¯ c = ¯ c \cdot ¯ b = 1 \times 1 \times {cos 30}^{\circ} = \frac{\sqrt{3}}{2}$
Now, volume of tetrahedron $= \frac{1}{6} [¯ a ¯ b ¯ c]$
$∴ {(volume)}^{2} = \frac{1}{36} {([¯ a ¯ b ¯ c])}^{2}$
$= \frac{1}{36} ∣ ∣ ∣ ∣ ∣ \begin{matrix} ¯ a \cdot ¯ a ¯ a \cdot ¯ b ¯ a \cdot ¯ c ¯ b \cdot ¯ a ¯ b \cdot ¯ b ¯ b \cdot ¯ c ¯ c \cdot ¯ a ¯ c \cdot ¯ b ¯ c \cdot ¯ c \end{matrix} ∣ ∣ ∣ ∣ ∣$
$= \frac{1}{36} ∣ ∣ ∣ ∣ ∣ ∣ ∣ \begin{matrix} 1 & \frac{\sqrt{3}}{2} & \frac{\sqrt{3}}{2} \frac{\sqrt{3}}{2} & 1 & \frac{\sqrt{3}}{2} \frac{\sqrt{3}}{2} & \frac{\sqrt{3}}{2} & 1 \end{matrix} ∣ ∣ ∣ ∣ ∣ ∣ ∣$
$= \frac{1}{36} [1 (1 - \frac{3}{4}) - \frac{\sqrt{3}}{2} (\frac{\sqrt{3}}{2} - \frac{3}{4}) + \frac{\sqrt{3}}{2} (\frac{3}{4} - \frac{\sqrt{3}}{2})]$
$= \frac{1}{36} (\frac{3 \sqrt{3}}{4} - \frac{5}{4}) = \frac{3 \sqrt{3} - 5}{144}$
$∴$ volume of tetrahedron $= \frac{\sqrt{3 \sqrt{3} - 5}}{12}$ cu units

|

Updated on:21/07/2023

Class 12 MATHS VECTOR ALGEBRA

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If $¯ a, ¯ b, ¯ c$ are non-coplanar vectors then $[¯ a + 2 ¯ b ¯ a + ¯ c ¯ b] =$
A0
B $[¯ a, ¯ b, ¯ c]$
C $- [¯ a, ¯ b, ¯ c]$
D $2 [¯ a, ¯ b, ¯ c]$

NAVNEET PUBLICATION - MAHARASHTRA BOARD-VECTORS-Multiple choice question

Home
Ask Doubt
Profile