¯a,¯b,¯c are three coplanar vectors, If ¯a is not parallel to ¯b, Show that
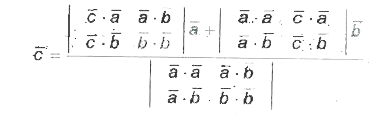
Step by step video & image solution for bar(a),bar(b),bar(c ) are three coplanar vectors, If bar(a) is not parallel to bar(b), Show that by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
¯a,¯b,¯c are three non-coplanar vectors.
If ¯p=¯bׯc¯a⋅(¯bׯc),¯q=¯cׯa¯a⋅(¯bׯc),¯r=¯aׯb¯a⋅(¯bׯc),
show that ¯a⋅¯p+¯b⋅¯q+¯c⋅¯r=3.
View SolutionIf ¯a,¯b,¯c are three non zero vectors, then ¯a.¯b=¯a.¯c⇒
View SolutionLet ¯a,¯b,¯c be three non-coplanar vectors and ¯d be a non-zero vector, which is perpendicularto ¯a+¯b+¯c. Now, if ¯d=(sinx)(¯aׯb)+(cosy)(¯bׯc)+2(¯cׯa) then minimum value of x2+y2 is equal to
View SolutionIf ¯a,¯b,¯c are non coplanar vectors and λ is a real number then [λ(¯a+¯b)λ2¯bλ¯c]=[¯a¯b+¯c¯b] for
View SolutionIf ¯a,¯b,¯c are three non coplanar vectors ¯p=¯bׯc[¯a¯b¯c],¯q=¯cׯa[¯a¯b¯c],¯r=¯aׯb[¯a¯b¯c] then (2¯a+3¯b+4¯c).¯p+(2¯b+3¯c+4¯a)¯q+(2¯c+3¯a+4¯b).¯r=
View SolutionIf ¯a,¯b,¯c are three non coplanar vectors ¯p=(¯bׯc)[¯a¯b¯c],¯q=¯cׯa[¯a¯b¯c],¯r=¯aׯb[¯a¯b¯c] then (2¯a+3¯b+4¯c)⋅¯p+(2¯b+3¯c+4¯a)⋅¯q+(2¯c+3¯a+4¯b)⋅¯r=
View Solutionlet ¯a,¯b&¯c be three non-zero non coplanar vectors and ¯p,¯q&¯r be three vectors defined as ¯p=¯a+¯b−2¯c;¯q=3¯a−2¯b+¯c&¯r=¯a−4¯b+2¯c .If v1,v2 are the volumes of parallelopiped determined by the vectors ¯a,¯b,¯c and ¯p,¯q,¯r respectively then v2:v1 is
View SolutionLet ¯a,¯b,¯c are three non-coplanar vectors such that ¯r1=¯a−¯b+¯c;¯r2=¯b+¯c−¯a;¯r3=¯c+¯a+¯b,¯r=2¯a−3¯b+4¯c.If ¯r=λ1¯r1+λ2¯r2+λ3¯r3 then λ1+λ2+λ3 is equal to
View SolutionIf ¯a,¯b,¯c are non-coplanar unit vectors each including the angle of measure 30∘ with the other, then find the volume of tetrahedron whose co-terminal edges are ¯a,¯b,¯c.
View Solution¯a×(¯bׯc) is parallel to ¯b,then
View Solution
