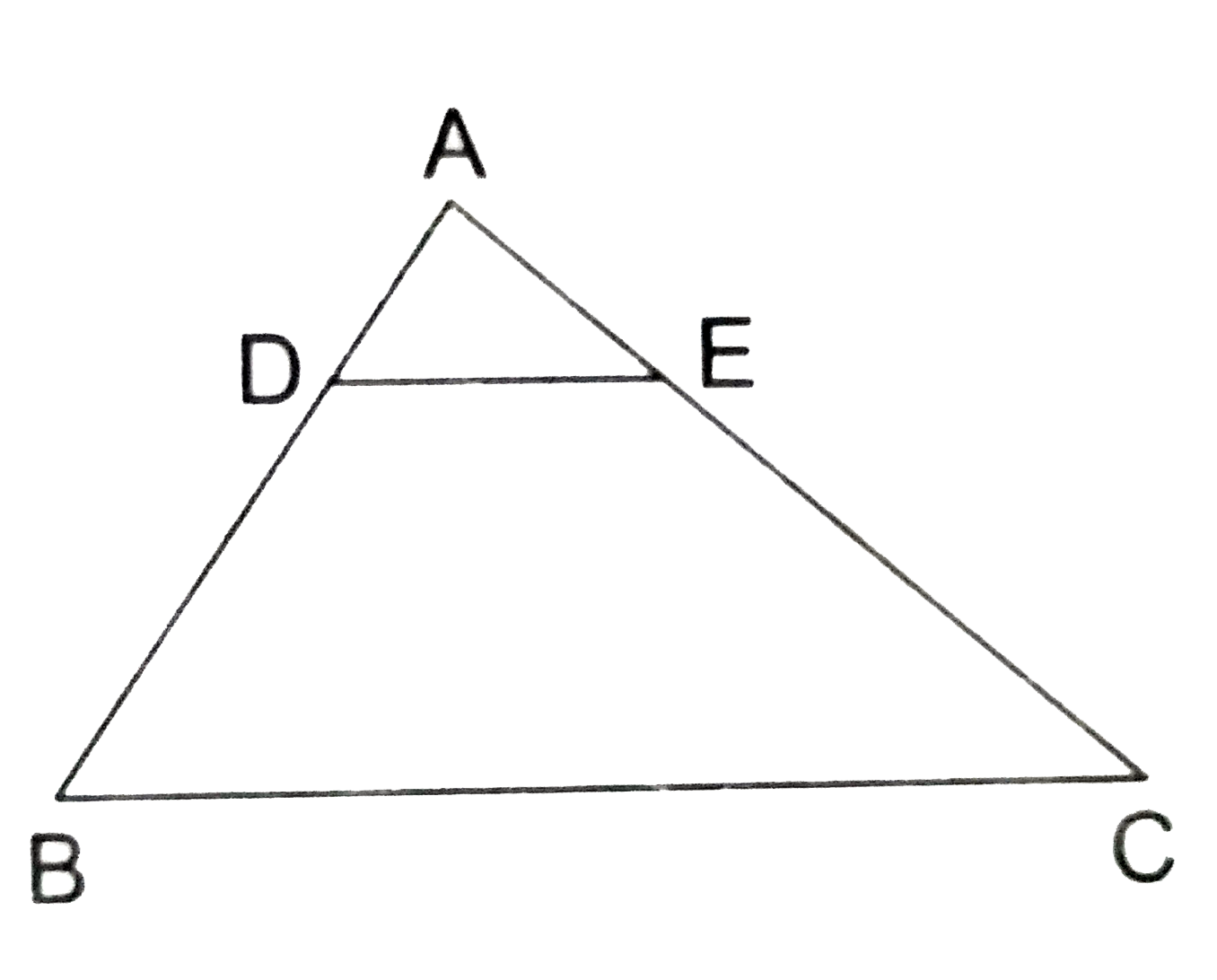
if D and E are points on the sides AB and AC of a $Δ A B C$ . Such that AB=12 cm, AD=8 cm, AE=12 cm, AC= 18 cm. show that DE||BC.

Video Solution

Text Solution

Verified by Experts

Here BD=AB-AD=12-8=4 cm
CE=AC-AE= 18-12=6 cm
Now, $\frac{A D}{B D} = \frac{8}{4} = 2 c m$
and $\frac{A E}{E C} = \frac{12}{6} = 2 c m$
from (1) and (2) , $\frac{A D}{B D} = \frac{A E}{E C}$
DE||BC (converse of Thale's theorem) Hence proved.

|

Updated on:21/07/2023

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Problems From NCERT/ Exemplar
14videos
TRIANGLES
BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Exercise 6a
23videos
TRIANGLES
BOOK - NAGEEN PRAKASHANCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions
5videos
STATISTICS
BOOK - NAGEEN PRAKASHANCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions
5videos
VOLUME AND SURFACE AREA OF SOLIDS

Similar Questions

Knowledge Check

Question 1 - Select One
The points D and E are taken on the sides $A B and A C$ of $Δ A B C$ such that $A D = \frac{1}{3} A B, A E = \frac{1}{3} A C$ . If the length of BC is 15 cm, then the length of DE is :
A10 cm
B8 cm
C6 cm
D5 cm
Question 2 - Select One
D and E are two points on the sides AC and BC respectively of $Δ A B C$ such that $D E = 18 c m, C E = 5 c m$ and $∠ D E C = 90^{\circ}$ . If tan $∠ A B C = 3.6$ , then $A C : C D =$
A $B C : 2 C E$
B $2 C E : B C$
C $2 B C : C E$
D $C E : 2 B C$
Question 3 - Select One
If D and E are points on the sides AB and AC respectively of a triangle ABC such that DE || BC. If AD =x cm, DB =(x-3) cm, AE = ( x+ 3 ) cm and EC = ( x -2) cm, what is the value (in cm. ) of x ?
A3
B3.5
C4
D4.5

NAGEEN PRAKASHAN-TRIANGLES -Revision Exercise Long Questions

Home
Ask Doubt
Profile