If in triangles PQR and XYZ PQXZ=PRXY=QRYZ, then :
ΔPRQ−ΔXZY
ΔQRP−ΔYXZ
ΔPQR−ΔXYZ
ΔPQR−ΔXZY
To solve the problem, we will use the concept of triangle similarity based on the ratio of corresponding sides.
1. Understanding the Given Ratios: We have two triangles, PQR and XYZ, with the following ratios given:
PQXZ=PRXY=QRYZ
This indicates that the ratios of the lengths of corresponding sides of the two triangles are equal.
2. Identifying Corresponding Sides:
- In triangle PQR, the sides are:
- PQ
- PR
- QR
- In triangle XYZ, the corresponding sides are:
- XZ (corresponds to PQ)
- XY (corresponds to PR)
- YZ (corresponds to QR)
3. Applying the Criteria for Similarity:
According to the Side-Side-Side (SSS) similarity criterion, if the ratios of the lengths of corresponding sides of two triangles are equal, then the triangles are similar.
4. Conclusion: Since the ratios of the corresponding sides are equal, we can conclude that:
△PQR∼△XYZ
This means triangle PQR is similar to triangle XYZ.
Final Answer:
The triangles PQR and XYZ are similar.
---
PQXZ=PRXY=QRYZ
This indicates that the ratios of the lengths of corresponding sides of the two triangles are equal.
- In triangle PQR, the sides are:
- PQ
- PR
- QR
- In triangle XYZ, the corresponding sides are:
- XZ (corresponds to PQ)
- XY (corresponds to PR)
- YZ (corresponds to QR)
According to the Side-Side-Side (SSS) similarity criterion, if the ratios of the lengths of corresponding sides of two triangles are equal, then the triangles are similar.
△PQR∼△XYZ
This means triangle PQR is similar to triangle XYZ.
The triangles PQR and XYZ are similar.
Topper's Solved these Questions
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 11 (SELF - ASSESSMENT)EXERCISE - SECTION -B13videos
SAMPLE PAPER 11 (SELF - ASSESSMENT)
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 11EXERCISE - PART -B (SECTION-V)4videos
SAMPLE PAPER 11
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 13EXERCISE - PART - B (SECTION - V)4videos
SAMPLE PAPER 13
Similar Questions
Knowledge Check
- Question 1 - Select One
If in ΔPQRandΔXYZ,PQXY=QRXZ then the triangles will be similar, when
A∠P≅∠XB∠R≅∠YC∠Q≅∠YD∠Q≅∠X - Question 2 - Select One
In ΔDEFandΔPQR, if PQ =DE, EF=PR and FD =QR, then
AΔDEF≡ΔRPQBΔDEF≡ΔQPRCΔDEF≡ΔQRPDΔDEF≡ΔPQR - Question 3 - Select One
In a triangle PQR, PQ=20 cm and PR =6 cm, the side QR is :
Aequal to 14 cmBless than 14 cmCgreater than 14 cmDnone of the above
In △ XYZ, XY =4cm , YZ =6 cm , XZ =5 cm. If △ XYZ △PQR and PQ =8 cm then find QR and PR.
View SolutionIf S is a point on side PQ of a △PQR such that PS = QS = RS, then PR2+QR2 = ……………..
View Solution△PQR~△LTR. In △PQR,PQ=4.2 cm, QR=5.4 cm, PR=4.8 cm. Construct △PQR and △LTR, such that PQLT=34.
View SolutionIn right-angled triangle PQR, if ∠P=60∘,∠R=30∘andPR =12, then find the values of PQ and QR.
View SolutionIn the triangle PQR, AB is parallel to QR. The ratio of the areas similar triangles PAB and PQR is 1:2, then PQ:AQ= __________
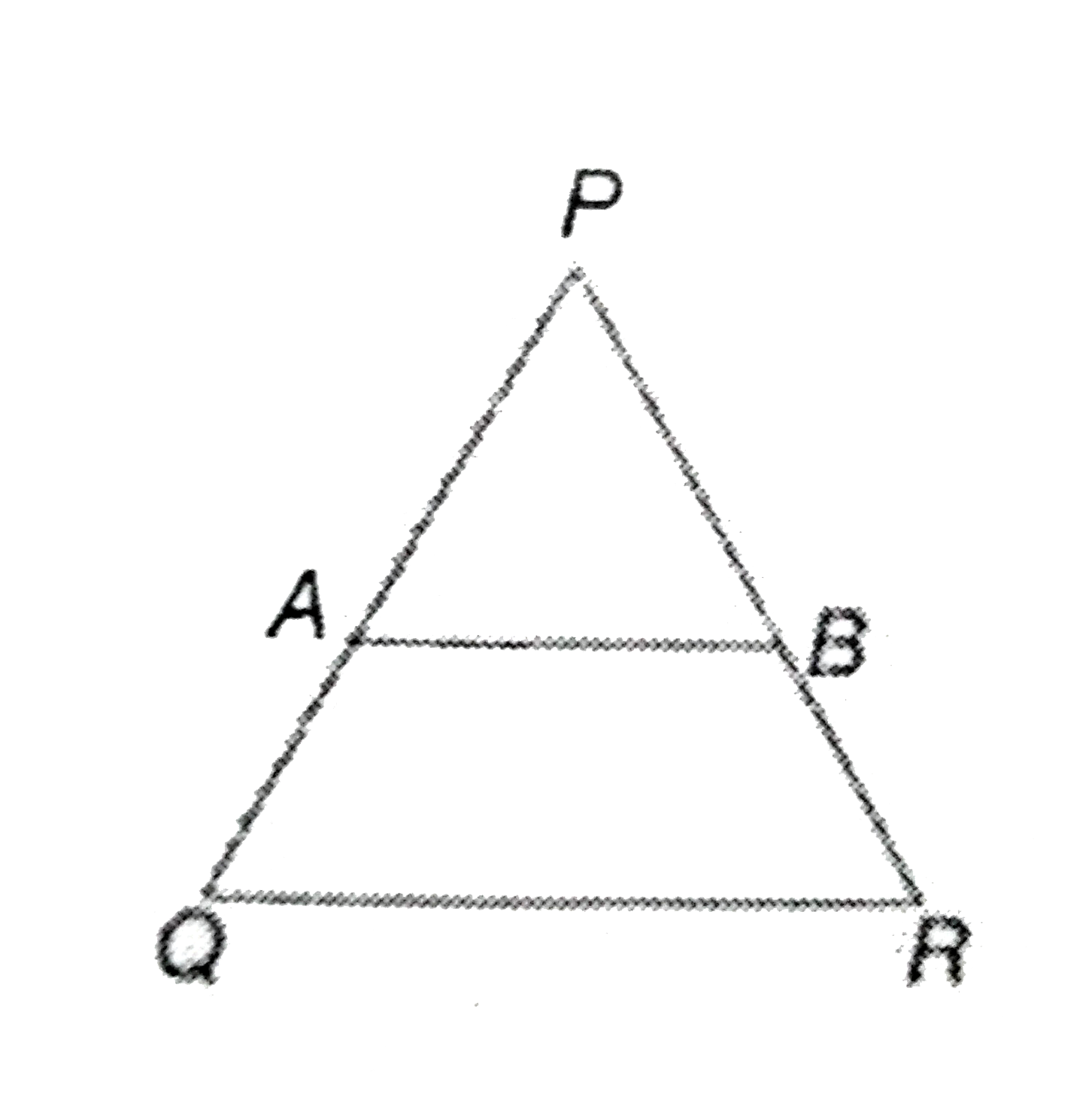 View Solution
View Solution

