If S is a point on side PQ of a △PQR such that PS = QS = RS, then PR2+QR2 = ……………..
The correct Answer is:PQ2
To solve the problem, we need to analyze the triangle PQR with point S on side PQ such that PS=QS=RS. We want to find the value of PR2+QR2.
1. Understanding the Given Information:
- We have a triangle PQR.
- Point S lies on side PQ such that PS=QS=RS.
- Let PS=QS=RS=x.
2. Identifying Angles:
- Since PS=QS, triangle PSQ is isosceles, which means ∠PSQ=∠PQS.
- Similarly, since QS=RS, triangle QSR is also isosceles, which means ∠QSR=∠QRS.
3. Using Angle Properties:
- The sum of angles in triangle PQR is 180∘.
- We can express the angles in terms of ∠R:
∠P+∠Q+∠R=180∘
- Since ∠PRS=∠RPS and ∠QRS=∠RQS, we can denote these angles as equal.
4. Applying the Pythagorean Theorem:
- Since PS=QS=RS and the angles formed are equal, we can conclude that △PRS and △QRS are right triangles.
- By the Pythagorean theorem:
PR2+QR2=PQ2
- Since PQ=PS+SQ=x+x=2x, we have:
PQ2=(2x)2=4x2
5. Final Expression:
- Thus, we can conclude:
PR2+QR2=PQ2=4x2
Conclusion:
The value of PR2+QR2 is 4x2.
---
- We have a triangle PQR.
- Point S lies on side PQ such that PS=QS=RS.
- Let PS=QS=RS=x.
- Since PS=QS, triangle PSQ is isosceles, which means ∠PSQ=∠PQS.
- Similarly, since QS=RS, triangle QSR is also isosceles, which means ∠QSR=∠QRS.
- The sum of angles in triangle PQR is 180∘.
- We can express the angles in terms of ∠R:
∠P+∠Q+∠R=180∘
- Since ∠PRS=∠RPS and ∠QRS=∠RQS, we can denote these angles as equal.
- Since PS=QS=RS and the angles formed are equal, we can conclude that △PRS and △QRS are right triangles.
- By the Pythagorean theorem:
PR2+QR2=PQ2
- Since PQ=PS+SQ=x+x=2x, we have:
PQ2=(2x)2=4x2
- Thus, we can conclude:
PR2+QR2=PQ2=4x2
The value of PR2+QR2 is 4x2.
Topper's Solved these Questions
- BOOK - EDUCART PUBLICATIONCHAPTER - TRIANGLESEXERCISE - VERY SHORT QUESTIONS10videos
TRIANGLES
- BOOK - EDUCART PUBLICATIONCHAPTER - TRIANGLESEXERCISE - SHORT ANSWER (SA-I) TYPE QUESTIONS30videos
TRIANGLES
- BOOK - EDUCART PUBLICATIONCHAPTER - TRIANGLESEXERCISE - LONG ANSWER TYPE QUESTIONS10videos
TRIANGLES
- BOOK - EDUCART PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - LONG ANSWER TYPE QUESTIONS30videos
SURFACE AREAS AND VOLUMES
Similar Questions
Knowledge Check
- Question 1 - Select One
If S is a point on side PQ of a ΔPQR such that PS=QS=RS, then
APR⋅QR=RS2BQS2+RS2=QR2CPR2+QR2=PQ2DPS2+RS2=PR2 - Question 2 - Select One
In a triangle PQR, PQ=20 cm and PR =6 cm, the side QR is :
Aequal to 14 cmBless than 14 cmCgreater than 14 cmDnone of the above - Question 3 - Select One
S and U are two points on the side PQ of ΔPQR , such that QU=PS . If ST||QR and UV∣∣PR , then
ATV||PQBTV⊥PQCTV and PQ intersect at 60∘DTV and PQ intersect at 45∘
S is any point on side QR of a ΔPQR. Show that PQ+QR+RP>2PS.
View SolutionIf S is the mid-point of side QR of a ΔPQR, then prove that PQ+PR=2PS.
View SolutionIn PQR , S is any point on the side QR . Show that PQ+QR+RP>2PS
View SolutionIn Figure, T is a point on side QR of PQR and S is a point such that RT=ST. Prove That : PQ+PR>QS
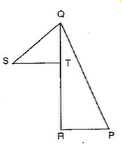 View Solution
View SolutionIn a triangle PQR, PQ = 20 cm and PR = 6 cm, the side QR is :
View Solution

