If mode of a series exceeds its mean by 12, then mode exceeds the median by (a) 4 (b) 8 (c) 6 (d) 10
4
8
6
10
The correct Answer is:B
We have,
Mode - Mean = 12
∴ Mode = 3 Median - 2 Mean
⇒ Mode - Mean = 3(Median - Mean)
⇒ 12 = 3(Median - Mean)
⇒ Median - Mean = 4
Again, Mode = 3 Median - 2 Mean
⇒ Mode - Median = 2( Median - Mean) = 2 ×4=8
Similar Questions
Number of vertices of a cuboid is:
View SolutionIf the difference of mode and median of a data is 24, then the difference of median and mean is (a) 12 (b) 24 (c) 8 (d) 36
View SolutionThe arithmetic mean and mode of a data are 24 and 12 respectively, then its median is (a) 25 (b) 18 (c) 20 (d) 22
View SolutionThe number of terms of an A.P. is even. The sum of the odd terms is 24, and of the even terms is 30, and the last term exceeds the first by 1012 then the number of terms in the series is a. 8 b. 4 c. 6 d. 10
View SolutionThe arithmetic mean of two positive numbers a and b exceeds their geometric mean by 32 and the geometric mean exceeds their harmonic mean by 65. If a+b=α and |a−b|=β, then the value of 10βα is equal to
View SolutionConsider two positive numbers a and b. If arithmetic mean of a and b exceeds their geometric mean by 3/2, and geometric mean of aand b exceeds their harmonic mean by 6/5 then the value of a2+b2 will be
View SolutionIf for a moderately skewed distribution, mode = 60 and mean = 66, then median =
View SolutionIf the mean of 9, 10, 15, x, 6, 8 and 12 is 11 and is 11. The median of the observations is
View SolutionFind mean, median and mode of the following data :
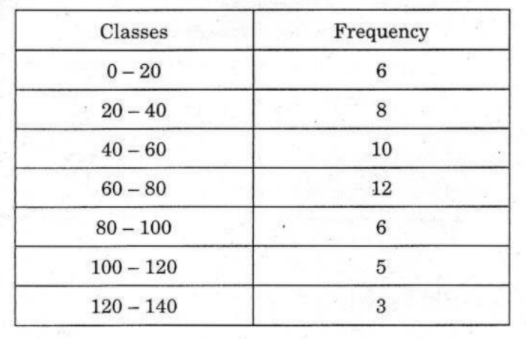
View SolutionFind the mean, median and mode of the following data :
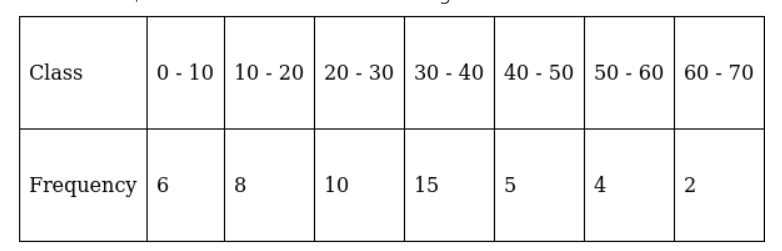
View Solution
