If for a moderately skewed distribution, mode = 60 and mean = 66, then median =
60
64
68
none of these
The correct Answer is:B
To find the median of a moderately skewed distribution given the mode and mean, we can use the relationship between mode, median, and mean. The formula is:
Mode=3×Median−2×Mean
1. Identify the given values:
- Mode = 60
- Mean = 66
2. Write the formula:
Mode=3×Median−2×Mean
3. Substitute the known values into the formula:
60=3×Median−2×66
4. Calculate 2×Mean:
2×66=132
5. Rearrange the equation:
60=3×Median−132
6. Add 132 to both sides:
60+132=3×Median
192=3×Median
7. Divide both sides by 3 to solve for Median:
Median=1923=64
Final Answer:
The median is 64.
- Mode = 60
- Mean = 66
Mode=3×Median−2×Mean
60=3×Median−2×66
2×66=132
60=3×Median−132
60+132=3×Median
192=3×Median
Median=1923=64
The median is 64.
Topper's Solved these Questions
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Exercise40videos
MEASURES OF CENTRAL TENDENCY
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Chapter Test29videos
MAXIMA AND MINIMA
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MISCELLANEOUS EQUATIONS AND INEQUATIONS EXERCISE - Chapter Test29videos
MISCELLANEOUS EQUATIONS AND INEQUATIONS
Similar Questions
The relationship between mean, median and mode for a moderately skewed distribution is (a) Mode = 2 Median – 3 Mean (b) Mode = Median – 2 Mean (c) Mode = 2 Median – Mean (d) Mode = 3 Median – 2 mean
View SolutionIf in a moderately asymmetrical distribution the mode and the mean of the data are 6λ and9λ, respectively, then the median is
View Solution
Knowledge Check
- Question 1 - Select One
If the mean and the mode of a moderately skewed frequency distribution are 90 and 96 respectively, then the median is
A93B92C98D88
Iin a moderately skewed distribution the values of mean and median are 5 and 6 respectively. The value of mode in such a situation is approximately equal to
View SolutionIn an asymmetrical distribution mean is 58 and median is 61. Find mode.
View SolutionIn a moderated asymmetrical distribution S.D. is 20 then the mean deviation is
View SolutionMode of the following distribution is
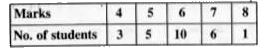 View Solution
View SolutionIf the sum of the mode and mean of a certain frequency distribution is 129 and the median of the observations is 63, mode and mean are respectively
View SolutionFor a normal distribution if the mean is M, mode is M0 and median is Md, then
View SolutionIn a binomial distribution, the mean is 4 and variance is 3. Then its mode is
View Solution

