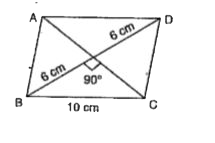
If one side of a rhombus if 17 cm and length of one of its diagonals is 16 cm. What is the length of other diagonal (incm)?
To find the length of the other diagonal of the rhombus, we can follow these steps:
1. Understand the Properties of a Rhombus:
A rhombus has all sides equal, and its diagonals bisect each other at right angles.
2. Identify Given Information:
- Length of one side of the rhombus (AB = 17 cm).
- Length of one diagonal (AC = 16 cm).
3. Label the Points:
Let the vertices of the rhombus be labeled as A, B, C, and D. The diagonals intersect at point O.
4. Calculate Half of the Given Diagonal:
Since the diagonals bisect each other, we can find AO and OC:
AO=OC=AC2=16 cm2=8 cm
5. Use Pythagoras Theorem:
In triangle AOB, we can apply the Pythagorean theorem:
AB2=AO2+BO2
Here, AB = 17 cm, AO = 8 cm, and BO is the half of the other diagonal (let's call it x):
172=82+x2
6. Calculate the Squares:
289=64+x2
7. Rearrange the Equation:
x2=289−64=225
8. Find x:
x=√225=15 cm
9. Calculate the Length of the Other Diagonal:
Since BO = OD = x, the full length of diagonal BD is:
BD=BO+OD=x+x=2x=2×15 cm=30 cm
Final Answer:
The length of the other diagonal (BD) is 30 cm.
---
A rhombus has all sides equal, and its diagonals bisect each other at right angles.
- Length of one side of the rhombus (AB = 17 cm).
- Length of one diagonal (AC = 16 cm).
Let the vertices of the rhombus be labeled as A, B, C, and D. The diagonals intersect at point O.
Since the diagonals bisect each other, we can find AO and OC:
AO=OC=AC2=16 cm2=8 cm
In triangle AOB, we can apply the Pythagorean theorem:
AB2=AO2+BO2
Here, AB = 17 cm, AO = 8 cm, and BO is the half of the other diagonal (let's call it x):
172=82+x2
289=64+x2
x2=289−64=225
x=√225=15 cm
Since BO = OD = x, the full length of diagonal BD is:
BD=BO+OD=x+x=2x=2×15 cm=30 cm
The length of the other diagonal (BD) is 30 cm.
Topper's Solved these Questions
- PRACTICAL GEOMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - PRACTICAL GEOMETRYEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL-2 COMPREHENSION TYPE)
4 Videos
- PLAYING WITH NUMBERSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - PLAYING WITH NUMBERSEXERCISE - Olympiad/HOTS Corner
6 Videos
- RATIONAL NUMBERS BOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIONAL NUMBERS EXERCISE - Olympiad/HOTS Corner
20 Videos
Similar Questions
Knowledge Check
The perimeter of a rhombus is 20 cm. The length of one of its diagonal is 6 cm. What is the length of the other diagonal ?
A6 cmB9 cmC8 cmD10 cmIf length of each side of a rhombus is 5 cm and length of one of its diagonal is 8 cm, find the length of other diagonal.
AA)5cmBB)6cmCC)7cmDD)8cmThe area of a rhombus is 36 cm and the length of one of its diagonals is 6 cm. The length of the second diagonal is
A6cmB6√2cmC12cmDnone of these
The perimeter of a rhombus is 40 cm. If the length of one of its diagonal is 10 cm, what is the length of the other diagonal?
View SolutionThe area of a rhombus is 132 cm2. If length of one of its diagonals is 11 cm, then what is the length of the other diagonal?
View SolutionIf each side of a rhombus is 10 cm and one of its diagonals is 16 cm , then the length of the other diagonal is k cm . Find k .
View SolutionOne side of a rhombus is 13 cm and one of its diagonals is 24 cm. What is the area of the rhombus?
View SolutionThe area of a rhombus is 150cm2. The length of one of its diagonals is 10 cm. The length of the other diagonal is :
View Solution
