If Sr=√r+√r+√r+√......∞,r>0 then which the following is\are correct.
S2,S6,S13,S20 are in A.P.
S4,S9,S16 are irrational
(2S3−1)2,(2S4−1)2,(2S2−1)2 are in A.P.
S2,S12,S36 are in G.P.
The correct Answer is:A, B, C, D
To solve the problem Sr=√r+√r+√r+… for r>0, we can follow these steps:
Step 1: Set up the equation
We start by recognizing that the expression inside the square root is the same as Sr. Therefore, we can write:
Sr=√r+Sr
Step 2: Square both sides
To eliminate the square root, we square both sides of the equation:
S2r=r+Sr
Step 3: Rearrange the equation
Rearranging gives us a standard quadratic equation:
S2r−Sr−r=0
Step 4: Apply the quadratic formula
Using the quadratic formula Sr=−b±√b2−4ac2a, where a=1,b=−1,c=−r:
Sr=1±√1+4r2
Step 5: Choose the correct root
Since Sr must be positive (as r>0), we take the positive root:
Sr=1+√1+4r2
Step 6: Analyze the options
Now we need to check the options given in the question:
1. First option: Check if S2,S6,S12,S20 are in arithmetic progression (AP).
- Calculate S2,S6,S12,S20:
- S2=1+√1+82=1+32=2
- S6=1+√1+242=1+52=3
- S12=1+√1+482=1+72=4
- S20=1+√1+802=1+92=5
- The sequence 2,3,4,5 has a common difference of 1, so it is in AP.
2. Second option: Check if S4,S9,S16 are irrational.
- Calculate S4,S9,S16:
- S4=1+√1+162=1+52=3 (irrational)
- S9=1+√1+362=1+72=4 (irrational)
- S16=1+√1+642=1+92=5 (irrational)
- All values are irrational.
3. Third option: Check if 2S2−1,2S4−1,2S6−1 are in AP.
- Calculate:
- 2S2−1=2(2)−1=3
- 2S4−1=2(3)−1=5
- 2S6−1=2(4)−1=7
- The sequence 3,5,7 has a common difference of 2, so it is in AP.
4. Fourth option: Check if S2,S12,S56 are in geometric progression (GP).
- Calculate:
- S12=4 (as calculated before)
- S56=1+√1+2242=1+152=8
- The sequence 2,4,8 has a common ratio of 2, so it is in GP.
Conclusion
All options are correct.
We start by recognizing that the expression inside the square root is the same as Sr. Therefore, we can write:
Sr=√r+Sr
To eliminate the square root, we square both sides of the equation:
S2r=r+Sr
Rearranging gives us a standard quadratic equation:
S2r−Sr−r=0
Using the quadratic formula Sr=−b±√b2−4ac2a, where a=1,b=−1,c=−r:
Sr=1±√1+4r2
Since Sr must be positive (as r>0), we take the positive root:
Sr=1+√1+4r2
Now we need to check the options given in the question:
- Calculate S2,S6,S12,S20:
- S2=1+√1+82=1+32=2
- S6=1+√1+242=1+52=3
- S12=1+√1+482=1+72=4
- S20=1+√1+802=1+92=5
- The sequence 2,3,4,5 has a common difference of 1, so it is in AP.
- Calculate S4,S9,S16:
- S4=1+√1+162=1+52=3 (irrational)
- S9=1+√1+362=1+72=4 (irrational)
- S16=1+√1+642=1+92=5 (irrational)
- All values are irrational.
- Calculate:
- 2S2−1=2(2)−1=3
- 2S4−1=2(3)−1=5
- 2S6−1=2(4)−1=7
- The sequence 3,5,7 has a common difference of 2, so it is in AP.
- Calculate:
- S12=4 (as calculated before)
- S56=1+√1+2242=1+152=8
- The sequence 2,4,8 has a common ratio of 2, so it is in GP.
All options are correct.
Topper's Solved these Questions
- SEQUENCE AND SERIES BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE (COMPREHENSION TYPE PROBLEMS)
16 Videos
- SEQUENCE AND SERIES BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE (MATCHING TYPE PROBLEMS)
4 Videos
- SEQUENCE AND SERIES BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
21 Videos
- QUADRATIC EQUATIONS BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - QUADRATIC EQUATIONS EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
45 Videos
- SOLUTION OF TRIANGLESBOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-5 : Subjective Type Problems
11 Videos
Similar Questions
Knowledge Check
If s=t+√r3q, what is the value of r in terms of q, s and t ?
A3√qs2−qtB3√s2−2st−t2qC3√qs2−2qst+qt2D√qs2+2qst−t23
Integrate the following w.r.t x
√1−sinx
View SolutionDifferentiate the following w.r.t x
√sinx
View Solutionn∑r=1sin−1(√r−√r−1√r(r+1)) is equal to
View SolutionConsider
∫x3+3x2+2x+1√x2+x+1dx
=(ax2+bx+c)√x2+x+1+λ∫dx√x2+x+1
Now, match the following lists and then choose the correct code.
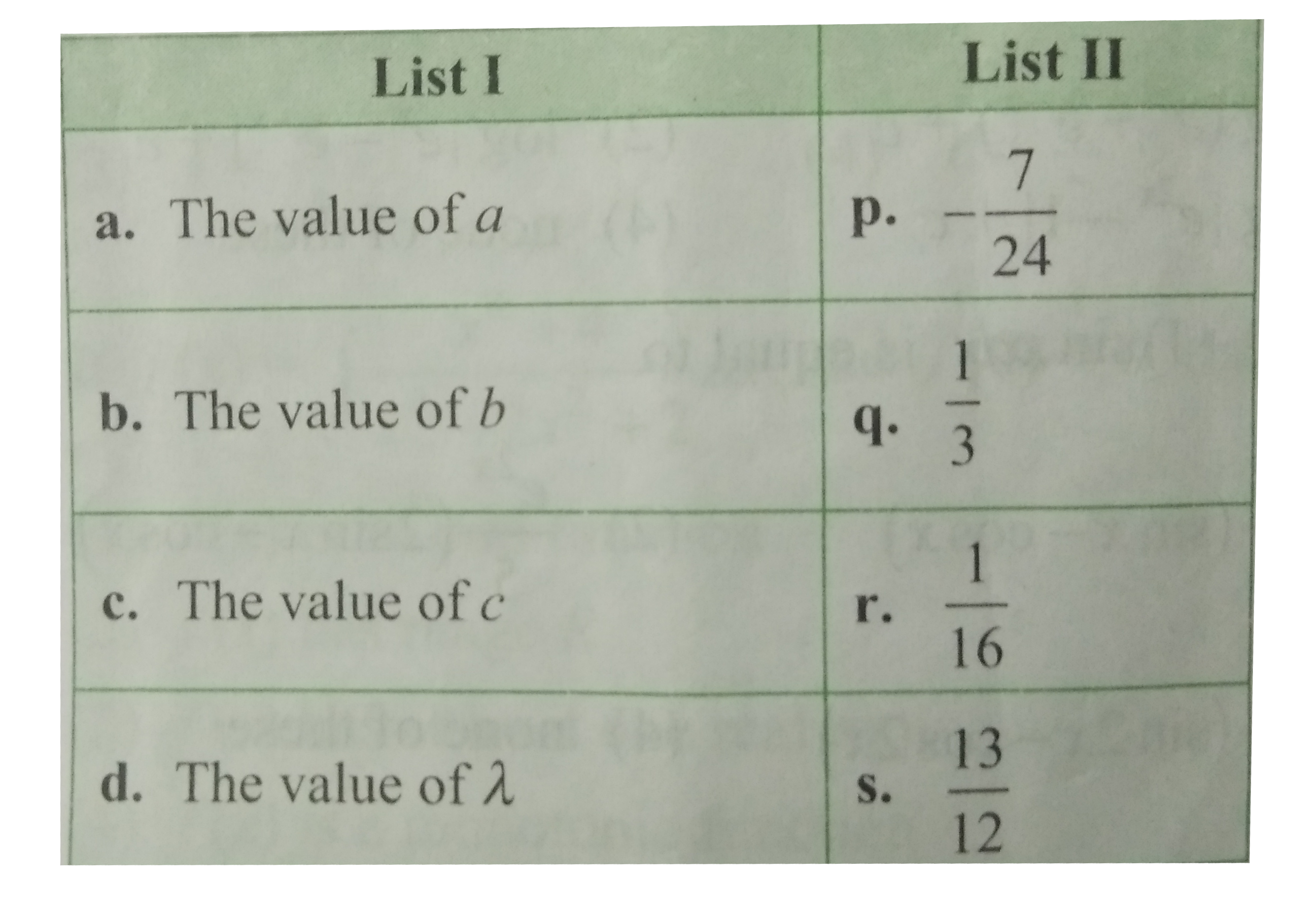
Codes:
abcd(1)qpsr(2)spqr(3)rqps(4)qspr
View SolutionDifferentiate √sin−1√x w.r.t. x, (0<x<1)
View SolutionIf r is positive real number such that 4√r−14√r=4, then find the value of 6√r+16√r.
View Solution(r2+r3)√rr1r2r3=
View Solution
