If the centroid of a triangle formed by the points a. b), (b, c), and (c a) is at the origin, then a3+b3+c3 = Solution in Kannada
abc
0
a + b + c
3 abc
The correct Answer is:A, B, C
Step by step video, text & image solution for If the centroid of a triangle formed by the points a. b), (b, c), and (c a) is at the origin, then a^(3) + b^(3) + c^(3) = by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CO-ORDINATE GEOMETRYBOOK - ZEN PUBLICATIONCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - ZEN ADDITIONAL QUESTIONS - VERY SHORT ANSWER [VSA] TYPE QUESTIONS
14 Videos
- CO-ORDINATE GEOMETRYBOOK - ZEN PUBLICATIONCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - ZEN ADDITIONAL QUESTIONS - SHORT ANSWER [SA] TYPE QUESTIONS
57 Videos
- CO-ORDINATE GEOMETRYBOOK - ZEN PUBLICATIONCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - TEXTUAL EXERCISE EXERCISE 7.4
8 Videos
- CIRCLESBOOK - ZEN PUBLICATIONCHAPTER - CIRCLESEXERCISE - ZEN ADDITIONAL QUESTIONS ( HOT [HIGHER ORDER THINKING SKILLS] - QUESTIONS) (IIT AND IMO)
9 Videos
- CONSTRUCTIONSBOOK - ZEN PUBLICATIONCHAPTER - CONSTRUCTIONSEXERCISE - ZEN ADDITIONAL QUESTIONS ( HOTS [HIGHER ORDER THINKING SKILLS] - QUESTIONS) IIT /OLYMPIAD/ IMO
5 Videos
Similar Questions
Find the area of the triangle Δ ABC with A(a,b+c), B(b,c+a), and C(c, a+ b).
View SolutionFind the value (s) of x if distance between A (0, 0) and B (x,-4) is 5 units.
View SolutionDetermine the ratio in which the line 2x + y - 4 = 0 divides the line segment joining the points A(2, -2) and B(3, 7).
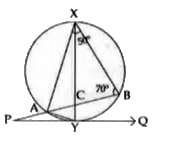
View SolutionIn the adjoining figure, XY is a diameter of the circle and PQ is a tangent to the circle at Y. Given that ⌊AXB=50∘ and ⌊ABX=70∘, calculate ⌊BAY and ⌊APY.
View SolutionGiven below are the steps of construction of a pair of tangents to a circle of radius 4 cm from a point on the concentric circle of radius 6 cm. Find which of the following steps is wrong.
(P) Step 1: Take a point O on the plane paper and draw a circle of radius OA = 4 cm. Also draw a concentric circle of radius OB = 6 cm.
(Q) Step 2: Find the midpoint C of OB and draw a circle of radius BA= AO. Suppose this circle intersects the circle of radius 4 cm at P and Q.
(R) Step 3: Join BP and BQ to get the desired tangents.
View SolutionIf the centroid of the triangle formed by the points (a,b),(b,c) and (c,a) is at the origin, then find the value of a^(3)+b^(3)+c^(3).
View SolutionIf the centroid of the triangle formed by points P(a, b), Q(b, c) and R(c, a) is at the origin, what is the value of a+b+c ?
View SolutionIf the centroid of the triangle formed by the points (a, b), (b, c) and (c, a) is at the origin, then a3+b3+c3= abc (b) 0 (c) a+b+c (d) 3 abc
View SolutionIf the centroid of ΔABC having vertices A(a, b), B(b, c) and C(c, a) is the origin, then find the value of (a+b+c).
View SolutionThe centroid of the triangle with vertices A(2, -3, 3), B(5, -3, -4) and C (2, -3, -2) is the point
View Solutionयदि बिन्दुओं (a,b),(b,c) तथा (c,a) से बने त्रिभुज का केन्द्रक मूल बिन्दु पर स्थित हो, तो a3+b3+c3=
View Solutionlf origin is centroid of triangle with vertices (a, 1 , 3), (- 2, b, -5) and (4, 7, c), then (a, b, c) ≡
View SolutionIf the centroid of the triangle formed with (a , b) , (b , c) and (c , a) is O(0 , 0) then a3+b3+c3= .....
View Solution(1, -2) and (-2, 3) are the points A and B of the ΔABC. If the centroid of the triangle be at the origin, then find the coordinates of C.
View SolutionA : The centroid of the triangle formed by the points 2a + 3b, 5a + 4b, 2a - b is 3a + 2b.
R : The centroid of the triangle formed by the points a, b, c is a+b+c3
View Solution
