If the circumference of a circle is equal to the perimeter of a square then the ratio of their areas is: (a)22:7 (b) 14:11 (c) 7:22 (d) 7:11
First, Equate perimeter of a square and circle.
Assume r be the radius of a circle
and be the side of a square.
Given: Perimeter of a square = Circumference of a circle
⇒4a=2πr
⇒ar=π2………….(1)
Now, Calculate the ratio of area of square and circle.
Area of circle : Area of square
⇒ Area of circle Area of square =πr2a2
⇒ Area of circle Area of square =π(ra)2
⇒ Area of circle Area of square =π(2π)2 (Fromequation(1)
⇒ Area of circle Area of square =π4π2
⇒ Area of circle Area of square =4π
⇒ Area of circle Area of square =4×722 (π=227)
⇒ Area of circle Area of square =1411
Hence, option (B)14:11 is required answer.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The mean of 100 numbers observations is 50 and their standards deviation is 5. The sum of all squares of all the observations is
A50,000B250,000C252500D255000
If 233 is divided by 17, then the remainder is
(1) 15 (2) 2
(3) 4 (4) 9
View SolutionIf the difference between mean and m0de is 63, the difference between mean and median is 189 (b) 21 (c) 31.5 (d) 48.5
View SolutionThe measure of the angle between the internal and external bisectors of an angle is:
(A) 60° (B) 70°
(C) 80° (D) 90°
View SolutionIf ∫(1+xtanx)−2dx=1x+f(x)+c; then value of f(x) is
(1) xtanx (2) cotx (3) tanx (4) tan2x
View SolutionIf α, β are the roots of 6x2−2x+1=0 and sx=αn+βn, then Ltn→∞n∑r=1sr is
(a) 517 (b) 0 (c) 337 (d) none
View SolutionFind the volume in cubic decimetre of each of the cubes whose side is (i)1.5m (ii) 75cm (iii) 2dm 5cm
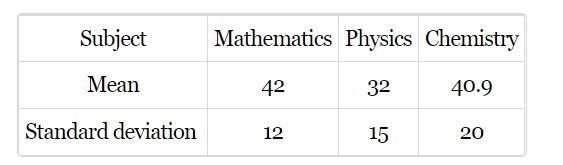
View SolutionThe mean and standard deviation of marks obtained by 50 students of a class in three subject, Mathematics, Physics and Chemistry are given below :
Which of the three subjects shows the highest variability in marks and which shows the lowest?
View Solution