If the inequality ${cot}^{2} x + (k + 1) cot x - (k - 3) < 0$ is true for at least one $x \in (0, π / 2)$ , then $k \in$ .Solution in Tamil

A

$(- \infty, 3 - 2 \sqrt{5})$

B

$(3, \infty)$

C

$(- 1, \infty)$

D

$(- \infty, 3)$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:1,2

Let cot x = t.
Since $x \in (0, π / 2), t \in (0, \infty)$
Therefore, $f (t) = t^{2} + (k + 1) t - (k - 3) < 0$ for at least one $t \in (0, \infty)$ .
We have following possibilities for the graph of f(t).
Case I :

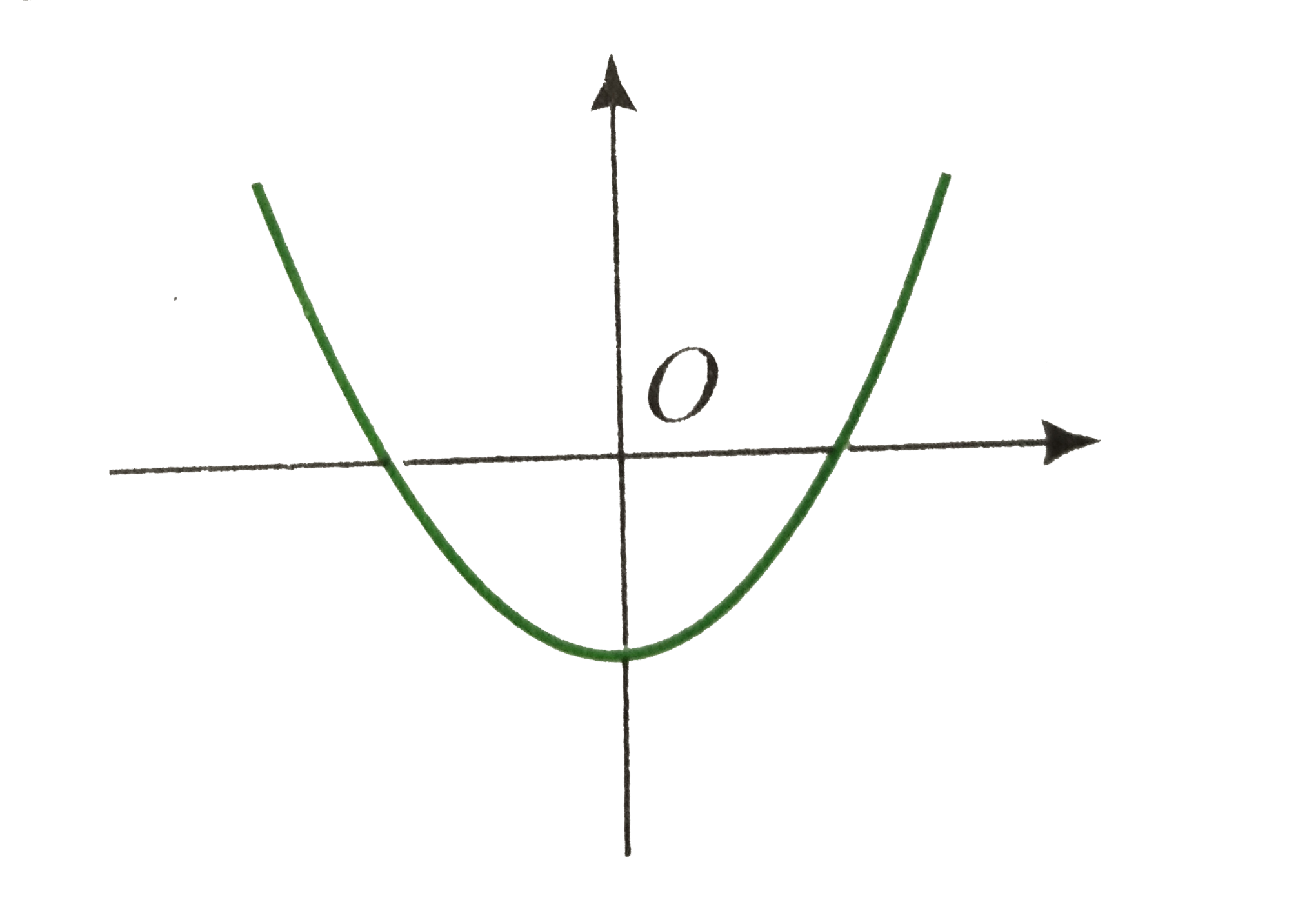
$f (0) < 0 \Rightarrow k > 3$
Case II :

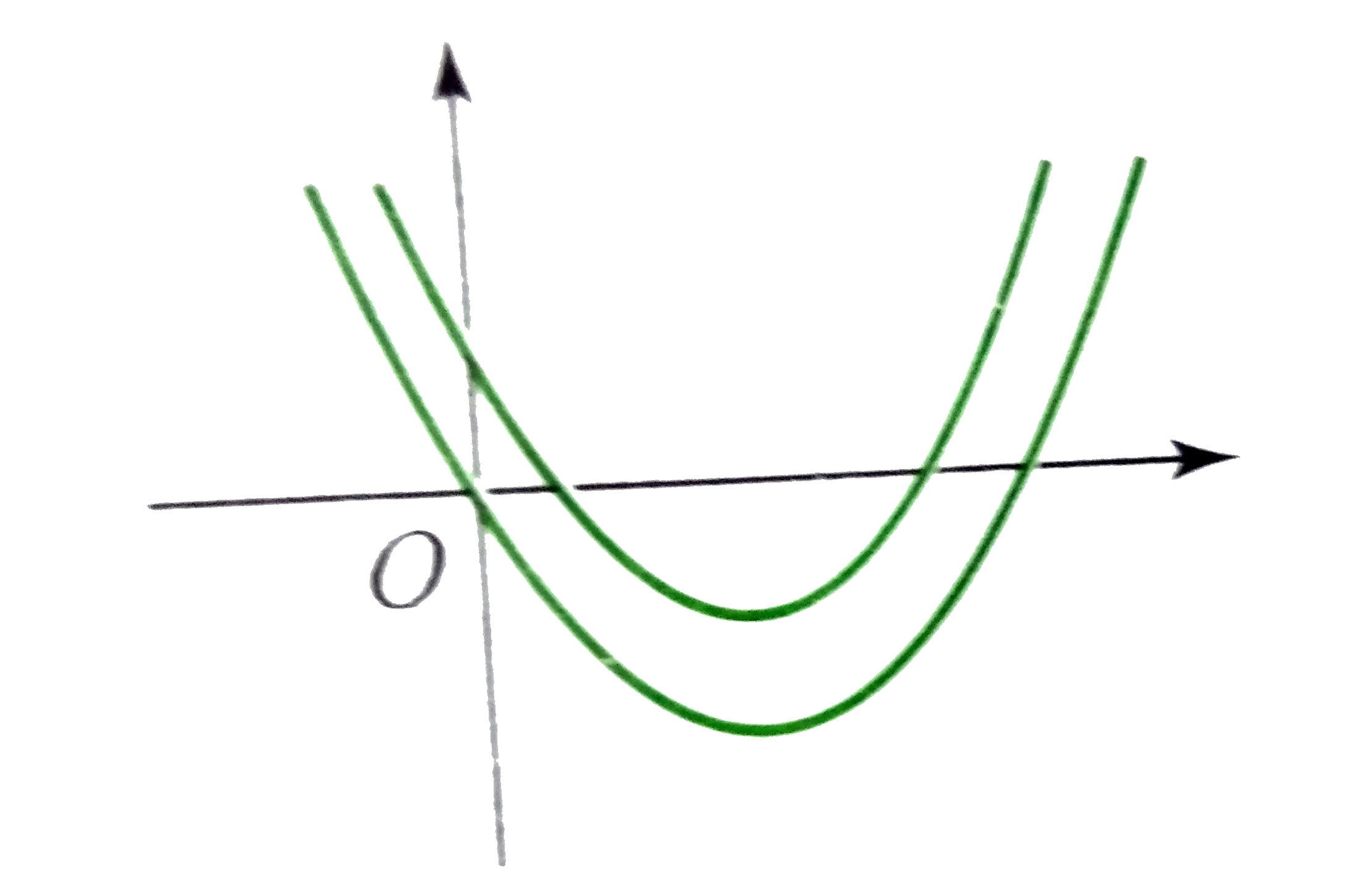
$D > 0$
$\Rightarrow {(k + 1)}^{2} + 4 (k - 3) > 0$
$\Rightarrow k^{2} + 6 k - 11 > 0$
$\Rightarrow {(k + 3)}^{2} > 20$
$\Rightarrow k + 3 < - 2 \sqrt{5} or k + 3 > 2 \sqrt{5}$
$\Rightarrow k < - 3 - 2 - \sqrt{2} or k > - 3 + 2 \sqrt{5}$
$\frac{- b}{2 a} > 0$
$\Rightarrow - (k + 1) > 0$
$\Rightarrow k < - 1 ... . . (2)$
$\Rightarrow k (0) \geq 0$
$\Rightarrow - k + 3 \geq 0$
$\Rightarrow k \leq 3 ... . (3)$
From (1),(2) and (3) we get
$k \in (- \infty, - 3 - 2 \sqrt{5})$
So, from the both cases, $k \in (- \infty, - 3 - 2 \sqrt{5}) \cup (3, \infty)$

Updated on:21/07/2023

|

Class 12 MATHS QUADRATIC EQUATIONS

Topper's Solved these Questions

Similar Questions

CENGAGE-THEORY OF EQUATIONS-Exercise (Multiple)

Home
Ask Doubt
Profile