If the mean of the following distribution is 54, find the value of p: Class: 0-20 20-40 40-60 60-80 80-100 Frequency: 7 p 10 9 13
To solve the problem step by step, we will follow the outlined approach in the video transcript.
Step 1: Set up the data in tabular form
We have the following classes and frequencies:
| Class | Frequency (F) |
|-----------|---------------|
| 0 - 20 | 7 |
| 20 - 40 | p |
| 40 - 60 | 10 |
| 60 - 80 | 9 |
| 80 - 100 | 13 |
Step 2: Calculate the mid-values (X_i)
The mid-values for each class are calculated as follows:
- For 0 - 20: X1=0+202=10
- For 20 - 40: X2=20+402=30
- For 40 - 60: X3=40+602=50
- For 60 - 80: X4=60+802=70
- For 80 - 100: X5=80+1002=90
Step 3: Assume a mean (A)
We will assume the mean A=50.
Step 4: Calculate the class interval (H)
The class interval H is calculated as:
H=20−0=20
Step 5: Calculate U_i values
Using the formula Ui=Xi−AH:
- U1=10−5020=−2
- U2=30−5020=−1
- U3=50−5020=0
- U4=70−5020=1
- U5=90−5020=2
Step 6: Calculate Ui×Fi
Now we calculate Ui×Fi:
- For class 0 - 20: 7×(−2)=−14
- For class 20 - 40: p×(−1)=−p
- For class 40 - 60: 10×0=0
- For class 60 - 80: 9×1=9
- For class 80 - 100: 13×2=26
Summing these gives:
∑Ui×Fi=−14−p+0+9+26=21−p
Step 7: Calculate total frequency
The total frequency ∑Fi is:
∑Fi=7+p+10+9+13=39+p
Step 8: Use the mean formula
The mean ¯X is given by:
¯X=A+∑Ui×Fi∑Fi×H
Substituting the values we have:
54=50+21−p39+p×20
Step 9: Simplify the equation
Subtract 50 from both sides:
4=21−p39+p×20
Dividing both sides by 20:
21−p39+p=15
Step 10: Cross-multiply
Cross-multiplying gives:
5(21−p)=1(39+p)
Expanding both sides:
105−5p=39+p
Step 11: Solve for p
Rearranging gives:
105−39=p+5p
66=6p
Dividing by 6:
p=11
Conclusion
Thus, the value of p is 11.
---
|-----------|---------------|
| 0 - 20 | 7 |
| 20 - 40 | p |
| 40 - 60 | 10 |
| 60 - 80 | 9 |
| 80 - 100 | 13 |
- For 20 - 40: X2=20+402=30
- For 40 - 60: X3=40+602=50
- For 60 - 80: X4=60+802=70
- For 80 - 100: X5=80+1002=90
- U2=30−5020=−1
- U3=50−5020=0
- U4=70−5020=1
- U5=90−5020=2
- For class 20 - 40: p×(−1)=−p
- For class 40 - 60: 10×0=0
- For class 60 - 80: 9×1=9
- For class 80 - 100: 13×2=26
Topper's Solved these Questions
Similar Questions
If the mean of the following distributions 54, find the value of p: Class: 0-20 20-40 40-60 60-80 80-100 Frequency: 7 p 10 9 13
View SolutionIf the mean of the following distribution is 27, find the value of p . Class: 0-10 10-20 20-30 30-40 40-50 Frequency: 8 p 12 13 10
View SolutionThe mean of the following frequency distribution is 50. Find the value of p. Classes 0-20 20-40 40-60 60-80 80-100 Frequency 17 28 32 p 19
View SolutionThe mean of the following distribution is 54. Find the value of p using direct method :
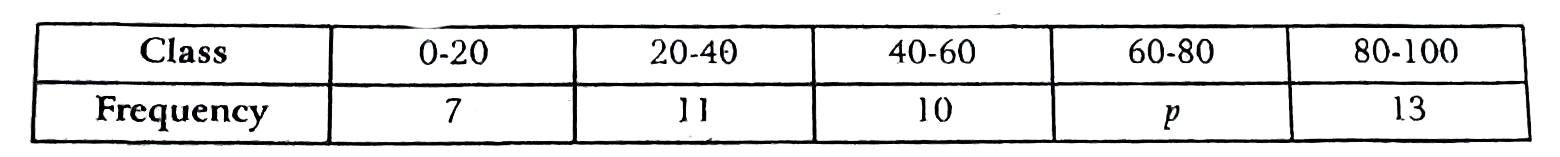 View Solution
View SolutionFind the mean of the following frequency distribution: Classes: 0-20 20-40 40-60 60-80 80-100 Frequency: 15 18 21 29 17
View SolutionFind the mean of the following frequency distribution: Classes: 0-20 20-40 40-60 60-80 80-100 Frequency: 15 18 21 29 17
View SolutionFind the mean of the following frequency distribution, using step-deviation method: Class 0-10 10-20 20-30 30-40 40-50 Frequency 7 12 13 10 8
View SolutionFind the mean of the following frequency distribution using step-deviation method. Class 0-10 10-20 20-30 30-40 40-50 Frequency 7 10 15 8 10
View SolutionFind the mode of the following distribution: (i) Class-interval: 0-10 10-20 20-30 30-40 40-50 50-60 60-70 70-80 Frequency: 5 8 7 12 28 20 10 10
View SolutionFind the mean of the following frequency distributions: Class interval: 0-10 10-20 20-30 30-40 40-50 Frequency: 9 12 15 10 14
View Solution

