If the nth term of an arithmetic progression an=24−3n, then it's 2nd term is Solution in Kannada
18
15
0
2
Step by step video & image solution for If the n^(th) term of an arithmetic progression a_(n)=24-3n, then it's 2^(nd) term is by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PRACTICE QUESTION PAPER 13BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - PRACTICE QUESTION PAPER 13EXERCISE - ANSWER THE FOLLOWING QUESTIONS
34 Videos
- PRACTICE QUESTION PAPER 12BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - PRACTICE QUESTION PAPER 12EXERCISE - ANSWER THE FOLLOWING QUESTION
32 Videos
- PROBABILITYBOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - PROBABILITYEXERCISE - EXERCISE 14. 2 (OPTIONAL)
5 Videos
Similar Questions
The given graph represents a pair of linear equations in two variables : write how many solutions these pair of equations have
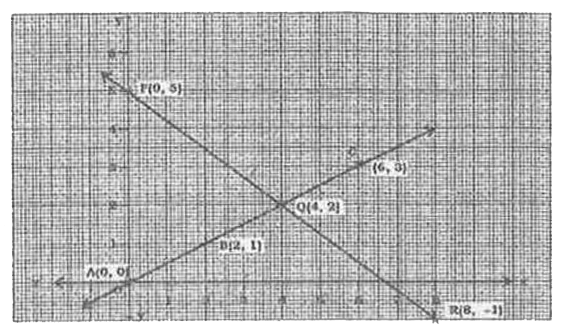
View SolutionIf the product of zeroes of polynomial f(y) =ay3−6y2+11y−6 is 4 then find the value of 'a'.
View SolutionTwo customers shyam and Ekta are visiting a particular shop in the same week (Tuesday to Saturday) . Each is equally likely to visit the shop on any day as on another day. What is the probability that both will visit the shop on (i) the same day ? (ii) consecutive day ? (iii) different days ?
View SolutionWrite the common differnece of an Arithmetic Progression, whose nth term is given by tn=3n+7.
View SolutionIf the n-th term of an arithmetic progression an = 24 - 3n, then its 2nd term is
View SolutionIf the nth term of an arithmetic progression an=24−3n, then it's 2nd term is
View SolutionThe sum of n terms of two arithmetic progressions are in the ratio (3n + 8) : (7n + 15). Find the ratio of their 12th terms.
View SolutionThe sum of n terms of two arithmetic progressions are in the ratio (3n + 8) : (7n + 15). Find the ratio of their 12th terms.
View SolutionThe sum of n terms of two arithmetic progressions are in the ratio (3n + 8) : (7n + 15). Find the ratio of their 12th terms.
View SolutionThe sum of n terms of two arithmetic progressions are in the ratio (3n + 8) : (7n + 15). Find the ratio of their 12th terms.
View SolutionIf the n−th term of an arithmetic progression an=24−3n then its 2nd term is
View SolutionThe sum of n terms of two arithmetic progressions are in the ratio (3n+8):(7n+15). Find the ratio of their 12th terms.
View SolutionThe n th term of a progression is 3n−5.Find its 15th term.
View SolutionIn an arithmetic progression,if Sn=n(5+3n) and tn=32 ,then the value of n is
[Note: S(n) and t(n) denote the sum of first n terms and n th term of arithmetic progression respectively.]
View SolutionThe sum of n terms of two arithmetic progressions are in the ratio (3n+8):(7n+15). Find the ratio of their 12th terms.
View SolutionIf the sum of the first n terms of an A.P. is 3n+n2, what is the first term? What is the 2nd term, 3rd term, 10th term and the nth terms.
View Solution
