If xi's are the mid-points of the class intervals of grouped data fi's are the corresponding frequencies and ¯x is the mean, then Σ(xifi−¯x) equal to:
0
−1
1
2
The correct Answer is:A
Topper's Solved these Questions
- STATISTICSBOOK - OSWAL PUBLICATIONCHAPTER - STATISTICSEXERCISE - Very Short Answer Type Questions
4 Videos
- STATISTICSBOOK - OSWAL PUBLICATIONCHAPTER - STATISTICSEXERCISE - Short Answer Type Questions
2 Videos
- STATISTICSBOOK - OSWAL PUBLICATIONCHAPTER - STATISTICSEXERCISE - BOARD CORNER (Long answer type questions)
7 Videos
- SOME APPLICATIONS OF TRIGONOMETRY (HEIGHTS AND DISTANCES) BOOK - OSWAL PUBLICATIONCHAPTER - SOME APPLICATIONS OF TRIGONOMETRY (HEIGHTS AND DISTANCES) EXERCISE - CASE-BASED MCQs
15 Videos
- SURFACE AREAS AND VOLUMES BOOK - OSWAL PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMES EXERCISE - BOARD CORNER (LONG ANSWER TYPE QUESTIONS)
9 Videos
Similar Questions
If xi's are the mid-points of the class intervals of grouped data, fi's are the corresponding frequencies and ¯x is the mean, then ∑(fixi−¯x) equal to
View SolutionIf xi' s are the midpoints of the class intervals of grouped data, fi's are the corresponding frequencies and ¯x is the mean, then what is Σ(fixi−¯x) equal to ?
View Solution
Knowledge Check
If xi's are the mid-points of the class intervals of grouped data, fi's are the corresponding frequencies and ¯x is the mean, then ∑(fixi−¯x) equal to
A0B−1C1D2If xi's are the mid-points of the class intervals of grouped data fi's are the corresponding frequencies and x is the mea, then ∑(fixi−x) is equal to
A0B−1C1D2If xi' is the mid-points of the class intervals of grouped data, fi' s are corresponding frequencies and ¯x is the mean , then find the value of ∑(fixi−¯x).
A0B1C2D3
The measurments ( in mm) of the diameters of the head of the screws are given below :
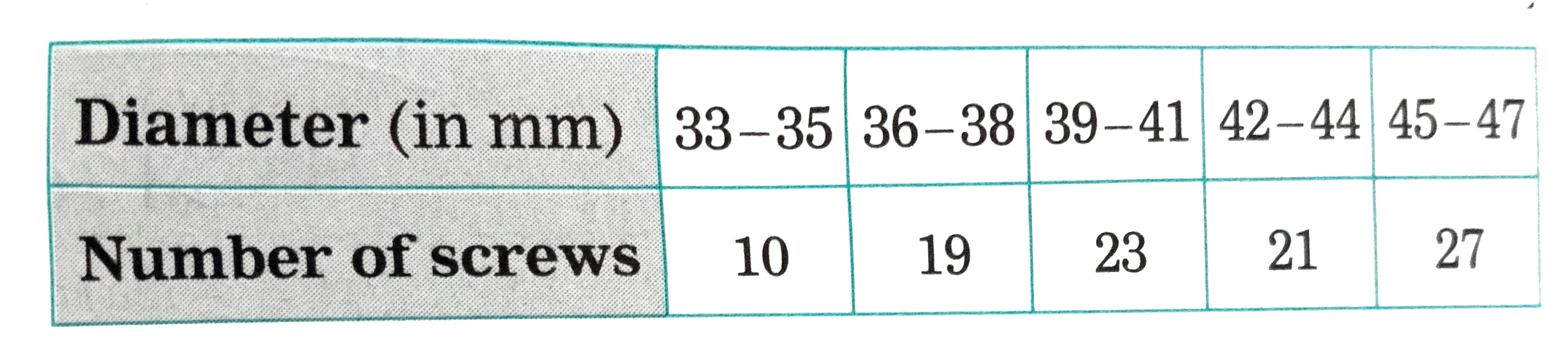
Calculate the mean diameter of the screw by ' Assumed Mean Method' .
Steps to calculate mean by this method :
(1) Assume mean A. In this example , A = 40. (Generally, the class mark of the class having the maximum frequency or the class mark of the middle class is chosen as the assumed mean.)
(2) In column 1, write the class intervals,(Here, diameter).
(3) In column 2, write the corresponding class marks.
(4) In column 3, write the value of di, where di=xi−A.
A is the assumed mean.
(5) In column 4, write the frequencies (fi). Write Σfi at the bottom of this column.
(6) In column 5, write the product (fi×di) and their sum Σfidi.
Calculate ¯d and ¯¯¯X using the following formulae :
¯d=ΣfidiΣfi. Mean¯¯¯X=A+¯d.
[d : mean of deviations.]
View SolutionMean of a frequency distribution (¯x) is 45. If Σfi=20 find Σfixi
View SolutionFine ¯u , if Σfiui252 and Σfi=420
View SolutionThe algebraic sum of the deviations of xifi from the mean is:
View SolutionThe algebraic sum of the deviations of xifi from the mean is:
View Solution
