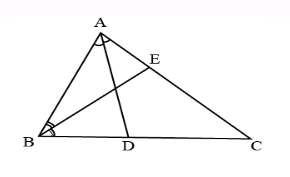
In ABC, ∠B=600,∠C=400, AL⊥BC and AD bisects ∠A such that L and D lie on side BC. Find ∠LAD.
To solve the problem step by step, we will follow the reasoning provided in the video transcript.
Step 1: Find Angle A in Triangle ABC
In triangle ABC, we know that the sum of the angles is 180 degrees. We are given:
- Angle B = 60 degrees
- Angle C = 40 degrees
Using the angle sum property:
Angle A+Angle B+Angle C=180∘
Substituting the known values:
Angle A+60∘+40∘=180∘
Angle A+100∘=180∘
Angle A=180∘−100∘=80∘
Step 2: Find Angle DAC
Since AD bisects Angle A, we can find Angle DAC:
Angle DAC=Angle A2=80∘2=40∘
Step 3: Find Angle D in Triangle ADC
In triangle ADC, we again use the angle sum property:
Angle A+Angle D+Angle C=180∘
We know:
- Angle A = 40 degrees (from step 2)
- Angle C = 40 degrees
Substituting these values:
40∘+Angle D+40∘=180∘
Angle D+80∘=180∘
Angle D=180∘−80∘=100∘
Step 4: Find Angle ADC
Since AL is perpendicular to BC, we have:
Angle ALD=90∘
Step 5: Use the Exterior Angle Theorem
According to the exterior angle theorem:
Angle ADC=Angle ALD+Angle LAD
We know:
- Angle ADC = 100 degrees (from step 3)
- Angle ALD = 90 degrees
Substituting these values:
100∘=90∘+Angle LAD
Angle LAD=100∘−90∘=10∘
Final Answer
Thus, the value of Angle LAD is:
Angle LAD=10∘
---
In triangle ABC, we know that the sum of the angles is 180 degrees. We are given:
- Angle B = 60 degrees
- Angle C = 40 degrees
Angle A+Angle B+Angle C=180∘
Substituting the known values:
Angle A+60∘+40∘=180∘
Angle A+100∘=180∘
Angle A=180∘−100∘=80∘
Since AD bisects Angle A, we can find Angle DAC:
Angle DAC=Angle A2=80∘2=40∘
In triangle ADC, we again use the angle sum property:
Angle A+Angle D+Angle C=180∘
We know:
- Angle A = 40 degrees (from step 2)
- Angle C = 40 degrees
40∘+Angle D+40∘=180∘
Angle D+80∘=180∘
Angle D=180∘−80∘=100∘
Since AL is perpendicular to BC, we have:
Angle ALD=90∘
According to the exterior angle theorem:
Angle ADC=Angle ALD+Angle LAD
We know:
- Angle ADC = 100 degrees (from step 3)
- Angle ALD = 90 degrees
100∘=90∘+Angle LAD
Angle LAD=100∘−90∘=10∘
Thus, the value of Angle LAD is:
Angle LAD=10∘
Topper's Solved these Questions
Similar Questions
In a parallelogram ABCD, the bisector of ∠A also bisects BC at X Find AB:AD
View SolutionIn Figure, ABC is a triangle in which ∠B=2∠C . D is a point on side BC such that AD bisects ∠BAC and AB=CD.BE is the bisector of ∠B . The measure of ∠BAC is
(a) 720
(b) 730
(c) 740
(d) 960
 View Solution
View SolutionIn Fig. 71, if ABCD and CDEF, find ∠ACE
View SolutionABCD is a cyclic quadrilateral in which: BCAD, ∠ADC=1100 and ∠BAC=500. Find ∠DAC ∠DBC=800 and ∠BAC=400. Find ∠BCD ∠BCD=1000and ∠ABD=700 find ∠ADB
View SolutionIn a parallelogram ABCD, the diagonals bisect each other at O. If ∠ABC=300, ∠BDC=100and ∠CAB=700, Find: ∠DAB, ∠ADC, ∠BCD, ∠AOD, ∠DOC, ∠BOC, ∠AOB, ∠ACD, ∠CAB, ∠ADB
Given that
∠ABC=302,∠ABC=∠ADC=300
[We know that measure of opposite angles are equal in a parallelogram]
∠BDC=100
∠CAB=700
∠BDA=∠ADB=∠ADC–∠BDC
302–102=202
∠DAB=1800–300=1500
∠ADB=∠DBC=200 (alternate angles)
∠BCD=∠DAB=1500
[we know, opposite angles are equal in a parallelogram]
∠DBA=∠BDC=100
[we know, Alternate interior angles are equal]
InΔABC
∠CAB+∠ABC+∠BCA=1800
[since, sum of all angles of a triangle is 180^0]
700+300+∠BCA=1800
∠BCA=1800–1000=800
∠DAB=∠DAC+∠CAB=700+800=1500
∠BCD=1500 (opposite angle of the parallelogram)
∠DCA=∠CAB=700
In ΔDOC∠BDC+∠ACD+∠DOC=1800
[since, sum of all angles of a triangle is 180^0]
100+700+∠DOC=1800
∠DOC=1800−800
∠ACB=800View SolutionIn a ABC, If LandM are points on ABandAC respectively such that LMparallelBC
Prove that: ar(LBC)=ar(MBC)View SolutionIn a ABC, If LandM are points on ABandAC respectively such that LMparallelBC
Prove that: ar(LCM)=ar(LBM)View SolutionIn a ABC , If LandM are points on ABandAC respectively such that LMparallel BC
Prove that: ar(LOB)=ar(MOC)View SolutionIn a triangle ∠ABC, ∠A=50∘, ∠B=60∘and∠C=70∘ . Find the measures of the angles of the triangle formed by joining the mid-points of the sides of this triangle.
View SolutionIn Fig.85, ABCD and ACBD. Find the value of x,y,z.
View Solution

