In a survey of 100 persons it was sound that 28 read magazine A, 30 readmagazine B, 42 read magazine C, 8 read magazines A & B, 10 read magazine A & C and 5 read magazine B&C and 3 read all the three. Find how many read none of three magazines?
To solve the problem step by step, we will use the principle of inclusion-exclusion to find the number of people who read at least one of the magazines and then subtract that from the total number of people surveyed to find those who read none of the magazines.
1. Identify the Total Number of People:
N(U)=100
This represents the total number of people surveyed.
2. Identify the Number of Readers for Each Magazine:
- Number of people reading magazine A:
N(A)=28
- Number of people reading magazine B:
N(B)=30
- Number of people reading magazine C:
N(C)=42
3. Identify the Number of Readers for Each Combination of Magazines:
- Number of people reading both A and B:
N(A∩B)=8
- Number of people reading both A and C:
N(A∩C)=10
- Number of people reading both B and C:
N(B∩C)=5
- Number of people reading all three magazines:
N(A∩B∩C)=3
4. Apply the Inclusion-Exclusion Principle:
The formula for the number of people reading at least one magazine is:
N(A∪B∪C)=N(A)+N(B)+N(C)−N(A∩B)−N(A∩C)−N(B∩C)+N(A∩B∩C)
Substituting the values:
N(A∪B∪C)=28+30+42−8−10−5+3
5. Calculate the Values:
- First, add the individual counts:
28+30+42=100
- Next, subtract the intersections:
100−(8+10+5)+3=100−23+3=80
6. Find the Number of People Who Read None of the Magazines:
To find the number of people who read none of the magazines, subtract the number of people who read at least one magazine from the total number of people surveyed:
N(none)=N(U)−N(A∪B∪C)=100−80=20
Final Answer:
The number of people who read none of the three magazines is:
20
N(U)=100
This represents the total number of people surveyed.
- Number of people reading magazine A:
N(A)=28
- Number of people reading magazine B:
N(B)=30
- Number of people reading magazine C:
N(C)=42
- Number of people reading both A and B:
N(A∩B)=8
- Number of people reading both A and C:
N(A∩C)=10
- Number of people reading both B and C:
N(B∩C)=5
- Number of people reading all three magazines:
N(A∩B∩C)=3
The formula for the number of people reading at least one magazine is:
N(A∪B∪C)=N(A)+N(B)+N(C)−N(A∩B)−N(A∩C)−N(B∩C)+N(A∩B∩C)
Substituting the values:
N(A∪B∪C)=28+30+42−8−10−5+3
- First, add the individual counts:
28+30+42=100
- Next, subtract the intersections:
100−(8+10+5)+3=100−23+3=80
To find the number of people who read none of the magazines, subtract the number of people who read at least one magazine from the total number of people surveyed:
N(none)=N(U)−N(A∪B∪C)=100−80=20
The number of people who read none of the three magazines is:
20
Topper's Solved these Questions
Similar Questions
In a survey of 60 persons, it is found that 25 read newspaper H, 26 read T, 26 read I, 9 read H and I, 11 and H and T,8 read T and I and 3 read all three newspaper. Find :
(a) No. of persons who read atleast one newspaper.
(b) No. of persons who read exactly one newspaper.View SolutionIn a survey of 60 persons, it is found that 25 read newspaper H, 26 read T, 26 read I, 9 read H and I, 11 and H and T,8 read T and I and 3 read all three newspaper. Find :
(a) No. of persons who read atleast one newspaper.
(b) No. of persons who read exactly one newspaper.View Solution
Knowledge Check
- Question 1 - Select One
A student a pages day for d day and then reads b page per day for 2d days . In terms of a,b,and d , how many pages did the student read ?
Aad+2bBad+2bdC2ad+2bdD2abd
In a city, three daily newspapers A,B,C are published. 42% of the people in that city read A, 51% read B and 68% read C. 30% read A and B, 28% read B and C ,36%read A and C. 8% do not read any of the three newspapers. The percentage of persons who read all the three papers is
View SolutionIn a school, 21 student read Maths, 26 reads Physics and 29 read Chemistry. If 14 students read Maths and Physics, 15 read Physics and Chemistry, 12 read Chemisry and Maths and 8 read all three subjects, then find the total students in the school.
View SolutionAll the people in a locality read the daily newspaper Indian Express or Hindustan Times or both If 120 read Indian Express and 150 read Hindustan Times and 36 read both , find ,
how many people are there in the locality.View SolutionAll the people in a locality read the daily newspaper Indian Express or Hindustan Times or both If 120 read Indian Express and 150 read Hindustan Times and 36 read both , find ,
how many people read only Indian Express.View SolutionIn a certain city of 15000 families, 3.5% of families who read A but not B look into advertisements, 25% of the families who read B but not A look into advertisements and 50% of the families, who read both A and B look into advertisements. It is known that 8000 families read A, 4000 read B and 1000 read both A and B. The number of families who read none of the newspaper are
View SolutionCells of emfs 10V and 20V are connected as shown. When an ammeter is connected across A and B its reads 1A, when connected across B and C it reads 5, when connected across A and C it reads 3A, find the resistance of the ammeter (in Ω)
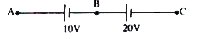 View Solution
View SolutionTimes, Mirror and Sun are three newspapers All readers of the Times read the Sun. Every person either reads the Sun or does not read the Mirror. 11 people read the Sun but does not read the Mirror. 8 people read either the Times or the Mirror but not both. 10 people read the Sun and either read the Mirror or do not read the Times. 14 people either read the Sun and not the Mirror or read both the Sun and Mirror. 9 people neither read the Times nor the Mirror. no. of people who read only none of them
View Solution

