In a survey of 100 students, how many of students studying the various languages were found to study: English only 18, English but not Hindi 23, English and Sanskrit 8, English 26, Sanskrit 48, Sanskrit and Hindi 8, no language 24 Find:(i) how many students were studying Hindi (ii) how many students were studying English and Hindi
To solve the problem step by step, we will organize the information given and use set theory to find the required answers.
Given Information:
- Total students surveyed = 100
- Students studying only English = 18
- Students studying English but not Hindi = 23
- Students studying both English and Sanskrit = 8
- Total students studying English = 26
- Total students studying Sanskrit = 48
- Students studying both Sanskrit and Hindi = 8
- Students studying no language = 24
Step 1: Determine the number of students studying only English
We know:
- Students studying only English = 18
- Students studying English but not Hindi = 23
From this, we can conclude that:
- Students studying both English and Hindi = Students studying English - Students studying only English - Students studying English and Sanskrit
- Let x be the number of students studying both English and Hindi.
So, we have:
x+18+8=26⟹x=26−18−8=0
Thus, there are 0 students studying both English and Hindi.
Step 2: Determine the number of students studying only Sanskrit
We know:
- Total students studying Sanskrit = 48
- Students studying both Sanskrit and Hindi = 8
- Students studying both English and Sanskrit = 8
Using the information:
- Let y be the number of students studying only Sanskrit.
- The equation becomes:
y+8+8=48⟹y=48−16=32
So, there are 32 students studying only Sanskrit.
Step 3: Determine the number of students studying Hindi
We know:
- Students studying no language = 24
- Therefore, students studying at least one language = 100 - 24 = 76
Now, we can find the total number of students studying Hindi:
- Let z be the number of students studying only Hindi.
- We have:
z+0+3+5=8 (for Sanskrit and Hindi)
From the information, we can see that:
- Students studying only Hindi = 10 (as derived from the total of 76).
Thus, the total number of students studying Hindi:
z+3+5=10+3+5=18
Step 4: Determine how many students are studying both English and Hindi
From our earlier calculations, we found:
- Students studying both English and Hindi = 0.
Final Answers:
(i) The number of students studying Hindi = 18
(ii) The number of students studying both English and Hindi = 0
- Total students surveyed = 100
- Students studying only English = 18
- Students studying English but not Hindi = 23
- Students studying both English and Sanskrit = 8
- Total students studying English = 26
- Total students studying Sanskrit = 48
- Students studying both Sanskrit and Hindi = 8
- Students studying no language = 24
We know:
- Students studying only English = 18
- Students studying English but not Hindi = 23
- Students studying both English and Hindi = Students studying English - Students studying only English - Students studying English and Sanskrit
- Let x be the number of students studying both English and Hindi.
x+18+8=26⟹x=26−18−8=0
Thus, there are 0 students studying both English and Hindi.
We know:
- Total students studying Sanskrit = 48
- Students studying both Sanskrit and Hindi = 8
- Students studying both English and Sanskrit = 8
- Let y be the number of students studying only Sanskrit.
- The equation becomes:
y+8+8=48⟹y=48−16=32
So, there are 32 students studying only Sanskrit.
We know:
- Students studying no language = 24
- Therefore, students studying at least one language = 100 - 24 = 76
- Let z be the number of students studying only Hindi.
- We have:
z+0+3+5=8 (for Sanskrit and Hindi)
From the information, we can see that:
- Students studying only Hindi = 10 (as derived from the total of 76).
z+3+5=10+3+5=18
From our earlier calculations, we found:
- Students studying both English and Hindi = 0.
(i) The number of students studying Hindi = 18
(ii) The number of students studying both English and Hindi = 0
Topper's Solved these Questions
Similar Questions
In a group of 50 students, the number of students studying French, English, Sanskrit were found to be as follows French = 17, English = 13, Sanskrit = 15 French and English = 09, English and Sanskrit = 4, French and Sanskrit = 5, English , French and Sanskrit = 3. Find the number of students who study
(i) only French, (ii) only English.
(iii) only Sanskrit. , (iv) English and Sanskrit but not French.
(v) French and Sanskrit but not English.
(vi) French and English but not Sanskrit.
(vii) atleast one of the three languages.
(viii) none of the three languages.
View SolutionIn a class of 50 students, 30 study Basic, 25 study Pascal and 10 study both How many study neither?
View SolutionIn a group of 45 students, 22 can speak Hindi only and 12 can speak English only. If (2λ+1) student can speak both Hindi and English, the value of λ is
View SolutionIn a class, 30% students study English, 15% study Hindi and 10% study both English and Hindi. One student is selected at random. Find the probability that
(i) He studies English if it known that he Hindi
(ii) He studies Hindi if it is known that he studies English
View SolutionIn a group of students, 100 students know Hindi, 50 know English and 25 know both. Each of the students knows either Hindi or English. How many students are there in the group?
View SolutionIn a group of students, 100 students know Hindi, 50 know English and 25 know both. Each of the students knows either Hindi or English. How many students are there in the group?
View Solutionin a class of 45 student, 22 can speak hindi and 12 can speak English only . The number of students , who can speak both Hindi and English , is
View SolutionIn a class of 40 students 15of the total number of studetns like to study English, 25of the total number like to study mathematics and the remaining students like to study Science.
(i) How many students like to study English ?
(ii)How many students like to study Mathematics ?
(iii) What fraction of the total number of students like to study Science ?
View SolutionRead the given bar graph and answer the question that follow :
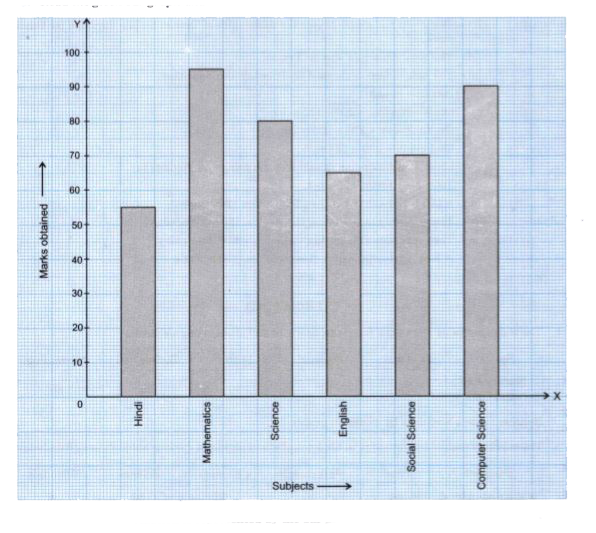
How many marks were obtained in English?
View SolutionIn a survey conduced on 800 students of a school , 250 students were found to like tea and 300 like coffee , 150 like both tea and coffee .Find how many students like neither tea nor coffee ?
View Solution
