In an equilateral triangle, the inradius, circumradius, and one of the exradii are in the ratio

A

$2 : 3 : 5$

B

$1 : 2 : 3$

C

$1 : 3 : 7$

D

$3 : 7 : 9$

Video Solution

Text Solution

Verified by Experts

Let a be the iength of each side of the triangle.
We have $, Δ = \frac{\sqrt{3}}{4} a^{2}$ and $s = \frac{3 a}{2}$
$∴ r = \frac{Δ}{s} = \frac{a}{2 \sqrt{3}}, R = \frac{a b c}{4 Δ} = \frac{a^{3}}{\sqrt{3} a^{2}} = \frac{a}{\sqrt{3}}$
and $r_{1} = \frac{Δ}{s - a} = \frac{\sqrt{3} / 4 a^{2}}{a / 2} = \frac{\sqrt{3}}{2} a$
$∴ r : R : r_{1} = \frac{a}{2 \sqrt{3}} : \frac{a}{\sqrt{3}} : \frac{\sqrt{3}}{2} a = 1 : 2 : 3$

|

Updated on:21/07/2023

Class 12 MATHS PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEM

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One

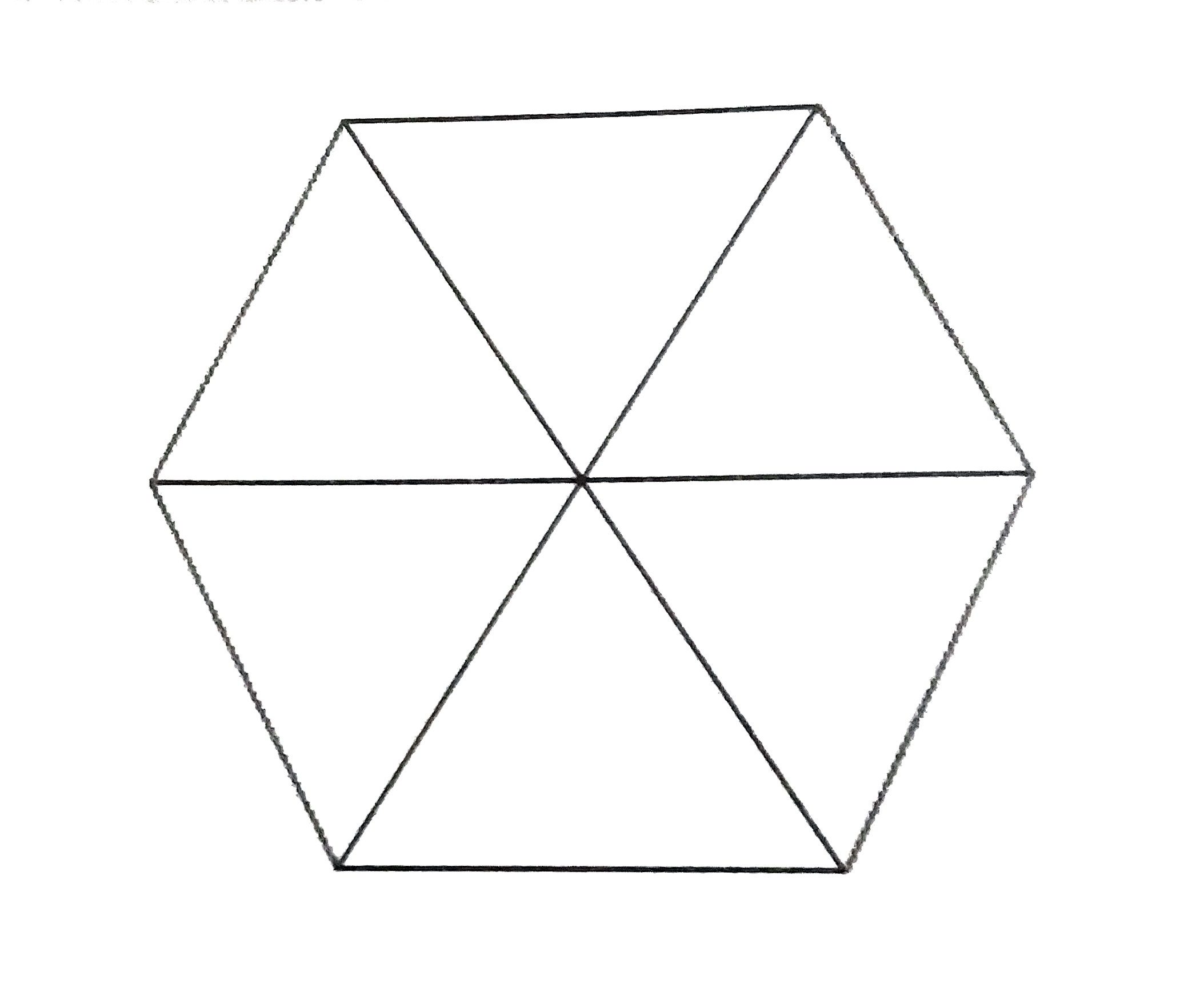
The regular hexagon shown above is divided into six congruent equilateral triangle. What is the measure, in degrees, of one of the interior angles of the hexagon?
A $60^{\circ}$
B $120^{\circ}$
C $180^{\circ}$
D $360^{\circ}$

OBJECTIVE RD SHARMA ENGLISH-PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEM-Chapter Test

Home
Ask Doubt
Profile