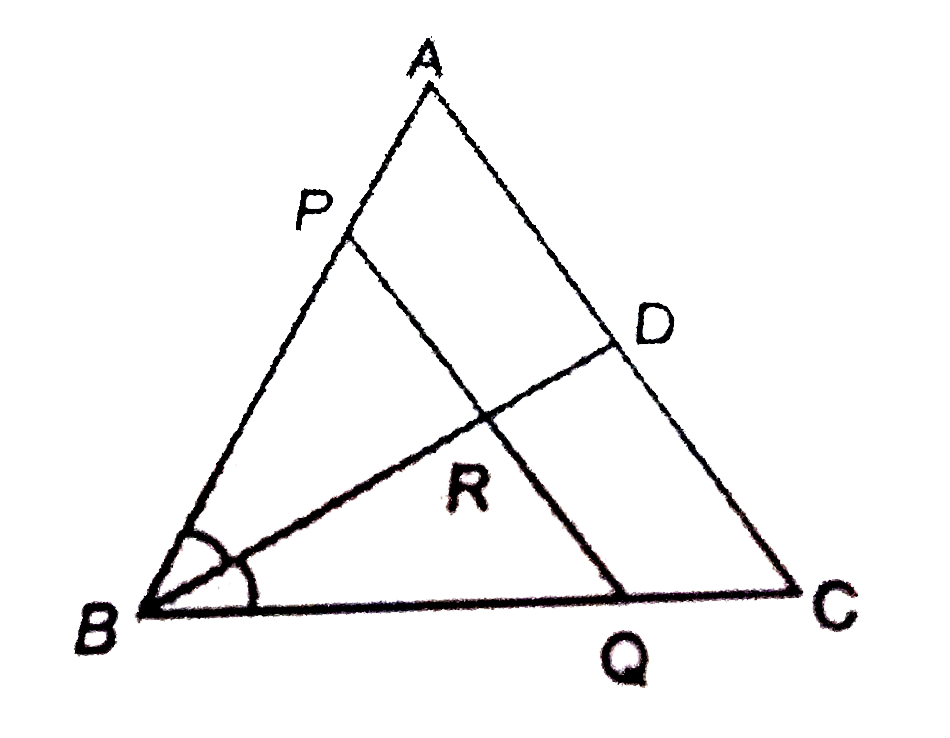
In ΔABC, the bisector of ∠B meets AC at D. A line PQ∣∣AC meets AB,BC and BD at P,Q and R respectively. Show that BP×QR=BQ×PR.
Video Solution
Text Solution
In ΔBQP,BR is the bisector of ∠B.
∴QRPR=BQBP [ by angle-bisector theorem]
|
Topper's Solved these Questions
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7B
19 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7C
13 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - EXERCISE 7 A
1 Videos
- T-RATIOS OF SOME PARTICULAR ANGLESBOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 11
27 Videos
- TRIGONOMETRIC IDENTITIESBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20 Videos
Similar Questions
Knowledge Check
In ΔABC, internal angle bisector of ∠A meet side BC in D. DE⊥AD meet AC in E and AB in F. then
AAE in H.M of b and cBAD=2bcb+ccos.A2CEF=4bcb+csin.A2DΔAEF is isoscelesABC is a cyclic triangle and the bisectors of ∠BAC,∠ABCand∠BCA meet the circle at P,Q and R respectively. Then the angle ∠RQP is:
A90∘−∠B2B90∘+∠B2C90∘+∠C2D90∘+∠B2In a ΔABC,∠C in an obtuse angle . The bisectors of the exterior angles at A and B meet BC and AC produced at D and E respectively . If AB=AD=BE. Find ∠ACB .
A105∘B108∘C110∘D135∘
In a ΔABC, the bisector of ∠ACBand∠ABC meet at D. The perpendicular bisectors of DB and DC meet BC at E and F respectively. Show that the perimeter of DEF is equal to BC
View SolutionIn a ΔABC,AD is a median. The bisectors of ∠ADBand∠ADC meets AB & AC at E and F respectively . AE:BE=3:4 . Find EF:BC.
View SolutionIn a ΔABC, the bisectors of ext ∠B and ext ∠C meet at D. If BD>CD, then prove that AC>AB.
View SolutionAD is a median of ΔABC . The bisector of ∠ADB and ∠ADC meet AB and AC in E and F respectively. Prove that EF||BC
View SolutionIn a ΔABC,∠C in an obtuse angle . The bisectors of the exterior angles at A and B meet BC and AC produced at D and E respectively . If AB=AD=BE. Find ∠ACB .
View Solution