In Fig: 6.9. lines PQ and RS intersect each other at point O. If ∠POR:∠ROQ=5:7,find the all the angles.
To solve the problem, we need to find all the angles formed by the intersection of lines PQ and RS at point O, given the ratio of angles ∠POR to ∠ROQ is 5:7.
1. Set up the angles based on the given ratio:
Let ∠POR=5x and ∠ROQ=7x.
Hint: Use a variable (like x) to represent the common factor in the ratio.
2. Use the property of linear pairs:
Since ∠POR and ∠ROQ are linear angles (they form a straight line), their sum is equal to 180∘:
∠POR+∠ROQ=180∘
Hint: Remember that linear angles always add up to 180∘.
3. Substitute the expressions for the angles:
Substitute the expressions we defined in step 1 into the equation:
5x+7x=180∘
Hint: Combine like terms to simplify the equation.
4. Solve for x:
Combine the terms:
12x=180∘
Now, divide both sides by 12:
x=180∘12=15∘
Hint: When dividing, ensure you perform the operation correctly to isolate x.
5. Calculate ∠POR:
Substitute x back into the expression for ∠POR:
∠POR=5x=5×15∘=75∘
Hint: Always double-check your multiplication to ensure accuracy.
6. Calculate ∠ROQ:
Substitute x back into the expression for ∠ROQ:
∠ROQ=7x=7×15∘=105∘
Hint: Use the same method as before to find the second angle.
7. Find the vertically opposite angles:
Since ∠QOS is vertically opposite to ∠POR, we have:
∠QOS=∠POR=75∘
Similarly, since ∠POS is vertically opposite to ∠ROQ:
∠POS=∠ROQ=105∘
Hint: Remember that vertically opposite angles are always equal.
Final Angles:
- ∠POR=75∘
- ∠ROQ=105∘
- ∠QOS=75∘
- ∠POS=105∘
Let ∠POR=5x and ∠ROQ=7x.
Since ∠POR and ∠ROQ are linear angles (they form a straight line), their sum is equal to 180∘:
∠POR+∠ROQ=180∘
Substitute the expressions we defined in step 1 into the equation:
5x+7x=180∘
Combine the terms:
12x=180∘
Now, divide both sides by 12:
x=180∘12=15∘
Substitute x back into the expression for ∠POR:
∠POR=5x=5×15∘=75∘
Substitute x back into the expression for ∠ROQ:
∠ROQ=7x=7×15∘=105∘
Since ∠QOS is vertically opposite to ∠POR, we have:
∠QOS=∠POR=75∘
Similarly, since ∠POS is vertically opposite to ∠ROQ:
∠POS=∠ROQ=105∘
- ∠POR=75∘
- ∠ROQ=105∘
- ∠QOS=75∘
- ∠POS=105∘
Topper's Solved these Questions
- BOOK - NCERT ENGLISHCHAPTER - LINES AND ANGLESEXERCISE - Exercise 6.16videos
LINES AND ANGLES
- BOOK - NCERT ENGLISHCHAPTER - LINES AND ANGLESEXERCISE - EXERCISE 6.26videos
LINES AND ANGLES
- BOOK - NCERT ENGLISHCHAPTER - LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Exercise 4.12videos
LINEAR EQUATIONS IN TWO VARIABLES
- BOOK - NCERT ENGLISHCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 10.121videos
NCERT THEOREMS
Similar Questions
Lines PQ and RS intersect each other at point O. If ∠POR:∠ROQ=5:7, find all the angles.
View SolutionLines PQ and RS intersect each other at point O. If ∠POR:∠ROQ=5:7, find all the angles.
View SolutionIn Figure, two straight lines PQ and RS intersect each other at O. If ∠POT=750, find the values of a,b and c
View SolutionIn Fig. 12, two straight lines PQ and RS intersect each other at O. If ∠POT=750, find the values of a,b and c.
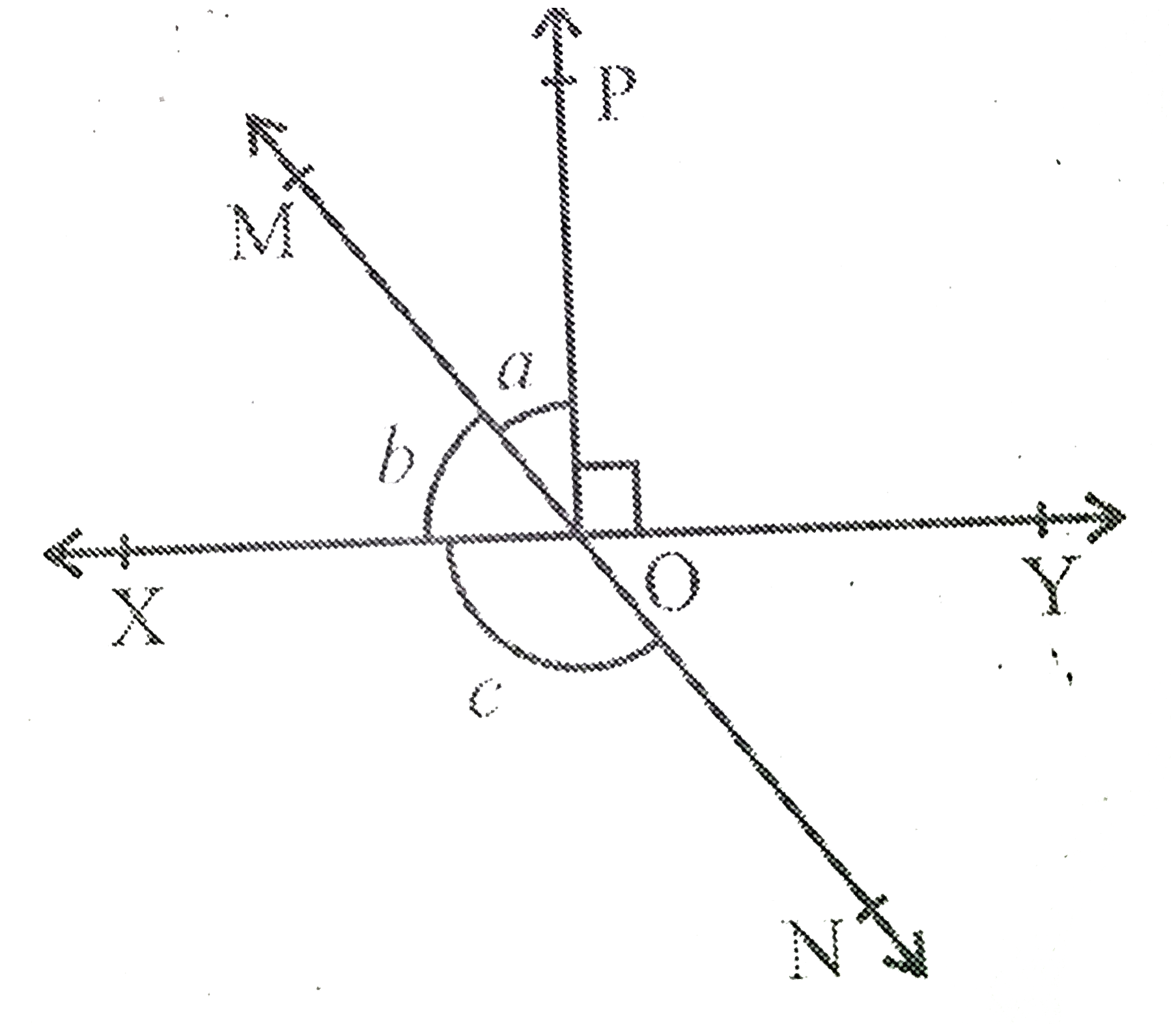
View SolutionIn fig: 6.14, lines XY and MN intersect at O. If ∠POY=90∘and a:b=2:3, find c.
 View Solution
View SolutionIn Fig. 6.13, lines AB and CD intersect at O. If ∠AOC +∠BOE=70∘and ∠BOD=40∘, find ∠BOE and reflex ∠COE.
 View Solution
View SolutionDiagonals of a trapezium PQRS intersect each other at the point 0 ,PQ||RS and PQ=3 RS. Find the ratio of the areas of ΔPOQ and ΔROS.
View SolutionPQ and RS are two parallel chords of a circle and lines RP and SQ intersect each other at O as shown in Figure. Prove that OP=OQ.
View SolutionPQRS is a square. PR and SQ intersect at O. State the measure of ∠POQ.
View SolutionPQRS is a square. PR and SQ intersect at O. State the measure of ∠POQ.
View Solution

