In fig., D is a point on hypotenuse AC of $Δ A B C, D M ⊥ B C$ and $D N ⊥ A B$ . Prove that
(i) $D M^{2} = D N \cdot M C$
(ii) $D N^{2} = D M \cdot A N$

Video Solution

Text Solution

Verified by Experts

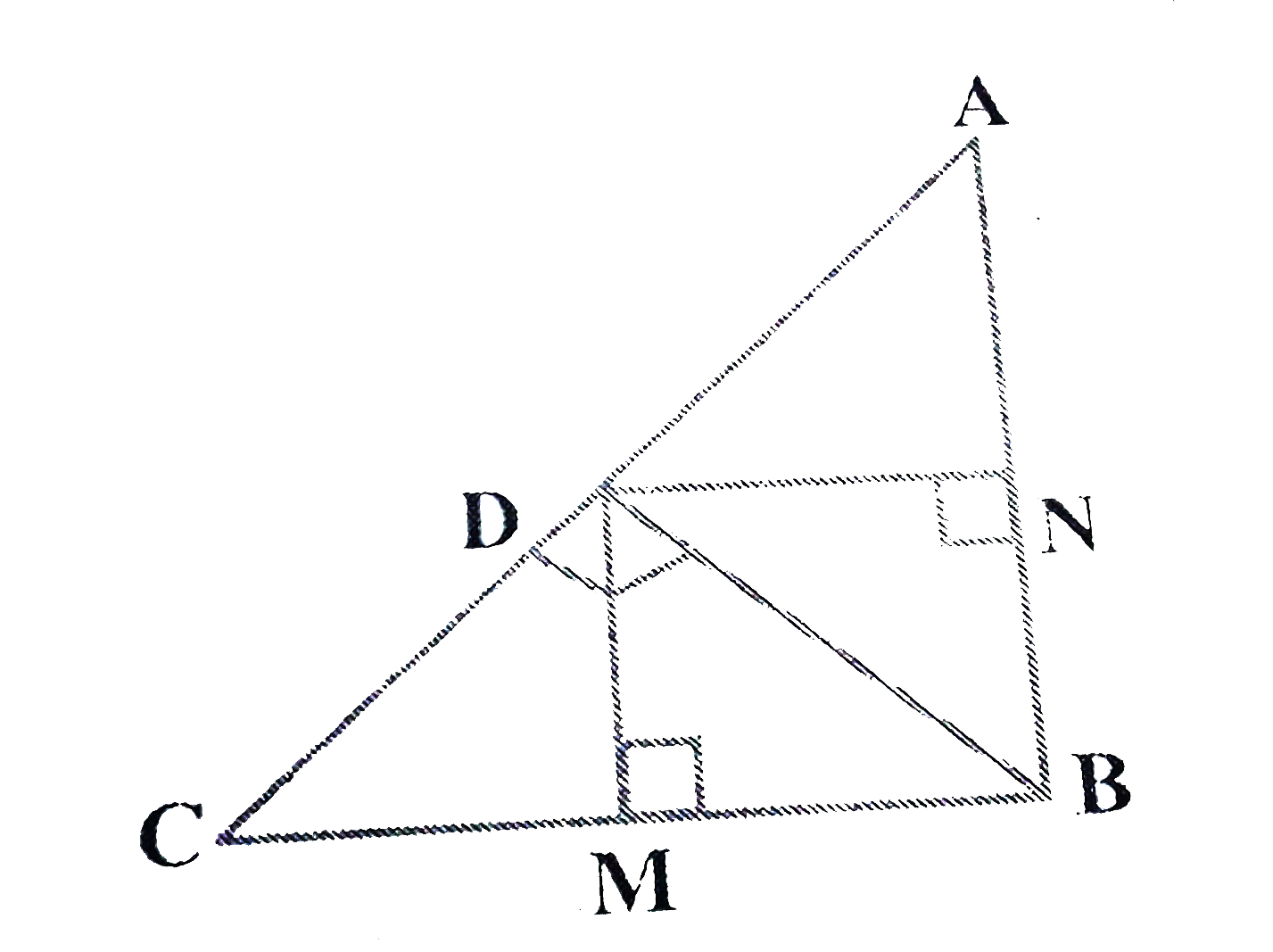
1)BNDM is rectangle
BM=DN
DM=BN
In $∠ D M C and ∠ B M D$
$∠ C = ∠ D$
$∠ M = ∠ M$
$∠ D M C and ∠ B M D$ (AAA)
$\frac{D M}{B M} = \frac{M C}{M D}$
DMMD=MCDN
DM^2=MCDN.
Similarly, DN^2=DMAN.

Updated on:21/07/2023

|

Class 10 MATHS TRIANGLES

Similar Questions

In Figure, $A B C$ is a right triangle right angled at $B$ and $D$ is the foot of the the perpendicular drawn from $B$ on $A C$ . If $D M ⊥ B C$ and $D N ⊥ A B$ , prove that: $(i) D M^{2} = D N \times M C$ $(i i) D N^{2} = D M \times A N$
View Solution
$A B C D$ is a rectangle. Points $M$ and $N$ are on $B D$ such that $A M ⊥ B D$ and $C N ⊥ B D$ . Prove that $B M^{2} + B N^{2} = D M^{2} + D N^{2}$ .
View Solution
In Figure $A B D C a n d A D B C .$ Prove that $∠ D A B = ∠ D C B$
View Solution
In Figure, it is given that $A B = C D a n d A D = B C .$ Prove that $A D C ≅ C B A$ .
View Solution
In Figure, $A D = B C a n d B D = C A$ . Prove that ∠A D B= ∠B C A a n d ∠D A B=∠ C B A.
View Solution
In Figure, $B M$ and $D N$ are both perpendiculars to the segments $A C$ and $B M = D N$ . Prove that $A C$ bisects $B D .$ Figure
View Solution
In right triangle $A B C$ , right angle at $C, M$ is the mid-point of the hypotenuse $A B .$ $C$ is jointed to $M$ and produced to a point $D$ such that $D M = C M .$ Point $D$ is joined to point $B .$ Show that $A M C ≅ B M D$ (ii) $∠ D B C = ∠ A C B$ $D B C ≅ A C B$ (iii) $C M = \frac{1}{2} A B$
View Solution
ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that (i) D is the mid-point of AC (ii) $M D ⊥ A C$ (iii) $C M = M A = \frac{1}{2} A B$
View Solution
In Fig.11, it is given that $A B = C D a n d A D = B C .$ Prove that $A D C ≅ C B A .$
View Solution
In Figure, $A B = A C a n d D B = D C .$ Prove that $∠ A B D = ∠ A C D$
View Solution

Recommended Questions

Home
Ask Doubt
Profile