In Figure, ABC is an equilateral triangle of side 8cm. A, B and C are the centres of circular arcs of radius 4cm. Find the area of the shaded region correct upto 2 decimal places.
Video Solution
Text Solution
Given : side of equilateral triangle a=8cm and radius of circular arcs r=4cm
Area of equilateral triangle =√34×a2
∴Area of equilateral triangle =√34×(82)=27.71
θ=600(triangle is equilateral )
Area of circular arc=(θ360)×π×r2
Area of circular arc=(60360)×(227)×42=8.38cm2
∴ Area of circular 3 arcs =3×8.38=25.14cm2
Area of the shaded region= Area of equilateral triangle - Area of circular 3 arcs
∴ Area of the shaded region =27.71−25.14=2.57cm2
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
ABC is an equilateral triangle of side x cm. The radius of its incircle is
 Ax√3cmB√3xcmC2x√3cmDx2√3cm
Ax√3cmB√3xcmC2x√3cmDx2√3cmFind the area of an equilateral triangle whose side is a cm.
A√34a2 cm2B√24a2 cm2C√35a2 cm2D√25a2 cm2
Find the area of an equilateral triangle of side 6 cm.
View SolutionFind the area of an equilateral triangle of side 6 cm.
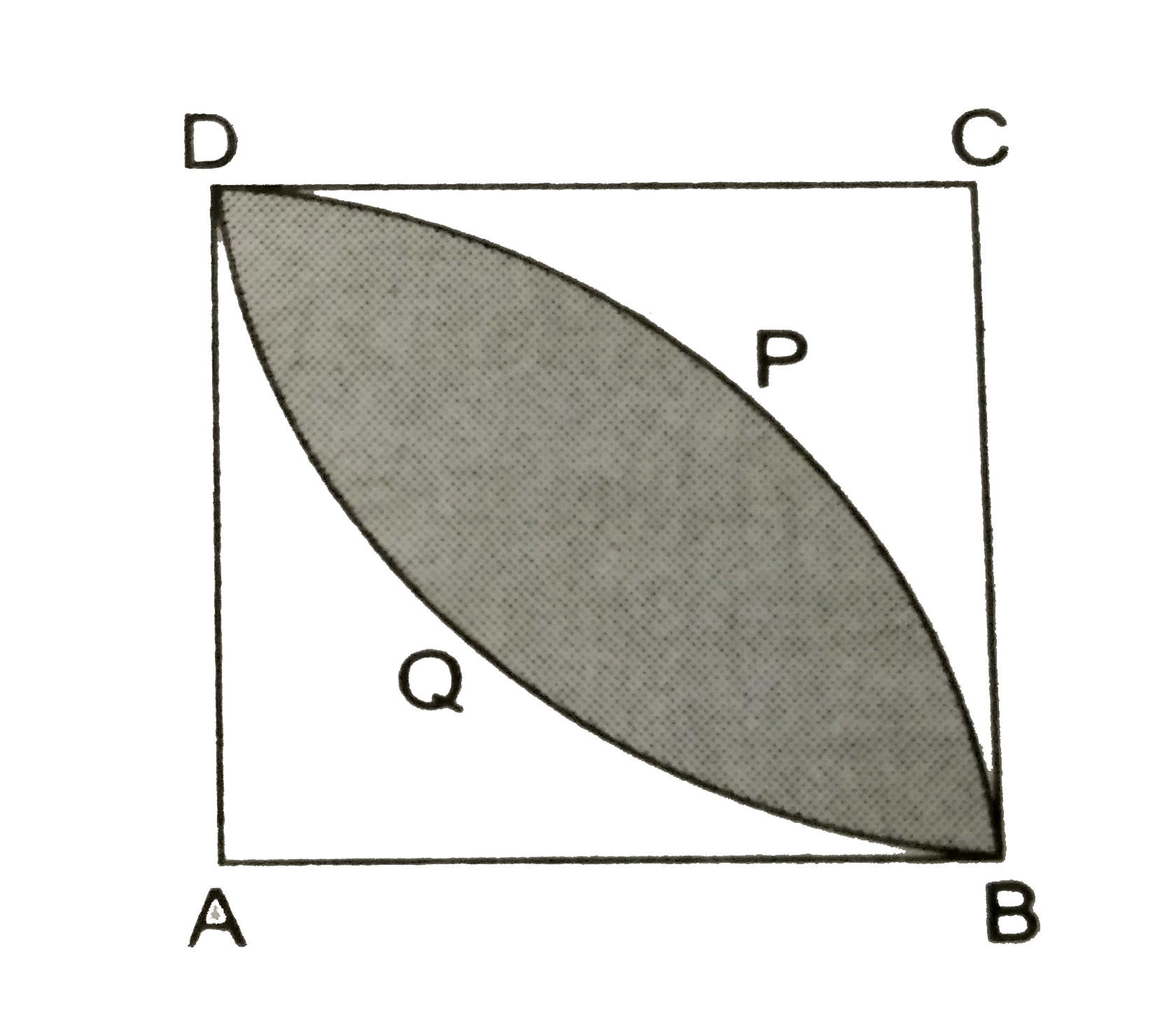
View SolutionIn the given figure, ABCD is a square of side 7 cm. DPBA and DQBC are quardrants of circles each of the radius 7 cm. Find the area of the shaded region.
View SolutionIn the given figure, ΔPQR is an equilateral triangle of side 8cmP,Q,R are centres of circular arcs each of radius 4cm find the area of shaded region. (use π=3.14and√3=1.732)
View SolutionIn the given figure, ABCD is a square of side 7cm, DPBA and DQBC are quadrants of circles each of the radius 7 cm. Find the area of shaded region.
View SolutionABC is an equilateral triangle inscribed in a circle of radius 4 cm. Find the area of the shaded portion.

View Solution
