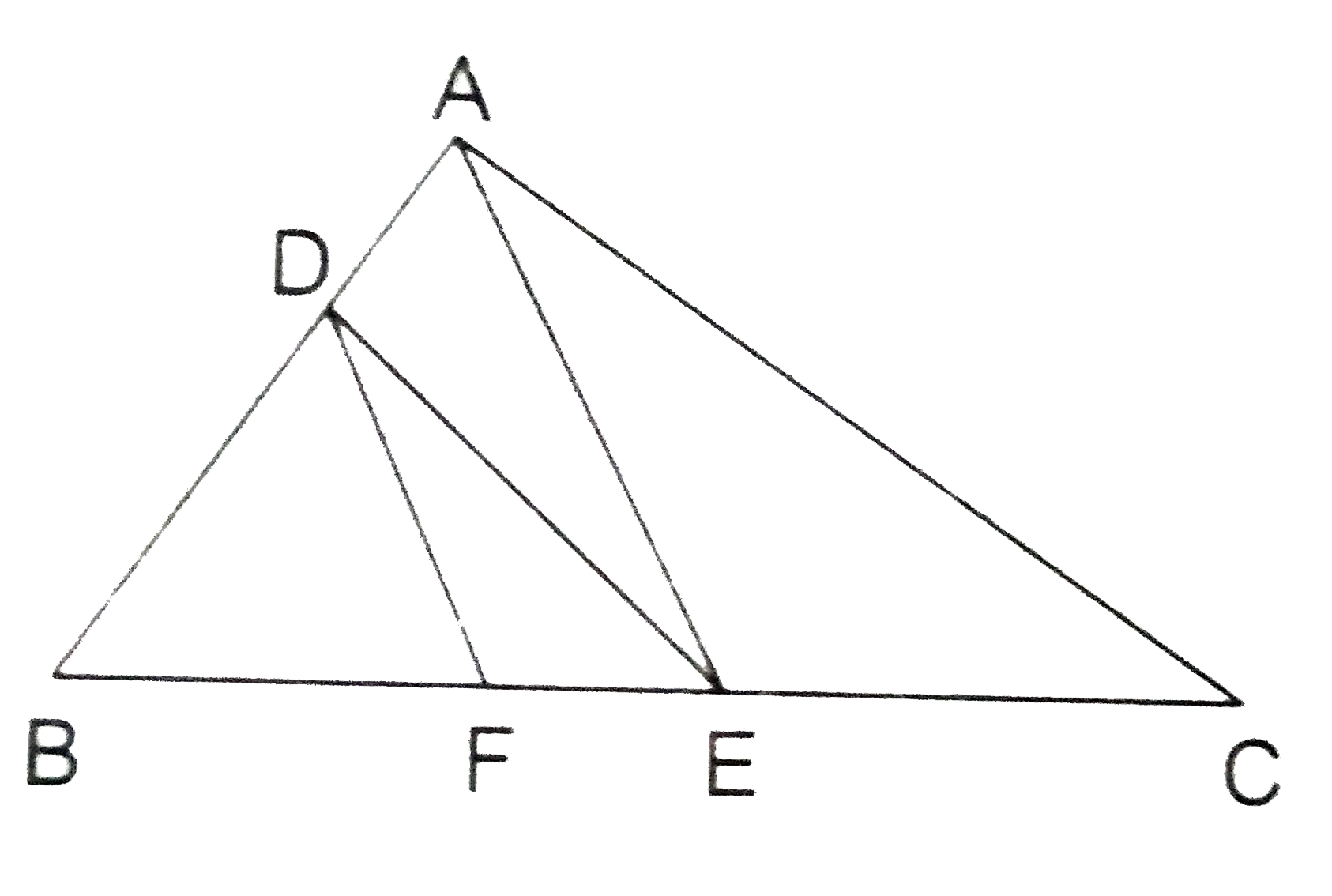
In Figure, DE∣∣AC and DF∣∣AE . Prove that EFBF=ECBE .
To prove that EFBF=ECBE given that DE∥AC and DF∥AE, we will use the properties of similar triangles and the Basic Proportionality Theorem (also known as Thales' theorem).
1. Identify the Triangles:
We have two triangles, △ABC and △ABE. The lines DE and DF are parallel to AC and AE respectively.
2. Apply the Basic Proportionality Theorem:
Since DE∥AC, by the Basic Proportionality Theorem, we can state that:
BEEC=BFFA
Let's denote this as Equation (1).
3. Apply the Basic Proportionality Theorem Again:
Now, considering triangle ABE with DF∥AE, we can apply the Basic Proportionality Theorem again:
BFFE=BDDA
Let's denote this as Equation (2).
4. Relate the Ratios:
From Equation (1), we can express EC in terms of BE:
EC=BE⋅FABF
From Equation (2), we can express FE in terms of BF:
FE=BF⋅DABD
5. Combine the Ratios:
Now we can combine the ratios from both equations:
EFBF=ECBE
6. Conclusion:
Thus, we have proved that:
EFBF=ECBE
We have two triangles, △ABC and △ABE. The lines DE and DF are parallel to AC and AE respectively.
Since DE∥AC, by the Basic Proportionality Theorem, we can state that:
BEEC=BFFA
Let's denote this as Equation (1).
Now, considering triangle ABE with DF∥AE, we can apply the Basic Proportionality Theorem again:
BFFE=BDDA
Let's denote this as Equation (2).
From Equation (1), we can express EC in terms of BE:
EC=BE⋅FABF
From Equation (2), we can express FE in terms of BF:
FE=BF⋅DABD
Now we can combine the ratios from both equations:
EFBF=ECBE
Thus, we have proved that:
EFBF=ECBE
Topper's Solved these Questions
Similar Questions
In the given figure, DE||ACandDF||AE.
Prove that BFFE=BEEC
 View Solution
View SolutionIn Figure DEAC and DCap. Prove that BEEC=BCCP
View SolutionIn Figure, AB||CF and BC||ED . Prove that ∠ABC= ∠FDE
View SolutionIn Fig. if EF||DC||AB. Prove that AEED=BFFC . (FIGURE)
View SolutionIn Fig. if EF||DC||AB. Prove that AEED=BFFC . (FIGURE)
View SolutionIn Figure, it is given that AB=BC and AD=EC . Prove that ABE≅CBD
View SolutionIn Figure, AC=AE, AB=AD and ∠BAD=∠EAC. Prove that BC=DE
View SolutionIn Figure, DEFG is a square and ∠BAC=90 . Prove that (i) A G F ~ D B G(ii)AGF~EFC (iii) D B G ~ E F C(iv)DE2=BDxEC
View SolutionIn Figure, DE∣∣BCandAD:DB=4:5. Find Area(DEF)Area(CFB).
View SolutionIn Figure, AD, and BE are medians of ABC and BE∣∣DF. Prove that CF=14AC.
View Solution

