Question

In Figure, ${\displaystyle DE\mid \mid BC\text{and}AD:DB=4:5.}$
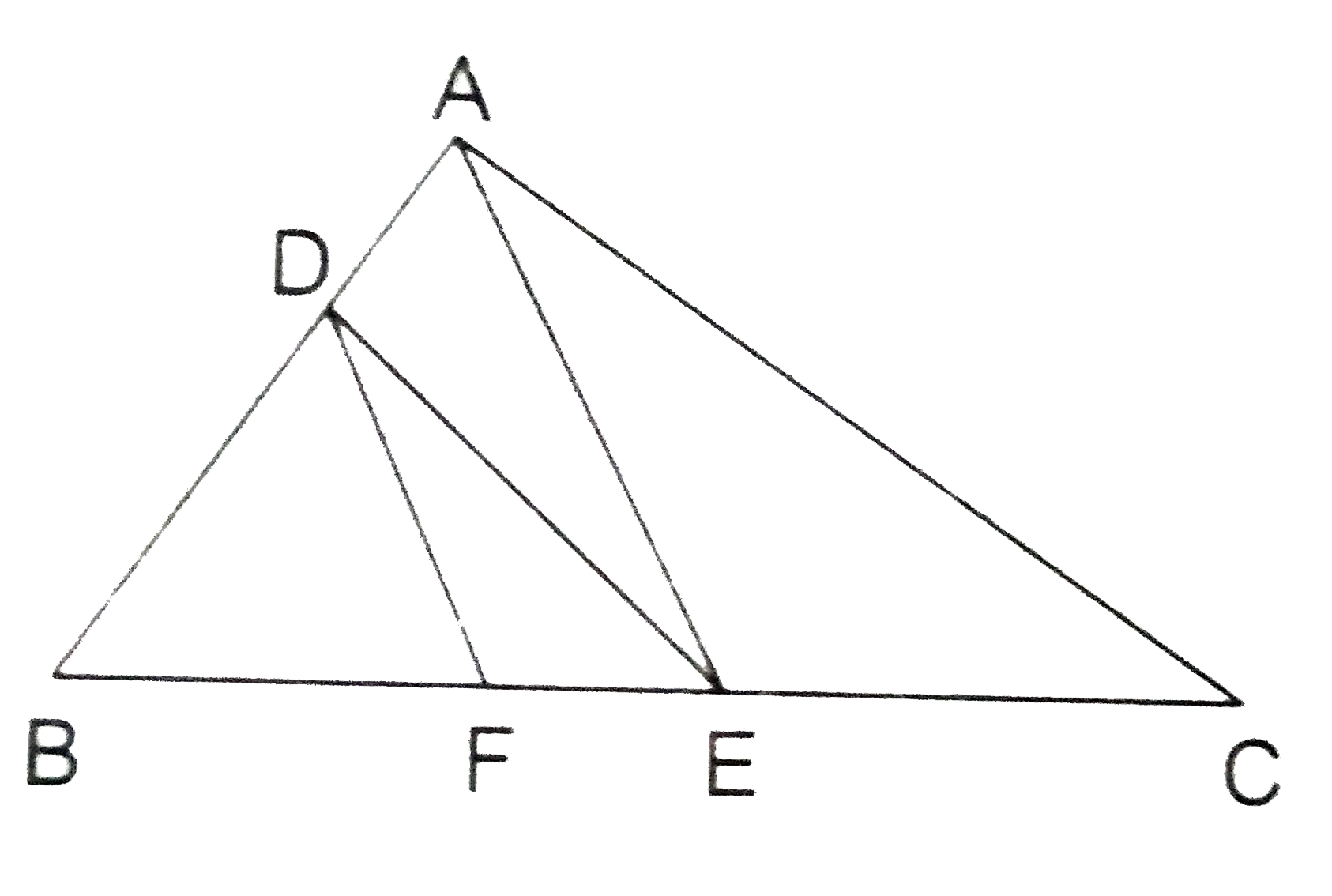
Find ${\displaystyle \frac{Area\left(DEF\right)}{Area\left(CFB\right)}\stackrel{.}{}}$

Explanation

To solve the problem, we need to find the ratio of the areas of triangles $$DEF$$ and $$CFB$$ given that $$DE\parallel BC$$ and the ratio $$AD:DB=4:5$$.

1. Identify Similar Triangles:
Since \( DE \parallel BC \), triangles \( ADE \) and \( ABC \) are similar by the AA (Angle-Angle) similarity criterion. This is because they share angle \( A \) and the corresponding angles \( ADE \) and \( ABC \) are equal.

Hint: Look for parallel lines and corresponding angles to establish similarity.

2. Set Up the Ratios:
From the similarity of triangles \( ADE \) and \( ABC \), we have:
\( \frac{AD}{AB} = \frac{DE}{BC} \)
Given \( AD : DB = 4 : 5 \), we can express \( AD \) and \( DB \) in terms of a common variable. Let \( AD = 4x \) and \( DB = 5x \). Thus, \( AB = AD + DB = 4x + 5x = 9x \).

Hint: Use a variable to express the segments in terms of a single ratio.

3. Calculate the Ratio of Sides:
Now we can find:
\( \frac{AD}{AB} = \frac{4x}{9x} = \frac{4}{9} \)
Therefore, we have:
\( \frac{DE}{BC} = \frac{4}{9} \)

Hint: Ensure to simplify the fractions correctly.

4. Identify Areas of Similar Triangles:
Next, we consider triangles \( DFE \) and \( CFB \). Since \( DE \parallel BC \), triangles \( DFE \) and \( CFB \) are also similar by AA similarity (alternate interior angles and vertically opposite angles).

Hint: Look for alternate interior angles when establishing similarity between the smaller triangles.

5. Set Up the Area Ratio:
The ratio of the areas of similar triangles is equal to the square of the ratio of their corresponding sides:
\( \frac{\text{Area}(DEF)}{\text{Area}(CFB)} = \left(\frac{DE}{BC}\right)^2 \)
Substituting the ratio we found:
\( \frac{\text{Area}(DEF)}{\text{Area}(CFB)} = \left(\frac{4}{9}\right)^2 = \frac{16}{81} \)

Hint: Remember that the area ratio involves squaring the ratio of corresponding sides.

6. Final Answer:
Thus, the required ratio of the areas is:
\( \frac{\text{Area}(DEF)}{\text{Area}(CFB)} = \frac{16}{81} \)