In figure, ⌢AB≅⌢AC and O is the centre of the circle,Prove that OA is the perpendicualr bisector of BC.
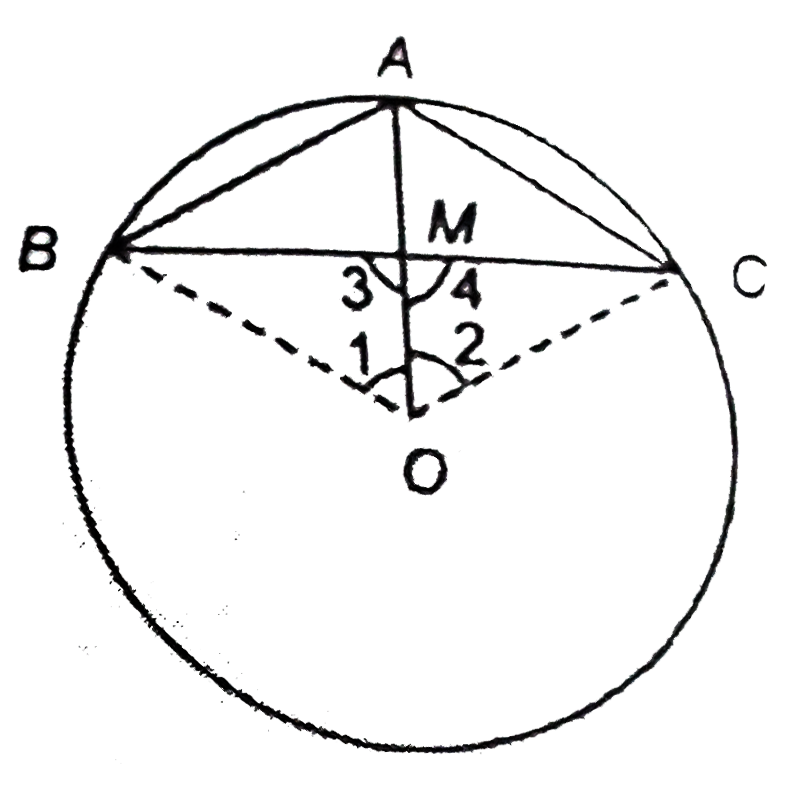
Since ⌢AB≅⌢AC (given)
⇒AB=AC (corresponding chords of congruent arcs are equal)
In ΔOAB and ΔOAC,
∵ ⎧⎪⎨⎪⎩AB=AC(just proved)OA=OA(common)OB=OC(each radii)
∴ΔOAB≅ΔOAC (by SSS congruency)
∴∠1=∠2 (c.p.c.t)
Now, in ΔOMB and ΔOMC,
∵ ⎧⎪⎨⎪⎩OB=AC(each radii)∠1=∠2(just proved)OM=OM(common)
∴ΔOMB≅ΔOMC (by S.A.S. congruency)
∴BM=MC (c.p.c.t) .......(1)
Also, ∠3=∠4 (c.p.c.t)
But ∠3+∠4=180∘ (L.P.A.)
⇒∠3=∠4=90∘ ......(2)
From (1) and (2) , we get
OM is the perpendicular bisector of BC.
⇒OA is the perpendicular bisector of BC.
Topper's Solved these Questions
- CIRCLE BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise 10a
22 Videos
- CIRCLE BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise 10b
19 Videos
- AREA OF PARALLELOGRAMS AND TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Revision Exercise (long Answer Question)
5 Videos
- CO-ORDINATE GEOMETRY BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CO-ORDINATE GEOMETRY EXERCISE - Exercise
8 Videos
Similar Questions
In Figure, ˆAB≅ˆAC and O is the centre of the circle. Prove that OA is the perpendicular bisector of BC
View SolutionIn Figure, O is the centre of the circle, prove that ∠x=∠y+∠z
View SolutionIn figure O is the centre of the circle, prove that ∠z=∠x+∠y.

View SolutionIn the figure, O is the center of the circle and BO is the bisector of ∠ABC show that AB=BC
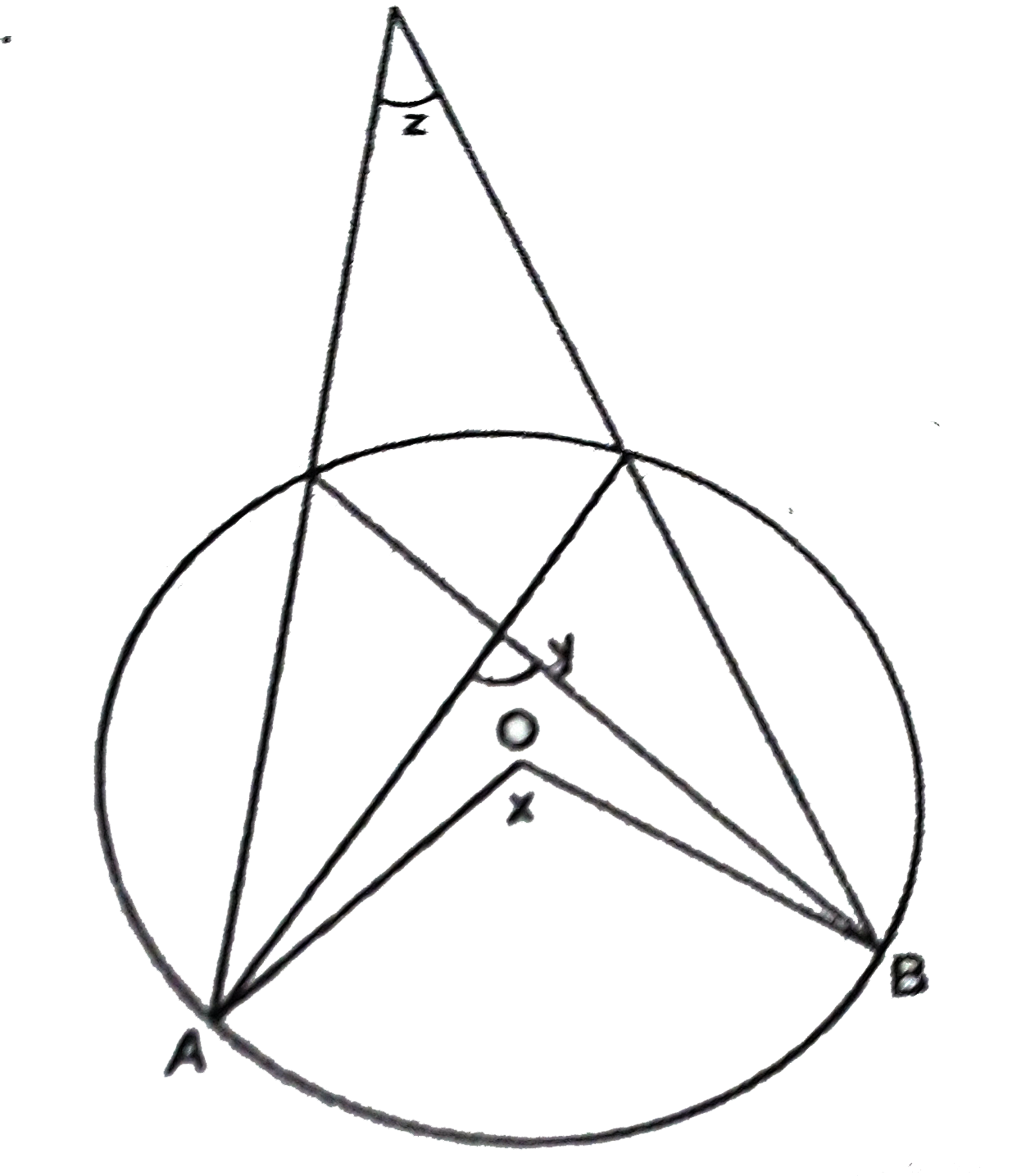
View SolutionIn the adjoining figure, O is the centre of the circle, Prove that ∠x=∠y+∠z.
View Solution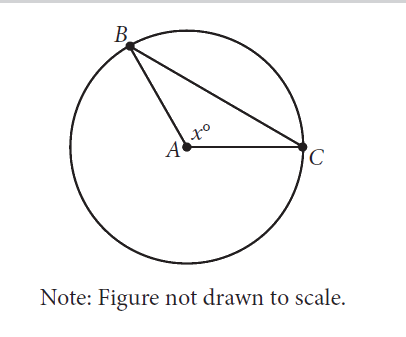
In the circle above, point A is the center and the length of arc ⌢BC is 25 of the circumference of the circle. What is the value of x ?
View SolutionIn the figure, AD is perpendicular to BC, prove that AB+AC>2AD.

View SolutionPerpendicular bisectors of the sides AB and AC of a triangle ABC meet at O.
Does the perpendicular bisector of BC pass through O?
View SolutionPoints A and B lie on a circle with radius 1, and arc ⌢AB has length π3. What fraction of the circumference of the circle is the length of arc ⌢AB ?
View SolutionIn the given figure AB is the diameter of a circle with centre O . If chord AC = chord AD, prove that :
(i) are BC=areDB
(ii) AB is bisector of ∠CAD.
Further, if the length of are AC is twice the length of are BC , find :
(i) ∠BAC
(ii) ∠ABC
View Solution
