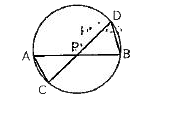
In Figure, two chords AB and CD intersect each other at the point P. Prove that:
(i) ΔAPC~ΔDPB
(ii) AP⋅PB=CP⋅DP

(i) In the given figure,
∠APC=∠DPB --> as opposite angles between two lines are equal
∠CAB=∠CDB --> as angle subtended by an arc are always equal on any point of circle
It means, ∠CAP=∠PDB
In ΔAPC and ΔDPB
∠APC=∠DPB
∠CAB=∠CDB
Thus, by Angle-Angle Similarity rule,
ΔAPC ~ ΔDPB
(ii) ΔAPC ~ ΔDPB
APDP=CPPB
Hence,AP.PB=CP.DP
Similar Questions
Knowledge Check
- Question 1 - Select One
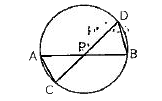
Two chords AB and CD of a circle intersects each other at P. which of the following is correct?
 AAP×PB=CP×PDBAP+PB=CP×PDCAP−PB=CP−PDDAP×CP=BP×PD
AAP×PB=CP×PDBAP+PB=CP×PDCAP−PB=CP−PDDAP×CP=BP×PD
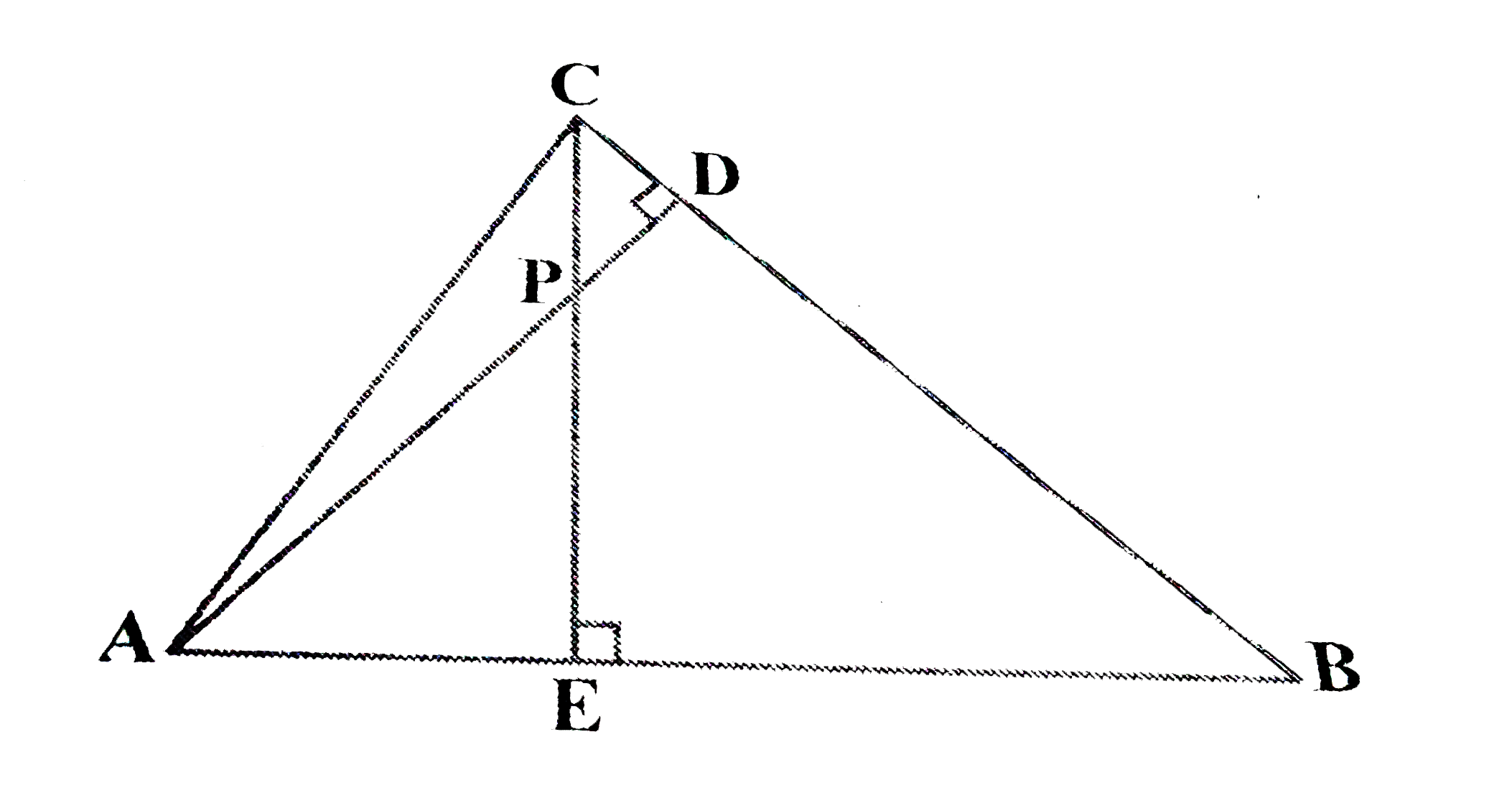
In Figure altitudes AD and CE of DABC intersect each other at the point P. Show that:
(i) ΔAEP~ΔCDP
(ii) ΔABD~ΔCBE
(iii) ΔAEP~ΔADB
(iv) ΔPDC~ΔBEC
 View Solution
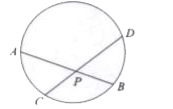
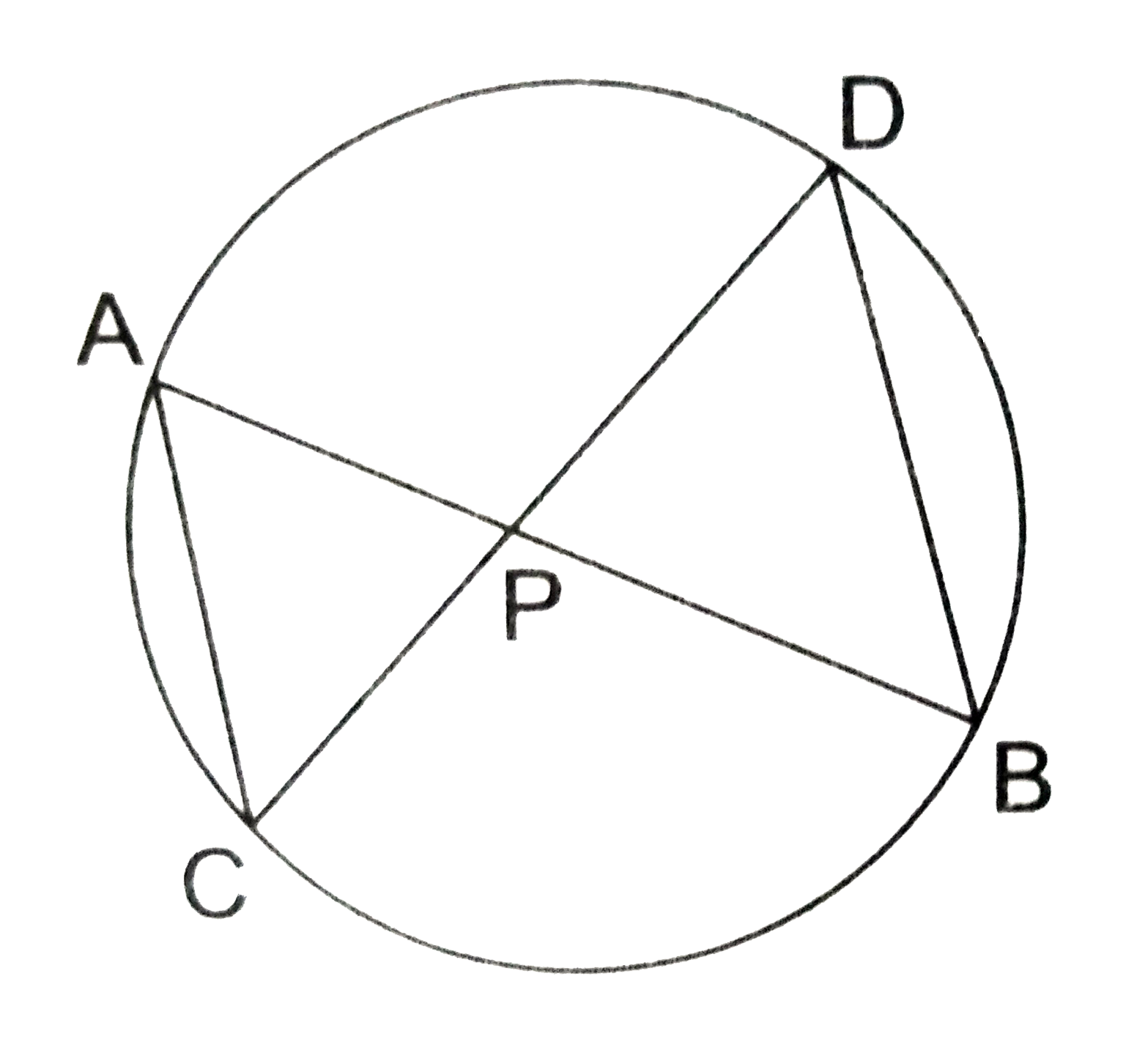
View SolutionIn a cricle, two chords AB and CD intersect at a point P inside the circle. Prove that
(a)ΔPAC~ΔPDB (b)PA⋅PB=PC⋅PD.
 View Solution
View SolutionIn Figure two chords AB and CD of a circle intersect each other at the point P (when produced) outside the circle. Prove that
(i) ΔPAC~ΔPDB
(ii) PA.PB=PC.PD
 View Solution
View SolutionIn trapezium AC and BD intersect each other at point P. Then prove that A(ΔABP)A(ΔCPD)=AB2CD2.
View SolutionIn Figure, AB and CD are two chords of a circle, intersecting each other at P such that AP=CP. Show that AB=CD
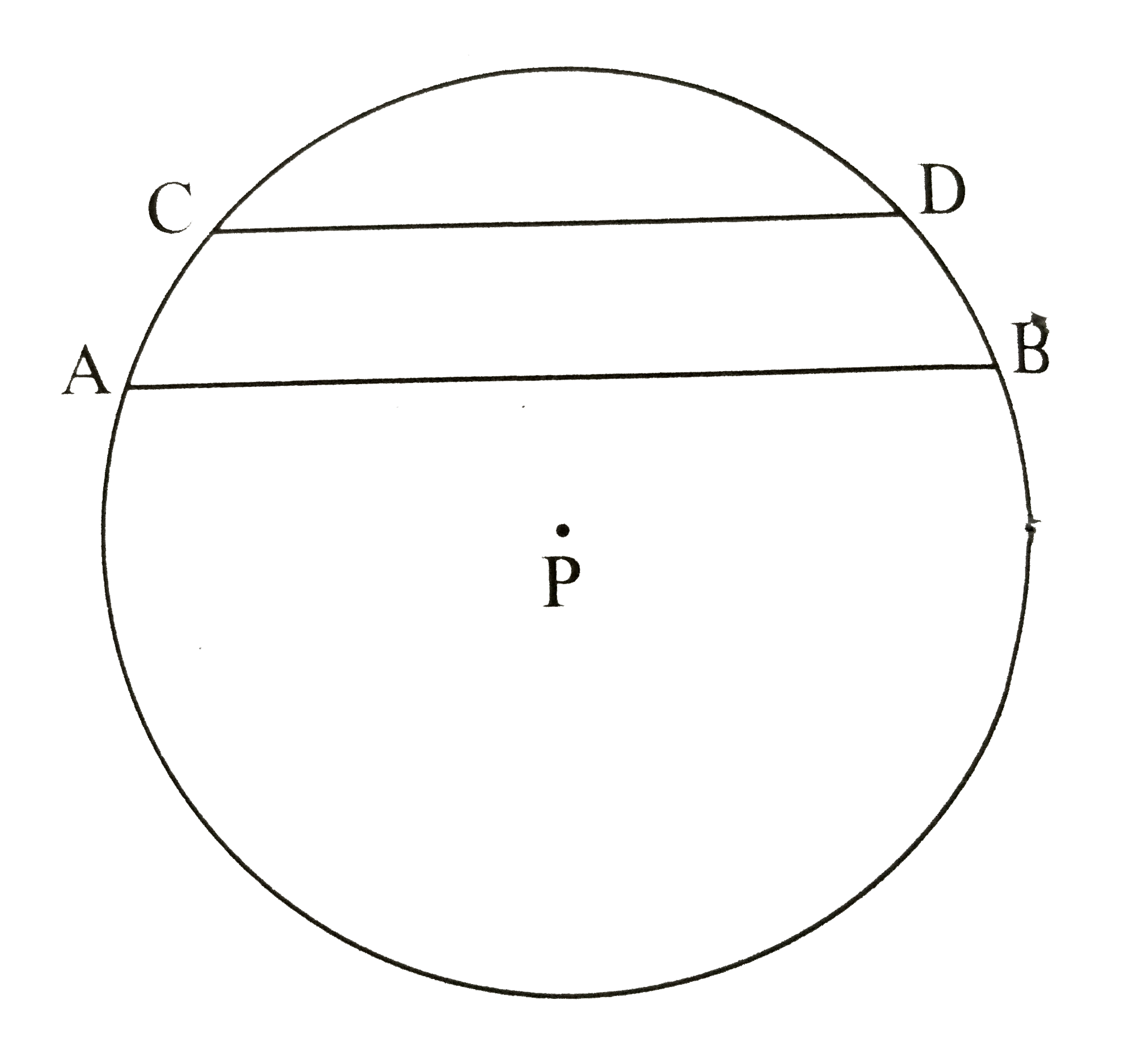
View SolutionIn the figure, two chords AB and CD are parallel to each other. P is the centre of the circle.
Show that ∠CPA≅∠DPB .
 View Solution
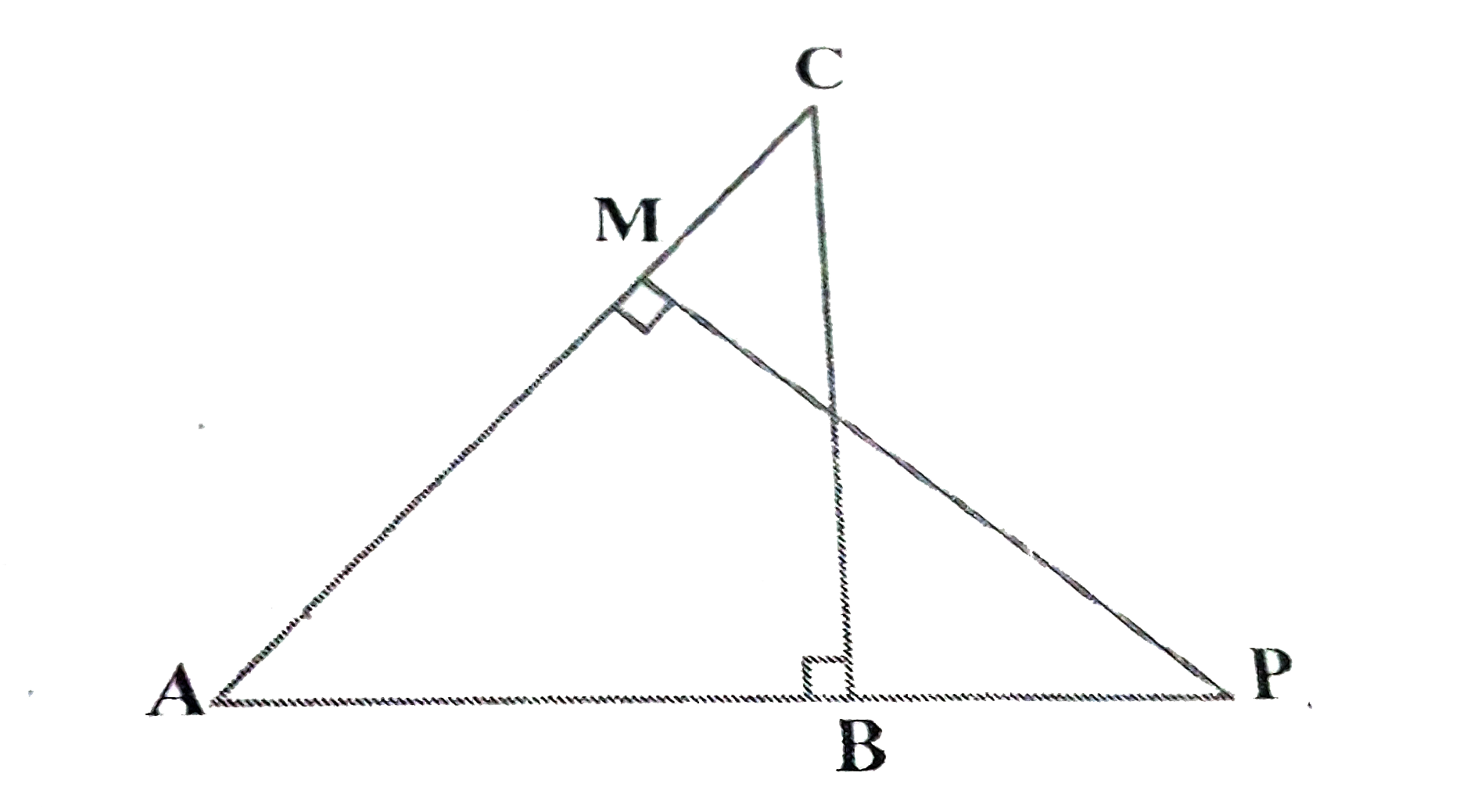
View SolutionIn figure ABC and AMP are two right triangles, right angles at B and M respectively. Prove that(i) ΔABC~ΔAMP (ii) CAPA=BCMP
 View Solution
View Solution