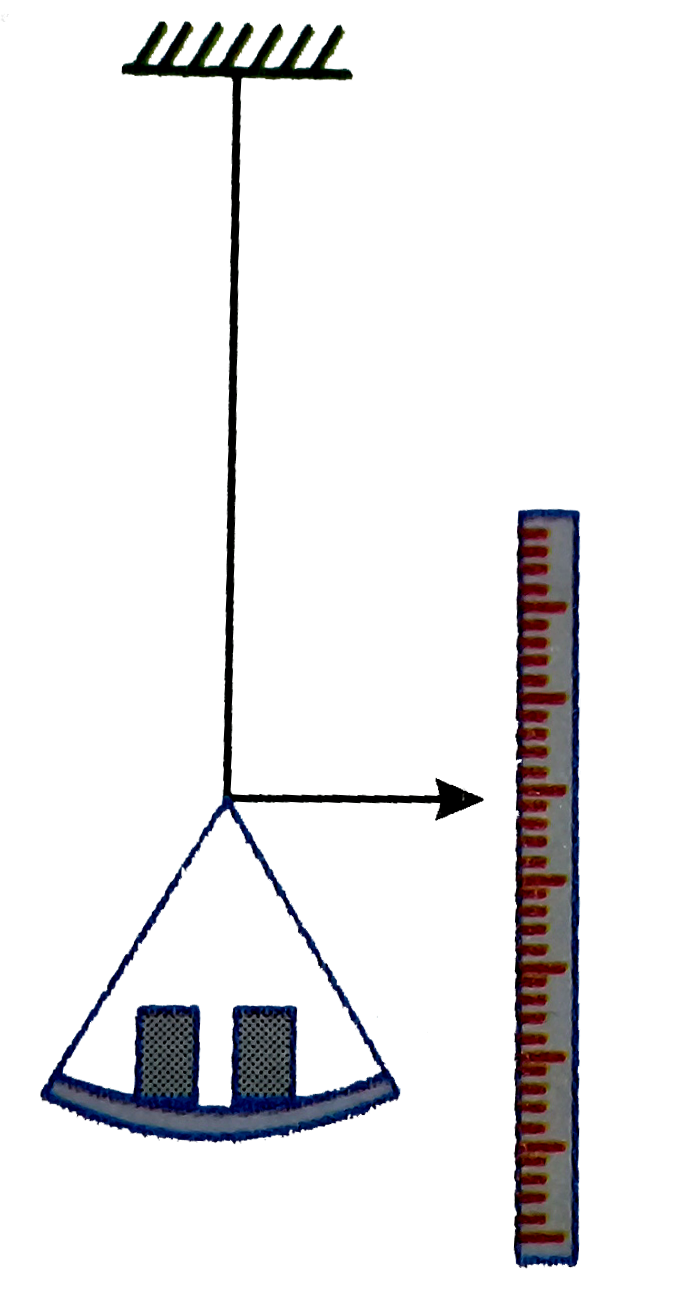
In the experiment to determine Young's modulus of the material of a wire under tension
used in the arrangement as shown. The percentage error in the measurement
of length is a in the measurement of the radius of the wire is b and in the
measurement of the change in length of the wire is c. Percentage error in the measurement
of Young's modulus for a given load is
A
a−2b+c
B
a−2b−c
C
a+2b+c
D
a+2b
Video Solution
Text Solution
The correct Answer is:C
Y=Fπr2×lΔl
Taking log of both the sides
logY=logF+logl−logπ−2logr−logΔl
Differentiating it, we have
ΔYY=0+Δll−0−2Δrr−ΔlΔl
% error, ΔYY×100=(Δll+2Δrr+Δ(Δl)Δl)×100
=a+2b+c
|
Topper's Solved these Questions
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Asseration - Reason Type Question
18 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance
18 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
Similar Questions
Knowledge Check
In the experiment to determine Young's modulus of the material of a wire under tension
used in the arrangement as shown. The percentage error in the measurement
of length is a in the measurement of the radius of the wire is b and in the
measurement of the change in length of the wire is c. Percentage error in the measurement
of Young's modulus for a given load is
 Aa−2b+cBa−2b−cCa+2b+cDa+2b
Aa−2b+cBa−2b−cCa+2b+cDa+2bThe Young's modulus of the material of a wire is equal ot the
Astress required to increase its length four timesBstress required to produce unit strainCstrain produced in itDhalf the strain produced in itAs temperture increases the Young's modulus of the material of a wire
AincreasesBdecreasesCremains the sameDbecomes infinite
The error in the measurement of the radius of a sphere is 1%. Find the error in the measurement of volume.
View SolutionGiven relative error in the measurement of length is .02, what is the percentage error ?
View SolutionError in the measurement of radius of a sphre is 1%. Then maximum percentage error in the measurement of volume is
View SolutionThe percentage error in the measurement of the voltage V is 3% and in the measurement of the current is 2%. The percentage error in the measurement of the resistance is
View SolutionThe maximum percentage error in the calculation of Young's modulus of the wire is
View Solution