In the given figure, ABCD is a quadrilateral in which AB = AD and BC = DC, Prove that
(i) AC bisects ∠Aand∠C, (ii) BE=DE,
(iii) ∠ABC=∠ADC.
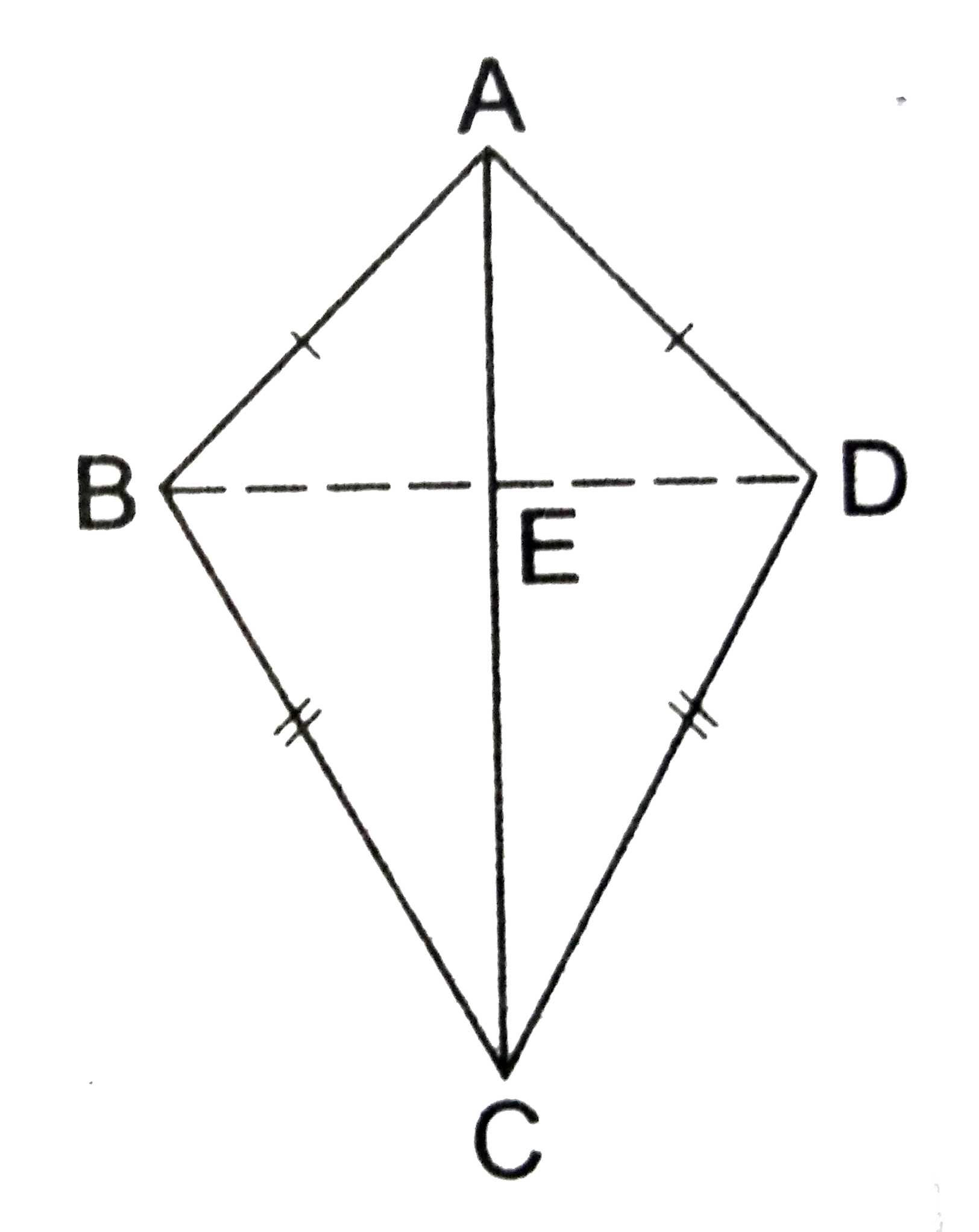
△ABC≅△ADC
⇒∠BAC=∠DAC,∠BCA=∠DCAand∠ABC=∠ADC.
△ADE≅△ADE⇒BE=DE.
Similar Questions
ABCD is a quadrilateral in which AB=BC and AD =CD ,Show that BD bisects boht the angle ABC and ADC
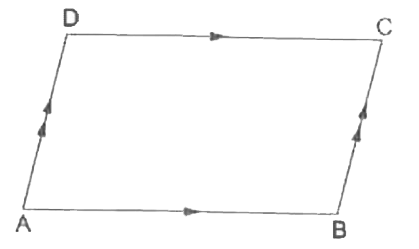
View SolutionABCD is a quadrilateral in which AB||DC and AD = BC. Prove that ∠A=∠Band∠C=∠D.
View SolutionIn the given figure ABCD is a trapezium with AB || Dc and AD = BC prove that
∠C=∠D

View SolutionIn the given figure ABCD is, ABCD is a quadrilateral in which AB∣∣DC and AD∣∣BC prove that ANGLEADC=ANGLEABC.
View SolutionIn quadrilateral ABCD, BA = BC and DA = DC. Prove that BD bisects ∠ ABC as well as ∠ ADC.
View SolutionIn the given figure, quadrilateral ABCD is a quadrilateral in which AB = AD and BC = DC. Prove that (i) AC bisects ∠A and ∠B, (ii) AC.is the perpendicular bisector of BD.
View SolutionIn the given figure ABCD is a trapezium with AB || Dc and AD = BC prove that
diagonal AC = diagonal BD

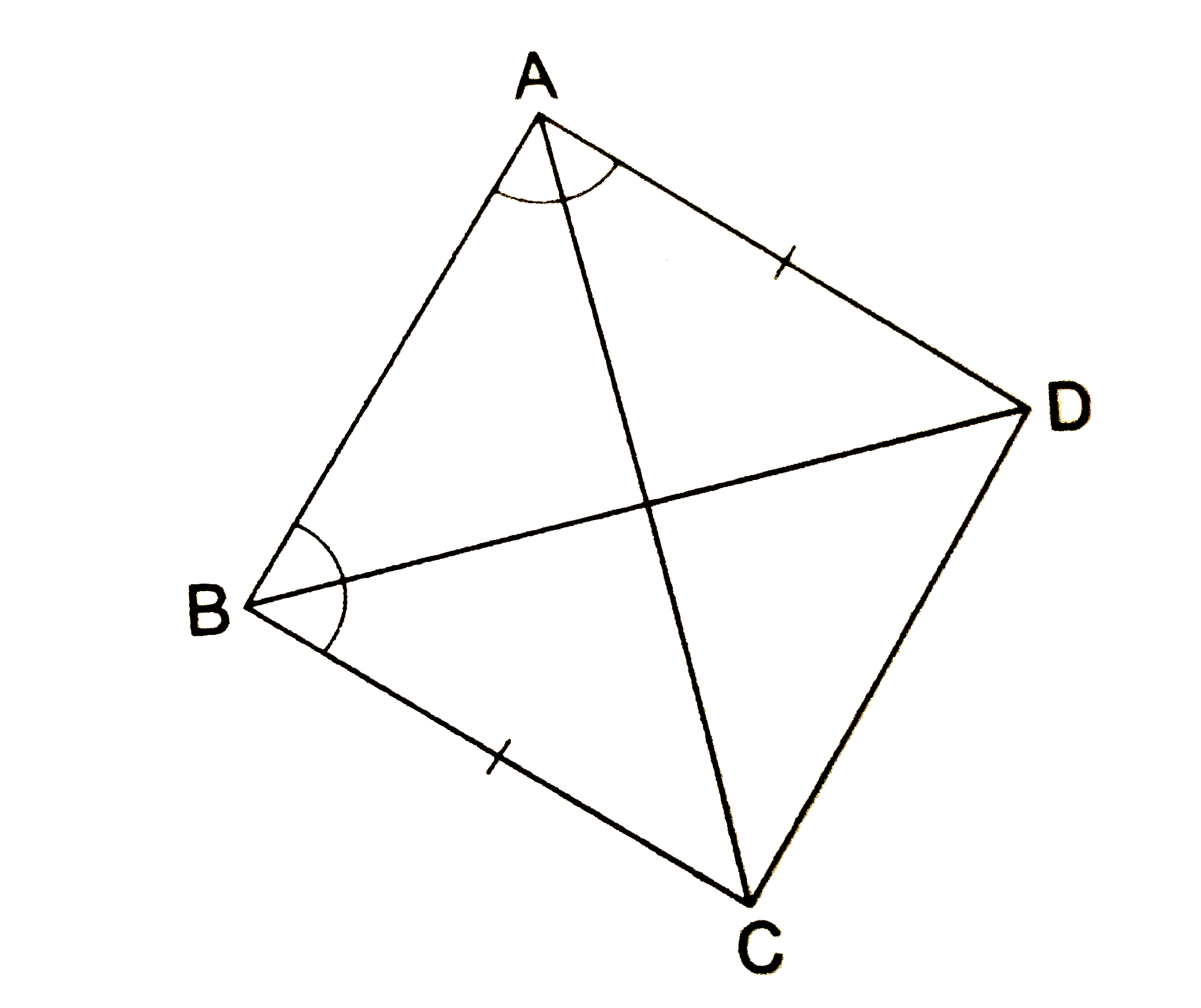
View SolutionIn the given figure, ABCD is a quadrilateral in which AD = BC and ∠DAB=∠CBA.
Prove that (i) ΔABD≅ΔBAC,
(ii) BD = AC,
(iii) ∠ABD=∠BAC.
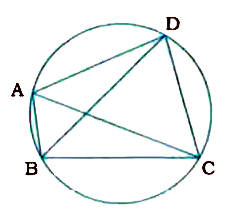
View SolutionIn the given figure ABCD is a cyclic quadrilateral in which AC and BD are its diagonals. If ∠DBC=55∘and∠BAC=45∘, find ∠BCD.
View SolutionIn the given figure, ABCD is a quadrilateral in which AB∣∣DC and P is the midpoint of BC. On producing, AP and DC meet at Q. Prove that (i) AB=CQ, (ii) DQ=DC+AB
View Solution
