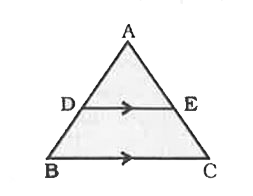
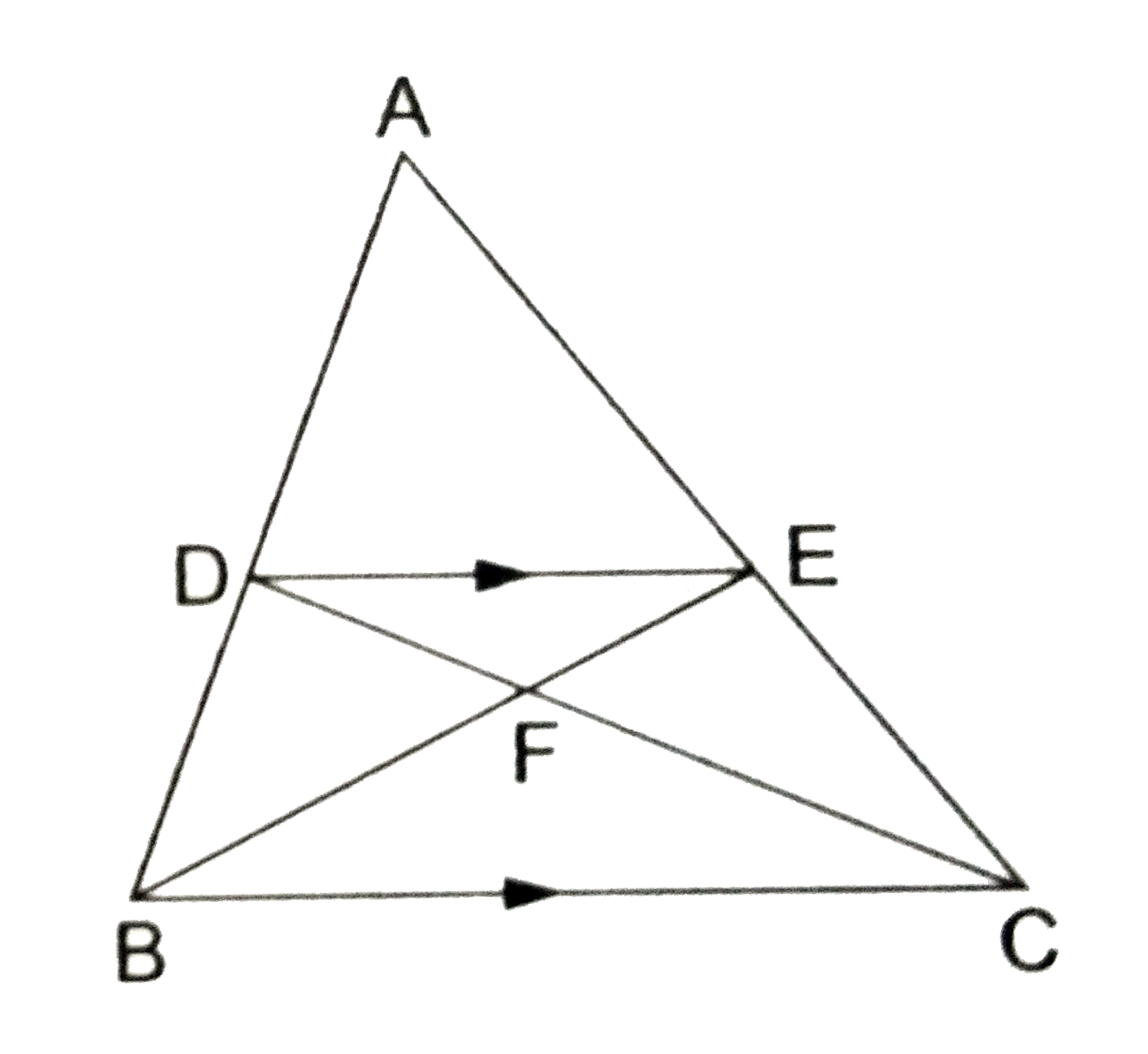
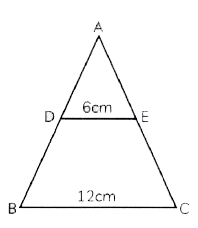
In the given figure DE∣∣BCandAD:DB=5:4
Find the ratio ar(ΔDEF):ar(ΔCFB)
Video Solution
Text Solution
Since DE||BC
In △ADEand△ABC
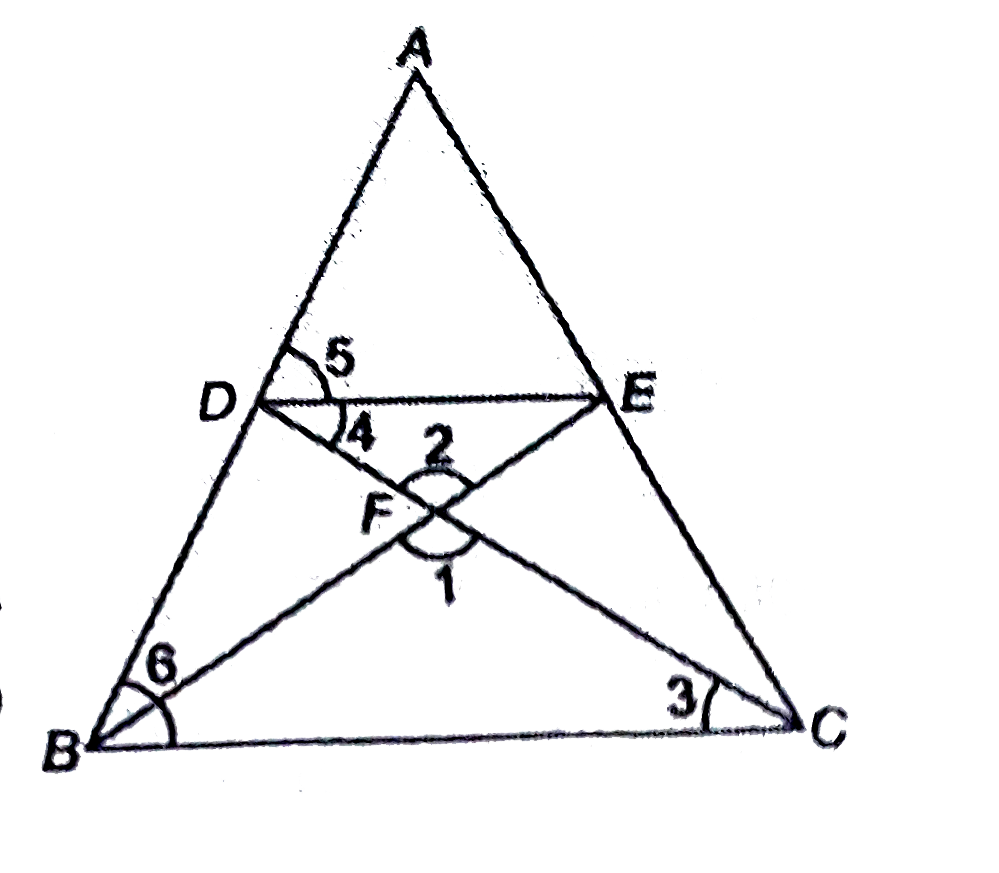
∠5=∠6 (corresponding angles)
∠aA=∠A (common) ,
△ADE~△ABC (AA corollary)
ADAB=)DEBC
⇒ ADAD+DB=DEBC⇒BCDE=AD+BDAD
⇒ BCDE=1+BDAD⇒BCDE=1+45 (ADBD=54, given )
BCDE=95
DEBC=59 ...(1)
In △DEFand△CFB
∠4=∠3 ( alternate angles as DE||BC)
and ∠2=∠1 ( vertically opposite angles)
△DEF~△CBF (AA corollary)
ar(△DEF)ar(△CBF)=DE2BC2=(59)2 (area ratio theorem)
Hence , ar(△DEF)ar(△CBF)=2581
|
Topper's Solved these Questions
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Problems From NCERT/ Exemplar
14 Videos
- TRIANGLES BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Exercise 6a
23 Videos
- STATISTICS BOOK - NAGEEN PRAKASHANCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions
5 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBOOK - NAGEEN PRAKASHANCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions
5 Videos
Similar Questions
Knowledge Check
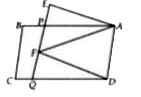
In the given figure, ABCD and AEFD a re two parallelograms.
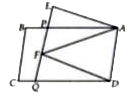
(ar(ΔAPE))/(ar(ΔPFA)) =A(ar(ΔQFD))/(ar(ΔPFD))B(ar(ΔAEF))/(ar(ΔPFD))C(ar(ΔQFD))/(ar(ΔAEF))DNone of theseIn the given figure, ABCD and AEFD a re two parallelograms.
ar(ΔPEA) =Aar(∣∣gmΔPEA)Bar(ΔPFD)Car(ΔQFD)Dar(∣∣gmΔCQPB)In figure, if DE||BC, then find the ratio of ar (ΔADE) and ar(DECB).
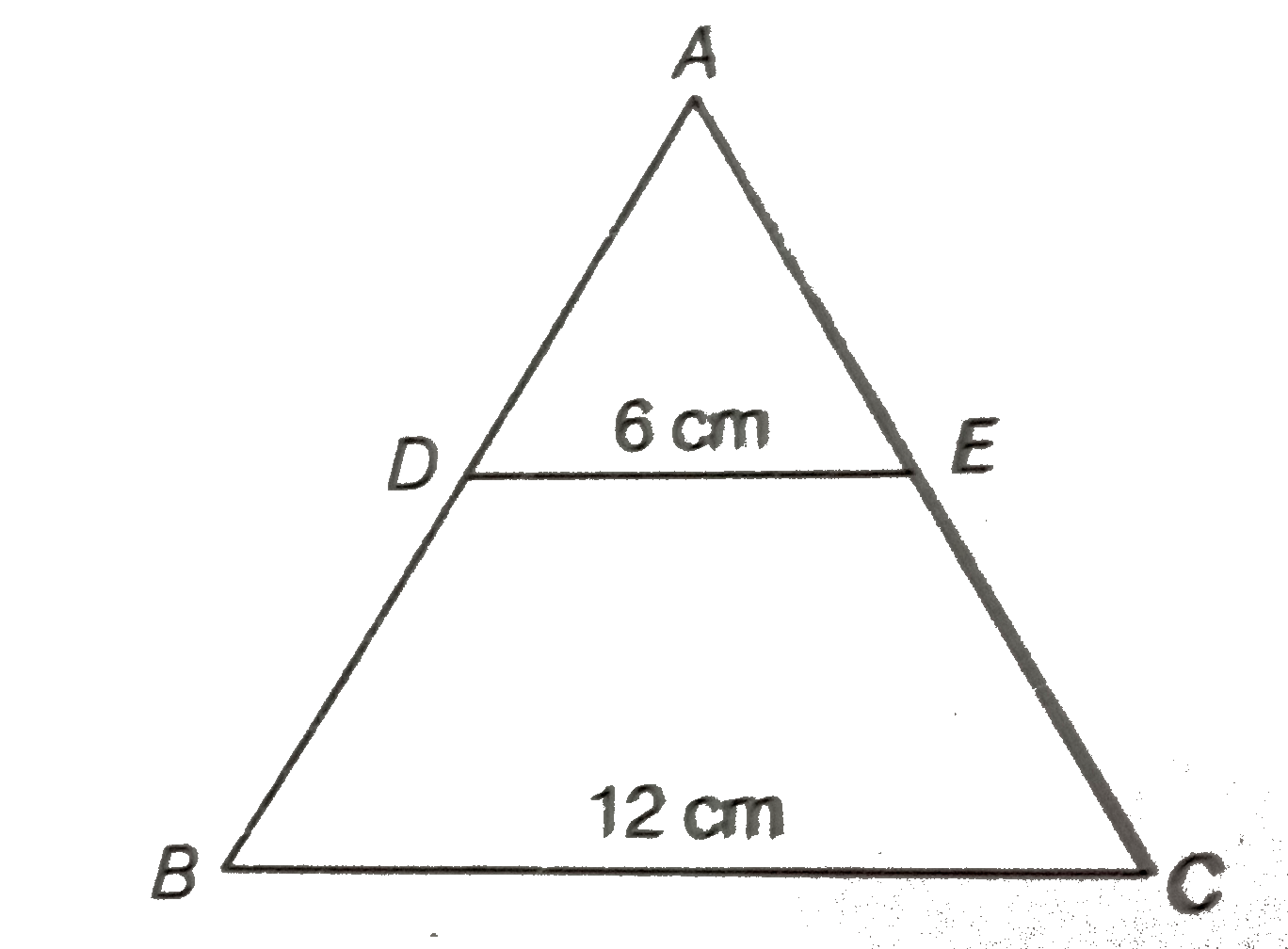 A1:3B1:2C2:5D3:5
A1:3B1:2C2:5D3:5
In the given figure, DE∣∣BCandDE:BC=3:5. Calculate the ratio of areas of ΔADE and the trpezium BCED.
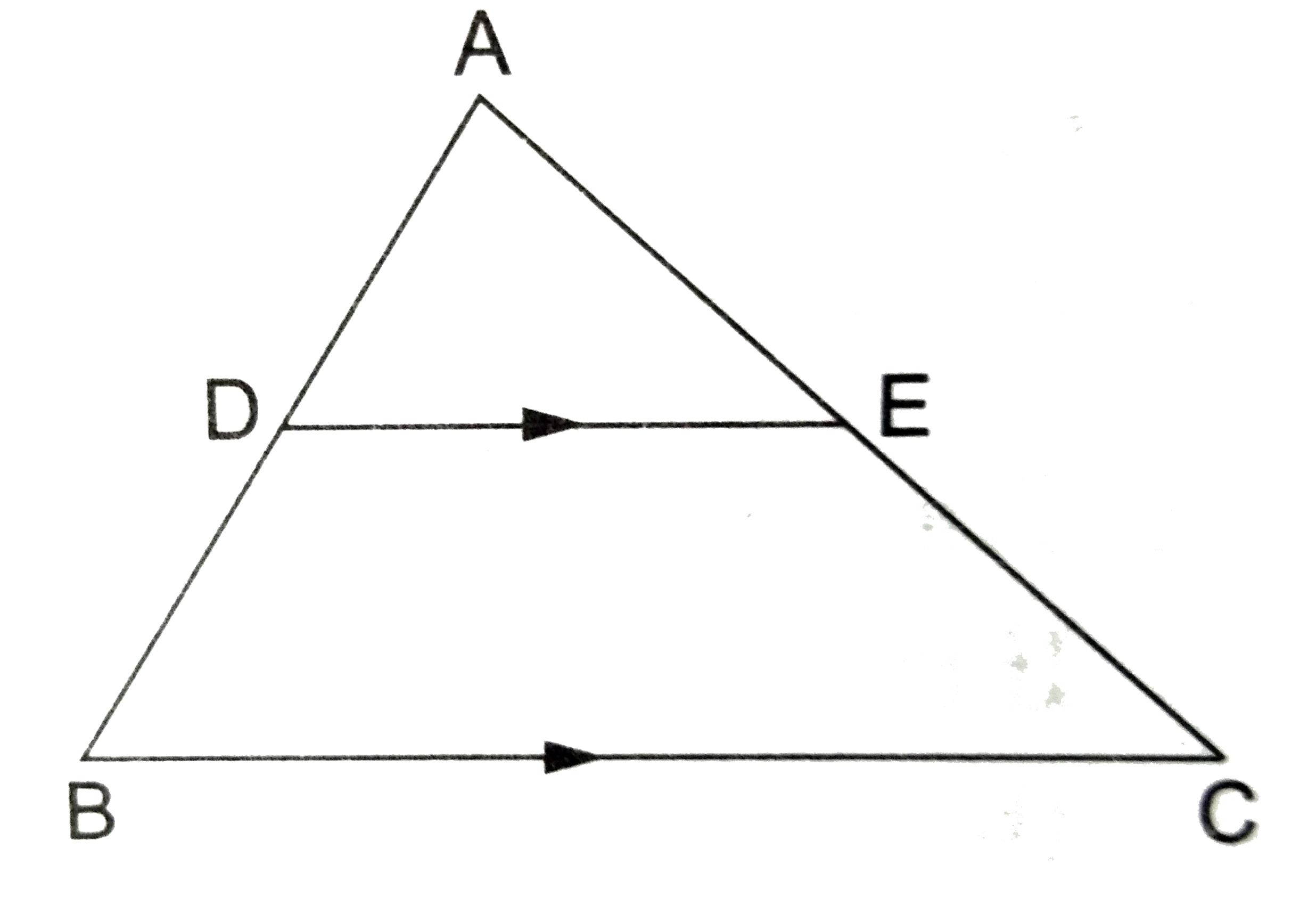
View SolutionIn a triangle ABC , D & E are two points on sides AB and AC such that DE∣∣BC and AD:DB=3:5. Find the ratio of area of ΔADE to the area of trapezium BDEC.
View SolutionIn the given figure, if DE∣∣BC, then find the ratio of area of (△ADE) and (□DECB).
View SolutionIn the figure, ABCD, DCFE and ABFE are parallelograms. Show that ar(ΔADE) = ar(ΔBCF).
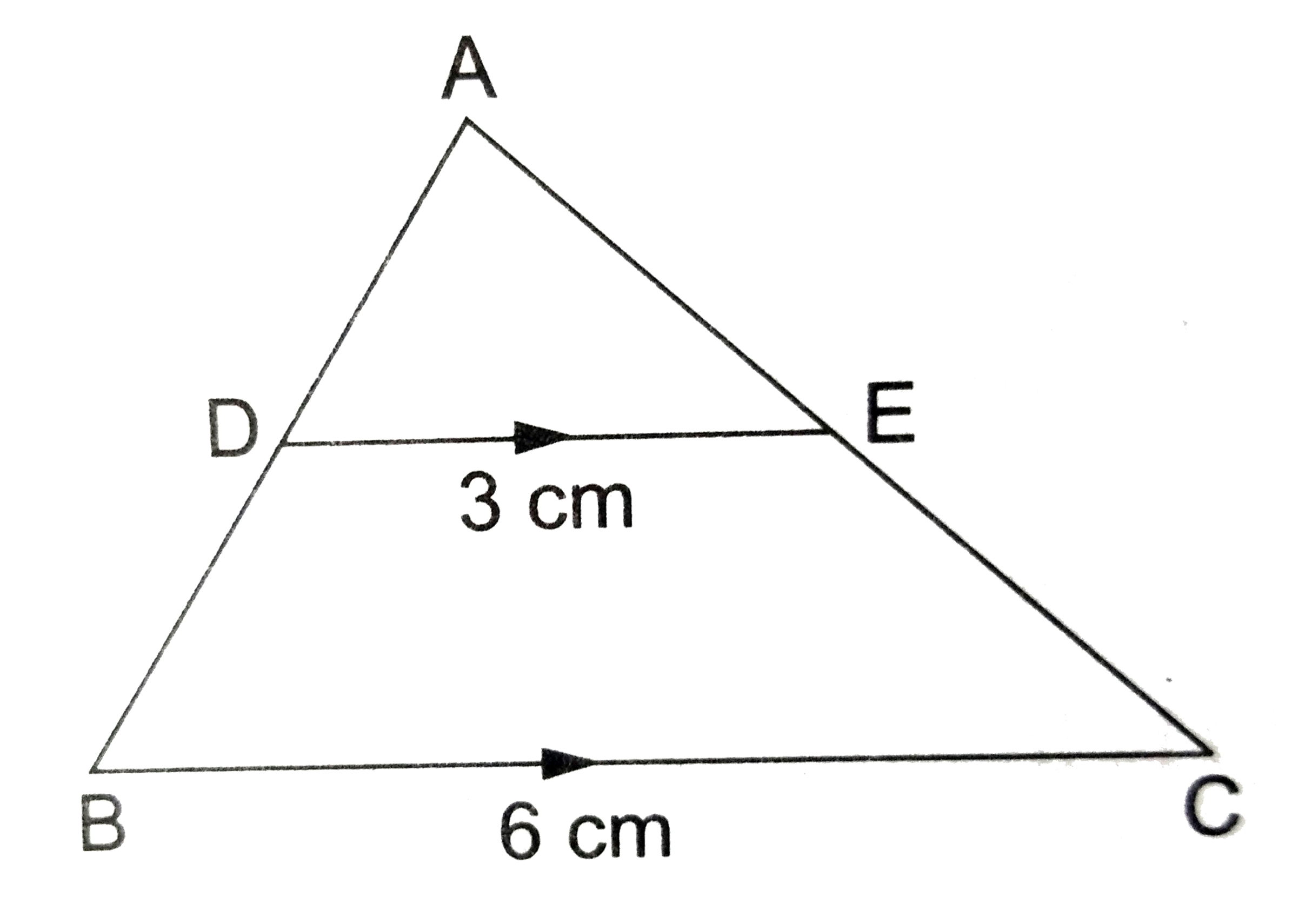
View SolutionIn the given figure, DE∣∣BC. If DE=3cm,BC=6andar(ΔADE)=15cm2, find the area of ΔABC.
View Solution