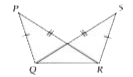
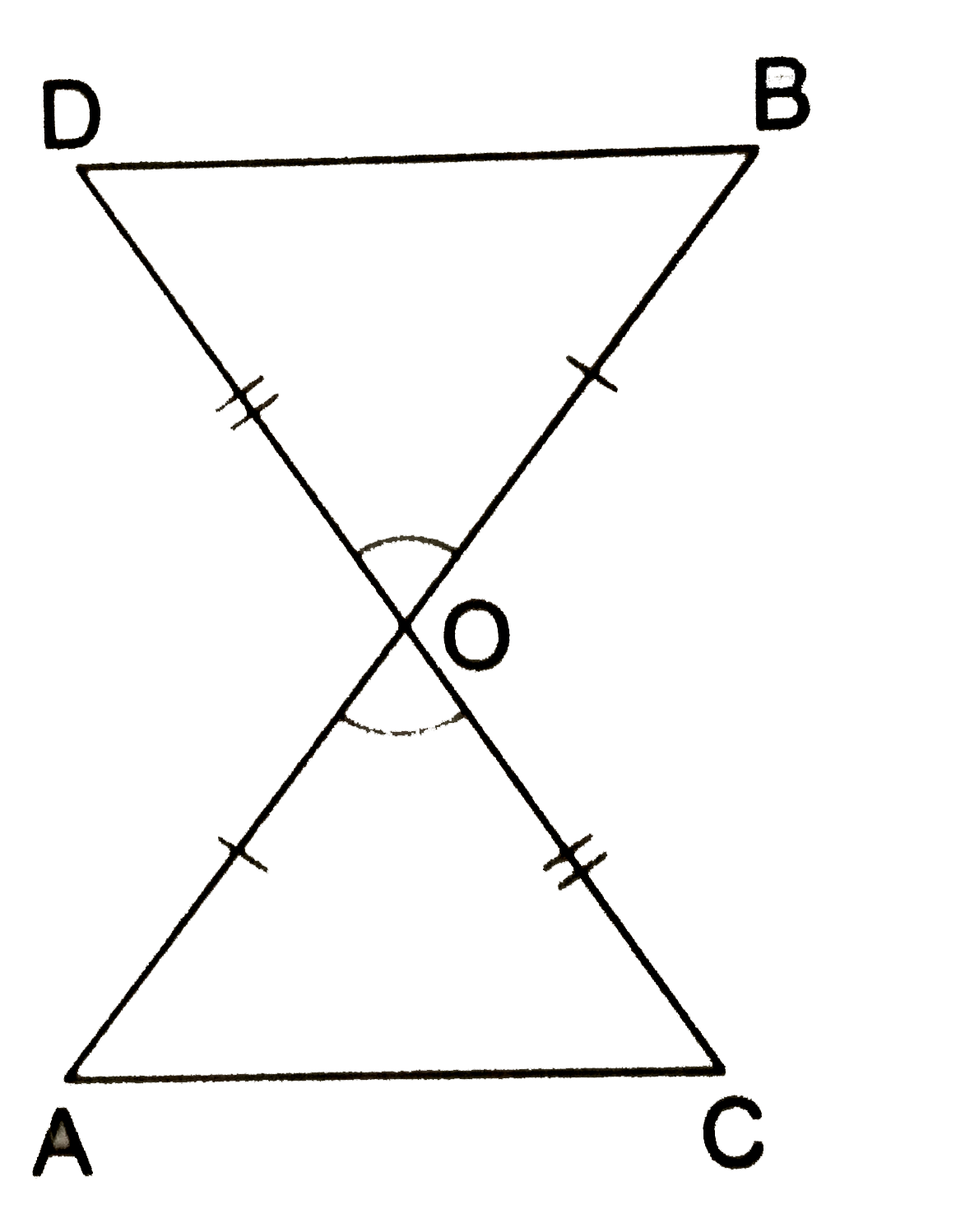
In the given figure, we have AO = BO and CO = DO. Prove that ΔAOC≅ΔBOD.

Step by step video & image solution for In the given figure, we have AO = BO and CO = DO. Prove that DeltaAOC~=DeltaBOD. by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CONGRUENCE OF TRIANGLES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - CONGRUENCE OF TRIANGLES EXERCISE - Subjective Problems (Short Answer Type)
12 Videos
- CONGRUENCE OF TRIANGLES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - CONGRUENCE OF TRIANGLES EXERCISE - Subjective Problems (Long Answer Type)
10 Videos
- CONGRUENCE OF TRIANGLES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - CONGRUENCE OF TRIANGLES EXERCISE - Comprehension Type
6 Videos
- COMPARING QUANTITIES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - COMPARING QUANTITIES EXERCISE - Olympaid/HOTS Corner
20 Videos
- DATA HANDLINGBOOK - MTG IIT JEE FOUNDATIONCHAPTER - DATA HANDLINGEXERCISE - Olympiad / Hots Corner
20 Videos
Similar Questions
Knowledge Check
In the given figure, If AB and CD bisect each other at O, then ΔAOC is congruent to
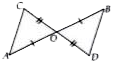 AΔBODBΔDOBCΔDOBDΔBDO
AΔBODBΔDOBCΔDOBDΔBDO
In the given figure, we have PQ = SR and Pr = SQ. Prove that:
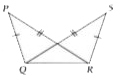
∠PQR=∠SRQ
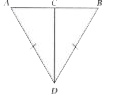
View SolutionIn the given figure, we have C is the mid-point of AB and DA = DB.
Prove that : ∠DCA=∠DCB.
View SolutionIn the given figure, AB=AC. prove that :
View SolutionIn the given figure, Oa = OB and OC = OD. Which is the criteria to prove
ΔAOC≅ΔBOD.
View SolutionIn the given figure we have ∠1=∠3and∠2=∠4 show that ∠A=∠C
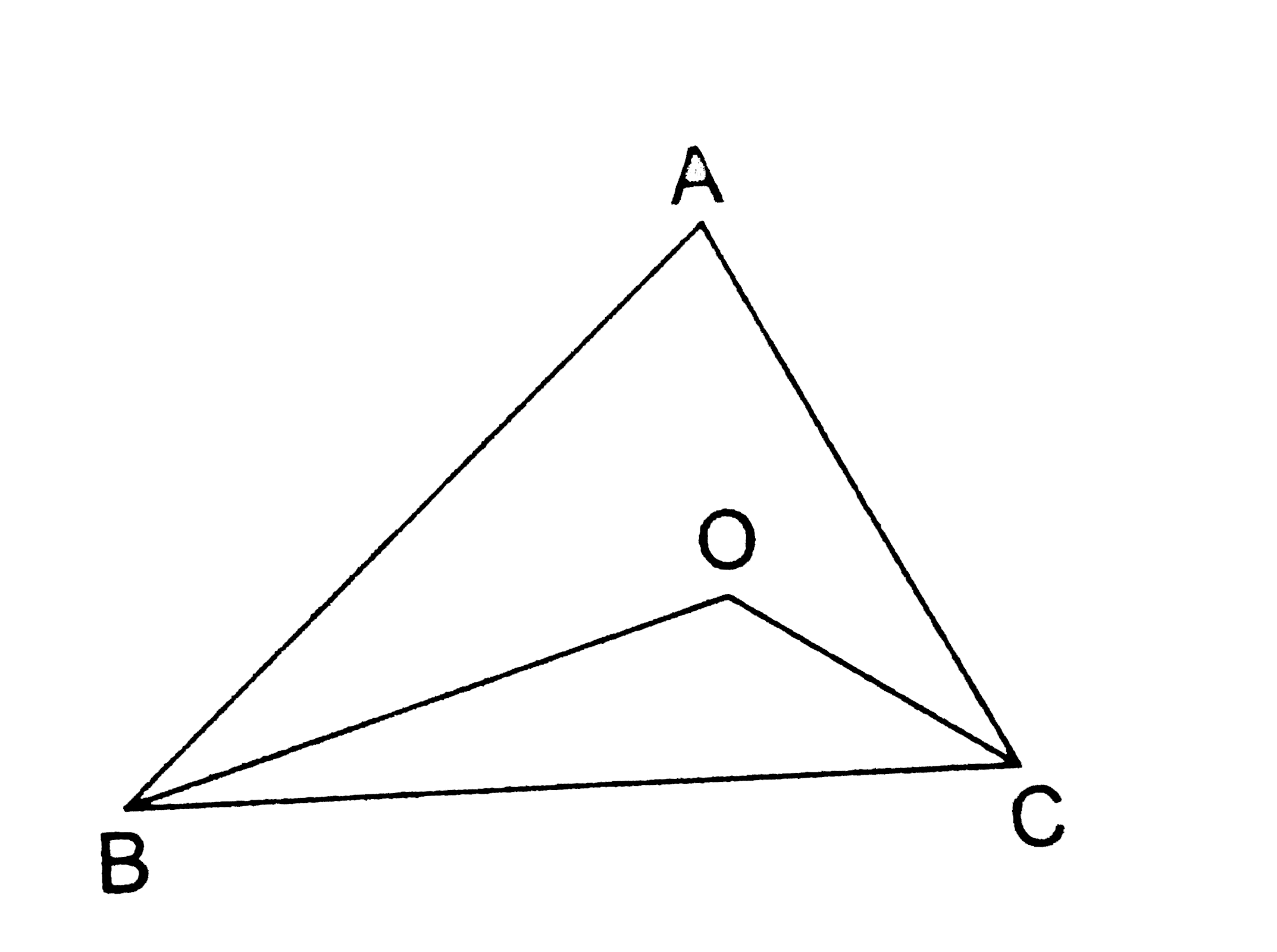
View SolutionIn the given figure, AB>AC. If BO and CO are the bisectors of ∠Band∠C respectively then
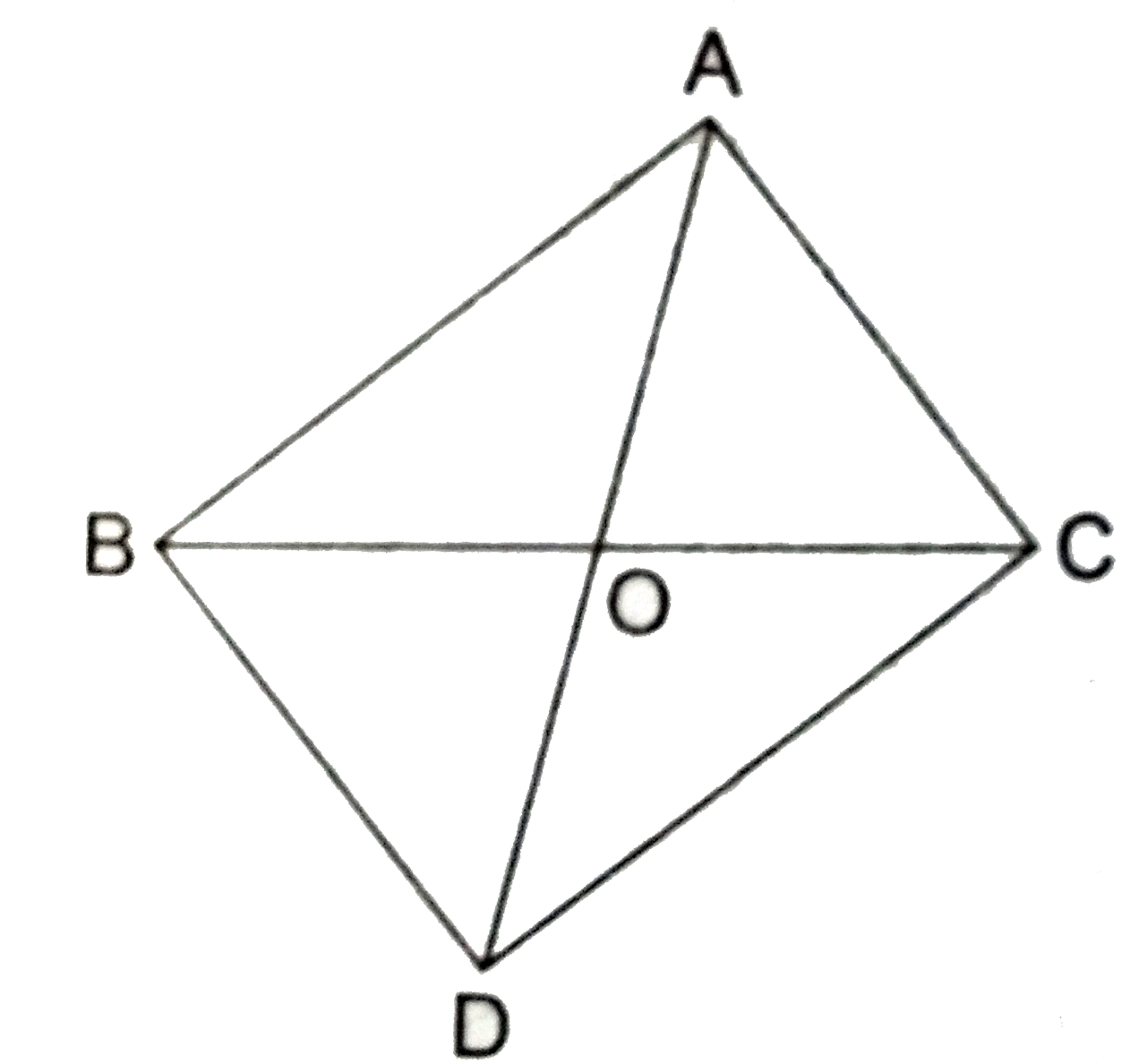
View SolutionIn the given figure, ΔABCandΔDBC have the same base BC. If AD and BC intersect at O, prove that ar(ΔABC)ar(ΔDBC)=AODO
View Solution