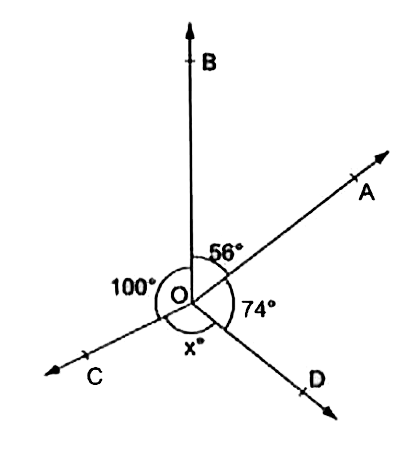
In the given figure,rays OA,OB,OC and OD are such that ∠AOB=56∘,∠BOC=100∘,∠COD=x∘ and ∠DOA=74∘,Find the value of x.
A
x=90∘
B
x=130∘
C
x=120∘
D
x=110∘
Video Solution
Text Solution
The correct Answer is:B
|
Answer
Step by step video & image solution for In the given figure,rays OA, OB, OC and OD are such that angleAOB=56^(@),angleBOC=100^(@),angleCOD=x^(@) and angleDOA=74^(@),Find the value of x. by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LINES AND ANGLESBOOK - RS AGGARWALCHAPTER - LINES AND ANGLESEXERCISE - Exercise 13
11 Videos
- LINEAR EQUATION IN ONE VARIABLEBOOK - RS AGGARWALCHAPTER - LINEAR EQUATION IN ONE VARIABLEEXERCISE - TEST PAPER 7D
1 Videos
- MENSURATIONBOOK - RS AGGARWALCHAPTER - MENSURATIONEXERCISE - TEST PAPER (True/False)
4 Videos
Similar Questions
Knowledge Check
In the given figure, rays OA, OB, OC and OD are such that ∠AOB = 50∘ , ∠BOC = 90∘, ∠COD=70∘ and ∠AOD=x∘. Then, the value of x is
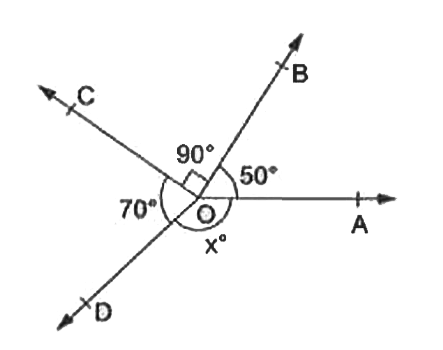 A50∘B70∘C150∘D90∘
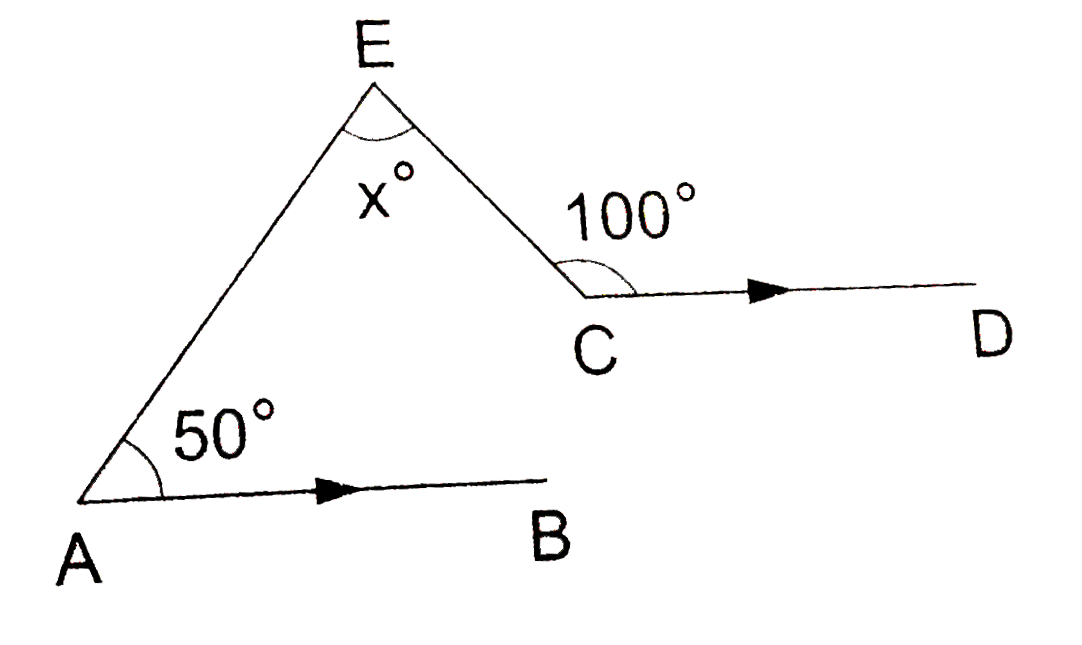
A50∘B70∘C150∘D90∘In the given figure, AB∣∣CD,∠ABE=120∘,∠ECD=100∘ and ∠BEC=x∘. Find the value of x.
 Ax=30Bx=20Cx=50Dx=40
Ax=30Bx=20Cx=50Dx=40In the given figure, find the value of ∠BOC
 A101∘B149∘C71∘D140∘
A101∘B149∘C71∘D140∘
In the figure.AOB is a straight line and rays OC and OD stand on it.
∠AOC=65∘,∠BOD=70∘and∠COD=x∘,find the value of x.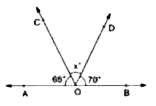
View SolutionIn the given figure, find the value of x.
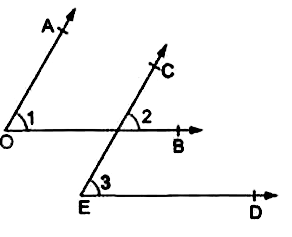
View SolutionIn the adjoining Figure it is given that OA∣∣EC and OB∣∣ED prove that ∠AOB=∠CED
View SolutionIn the given figure, AOB is a straight line. If ∠AOC=(3x+10)∘ and ∠BOC=(4x−26)∘, then ∠BOC= ?

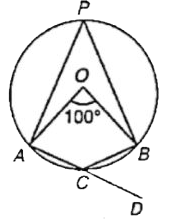
View SolutionIn the given figure, O is the centre of circle, ∠AOB=100∘. Find ∠BCD :
View Solution