Let A(−4,0) and B(4,0). Then the number of points C=(x,y) on the circle x2+y2=16 lying in first quadrant such that the area of the triangle whose vertices are A,B and C is a integer is
The correct Answer is:62
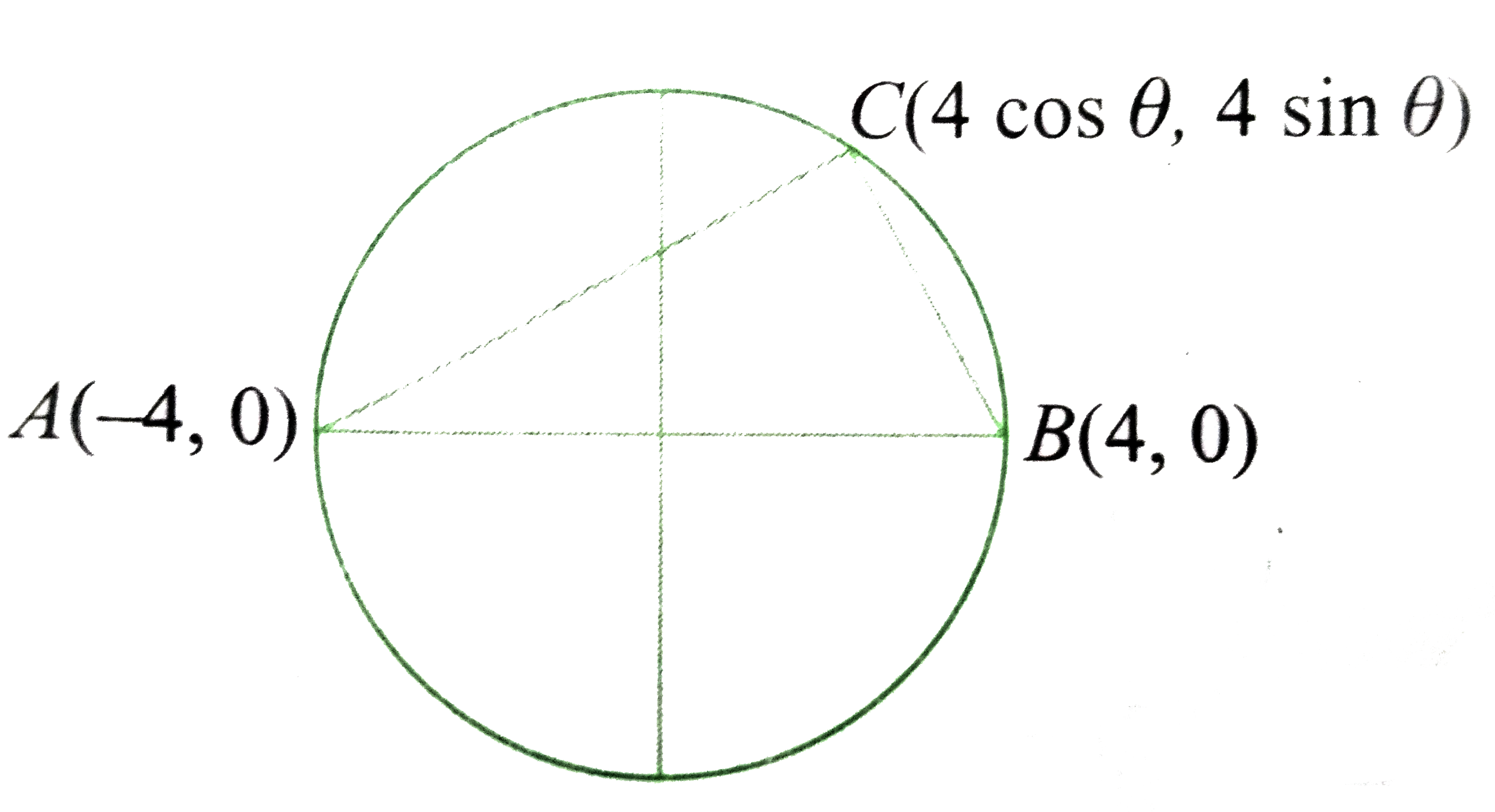
Required area
A=12×8×|4sinθ|=|16sinθ|
Now, the area is an integer. Then the possible values of sinθ are
116,216,.....,1516
i.e., 15 points in each quadrant or 60+2 more with sinθ=1.
Therefore,N=62 lt
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - ARCHIVES (JEE MAIN)1videos
CIRCLE
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - Single Correct Answer Type9videos
CIRCLE
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - For Problems43videos
CIRCLE
- BOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix4videos
BINOMIAL THEOREM
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Comprehension Type8videos
CIRCLES
Similar Questions
Let A(−4,0), B(4,0) & C(x,y) be points on the circle x2+y2=16 such that the number of points for which the area of the triangle whose vertices are A, B and C is a positive integer, is N then the value of [N7] is, where [⋅] represents greatest integer function.
View SolutionFind the vertices and the area of the triangle whose sides are x=y,y=2xandy=3x+4.
View SolutionLet A(−4,0),B(4,0) Number of points c=(x,y) on circle x2+y2=16 such that area of triangle whose verties are A,B,C is positive integer is:
View SolutionThe area of the region cut off by the line x−y=0, X-axis from the circle x2+y2=16, in first quadrant is
View SolutionNumber of points of intersectionsof circle x2+y2+2x=0
with y2=4x isView SolutionFind the vertices of the triangle whose sides are y+2x=3,4y+x=5and5y+3x=0
View SolutionThe area of the triangle whose sides y=m1x+c1,y=m2x+c2andx=0 is
View SolutionA planet of mass m moves along an ellipse around the sun (mass M) so that its maxima and minimum distances from the sun are equal to r1 and r2 respectively. The angular momentum of this plane relative to the centre of the sun is m√PGMr1r28(r1+r2)
View SolutionArea lying in the first quadrant and bounded by the circle x2+y2=4 and the lines x=0 and x=2 is:
View SolutionFor the circle x2+y2−4x+2y+c=0 radius is 4 then c=
View Solution

