Let A be a set containing elements. A subset P of the set A is chosen at random. The set A is reconstructed by replacing the elements of P, and another subset Q of A is chosen at random. The probability that P∩Q contains exactly m(m<n) elements, isSolution in Bengali
3n−m4n
.nCm.3m4n
.nCm.3n−m4n
none of these
The correct Answer is:C
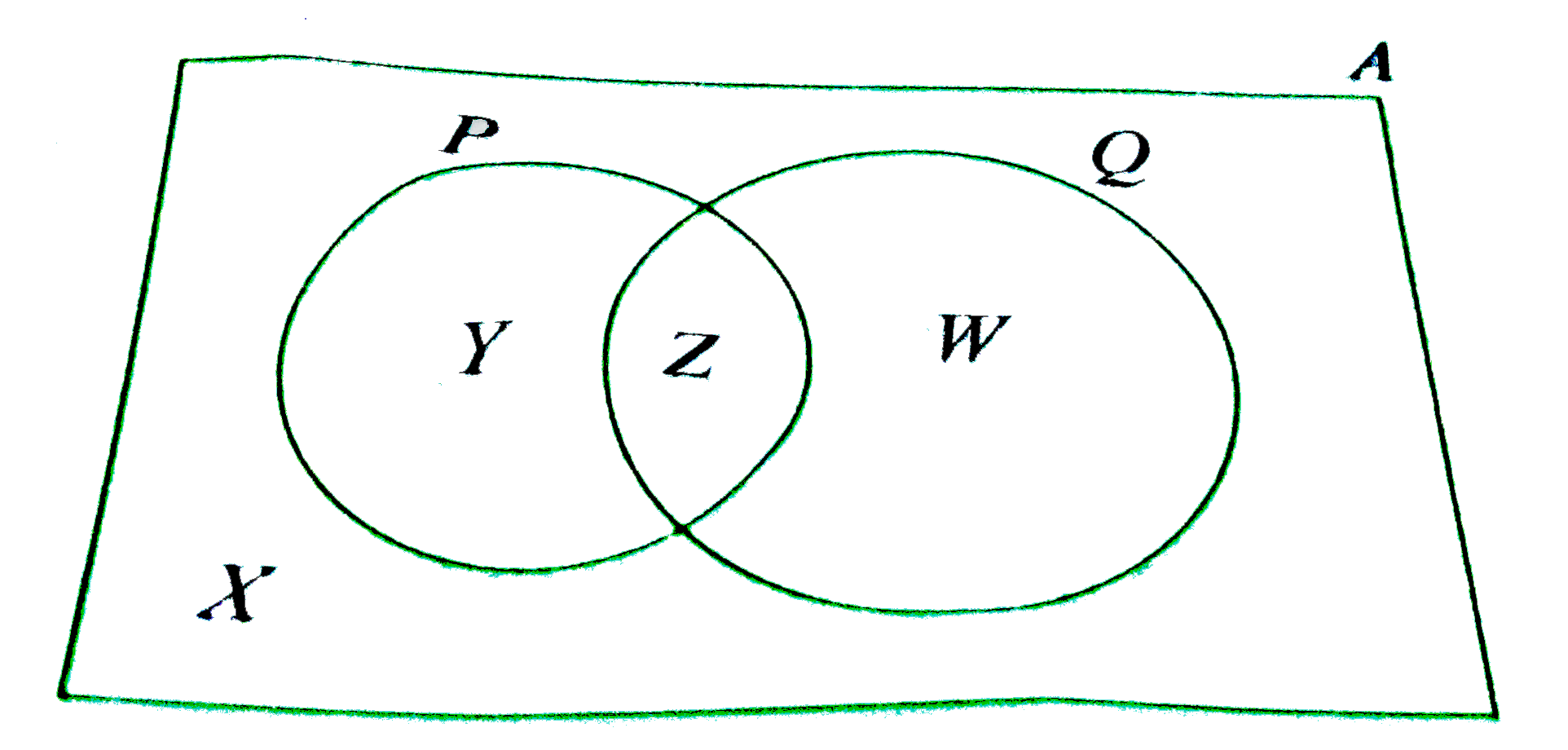
Sets P and Q are subsets of set A. Sets P and Q are dividing set A in four regions X, Y, Z and W.
∴ Total number of cases
= Number of ways in which n elements can be distributed in four regions = 4n
If set (P∩Q) has exactly m elements, we can select these m elements in .nCm ways. Remaining n - m elements can be distributed in the regions X, Y and W in 3n−m ways.
∴ Number of favourable ways = .nCm×3n−m
∴ Required probability = .nCm×3n−m4n
Topper's Solved these Questions
- PROBABILITY I BOOK - CENGAGE PUBLICATIONCHAPTER - PROBABILITY I EXERCISE - Comprehension
14 Videos
- PROBABILITY I BOOK - CENGAGE PUBLICATIONCHAPTER - PROBABILITY I EXERCISE - Match
2 Videos
- PROBABILITY I BOOK - CENGAGE PUBLICATIONCHAPTER - PROBABILITY I EXERCISE - Exercise 9.3
7 Videos
- PROBABILITYBOOK - CENGAGE PUBLICATIONCHAPTER - PROBABILITYEXERCISE - All Questions
470 Videos
- PROBABILITY IIBOOK - CENGAGE PUBLICATIONCHAPTER - PROBABILITY IIEXERCISE - MULTIPLE CORRECT ANSWER TYPE
6 Videos
Similar Questions
A shopping mall is running a scheme: Each packet of detergent SURF contains a coupon which bears letter of the word SURF, if a person buys at least four packets of detergent SURF, and produce all the letters of the word SURF, then he gets one free packet of detergent.
If a person buys 8 such packets, then the probability that he gets two free packets is
View Solutionn whole are randomly chosen and multiplied
Column I, Column II
The probability that the last digit is 1,3,7, or 9 is, p. 8n−4n10n
The probability that the last digit is 2,4,6, or 8 is, q. 5n−4n10n
The probability that the last digit is 5 is, r. 4n10n
The probability that the last digit is zero is, s. 10n−8n−5n+4n10n
View SolutionAandB are two candidates seeking admission in IIT. The probability that A is selected is 0.5 and the probability that AandB are selected is at most 0.3. Is it possible that the probability of B getting selected is 0.9?
View SolutionA coin is tossed three times, consider the following events. A : ‘No head appears’, B: ‘Exactly one head appears’ and C: ‘Atleast two appear’. Do they form a set of mutually exclusive and exhaustive events?
View SolutionLet EandF be two independent events. The probability that exactly one of them occurs is 1125 and the probability if none of them occurring is 225. If P(T) denotes the probability of occurrence of the event T, then (a) P(E)=45,P(F)=35 (b) P(E)=15,P(F)=25 (c) P(E)=25,P(F)=15 (d) P(E)=35,P(F)=45
View SolutionA is a set containing n elements. A subset P of A is chosen at random. The set A is reconstructed by replacing the elements of P. A subset Q is again chosen at random. The probability that P and Q are disjoint sets, is
View SolutionA is a set containing n elements. A subset P of A is chosen at random. The set A is reconstructed by replacing the elements of P. A subset Q is again chosen at random. The Probability that P∪Q=A, is
View SolutionA is a set containing n elements. A subset P of A is chosen at random. The set A is reconstructed by replacing the elements of P. A subset Q is again chosen at random. The Probability that P∩Q contain just one element, is
View SolutionA is a set containing n elements. A subset P of A is chosen at random. The set A is reconstructed by replacing the elements of P. A subset Q is again chosen at random. The Probability that P∪Q contain just one element, is
View SolutionA is a set containing n elements. A subset P of A is chosen at random. The set A is reconstructed by replacing the elements of P. A subset Q is again chosen at random. The Probability that Q is a subset of P, is
View SolutionA is a set containing n elements. A subset P of A is chosen at random. The set A is reconstructed by replacing the elements of P. A subset Q is again chosen at random. The Probability that P and Q have equal number of elements, is
View SolutionA is a set containing n elements. A subset P of A is chosen at random. The set A is reconstructed by replacing the elements of P. A subset Q is again chosen at random. The probability that Q contains just one element more than P, is
View SolutionLet A be a set containing elements. A subset P of the set A is chosen at random. The set A is reconstructed by replacing the elements of P, and another subset Q of A is chosen at random. The probability that P∩Q contains exactly m(m<n) elements, is
View SolutionLet A be a set containing elements. A subset P of the set A is chosen at random. The set A is reconstructed by replacing the elements of P, and another subset Q of A is chosen at random. The probability that P∩Q contains exactly m(m<n) elements, is
View SolutionA is a set containing n elements. A subset P of A is chosen at random. The set A is reconstructed by replacing the elements of P. A subset Q of A is again chosen at random. Find the probability that
P∪Q=A
View SolutionA is a set containing n elements. A subset P of A is chosen at random. The set A is reconstructed by replacing the elements of P. A subset Q of A is again chosen at random. Find the probability that
P∪Q contains exactly r elements
View Solution
