Let A={x−1≤x≤1}andf:A→A such that f(x)=x|x| then f is:
A
injective but not surjective
B
surjective but not injective
C
bijective
D
None of these
Video Solution
Text Solution
The correct Answer is:C
We have
f(x)=x|x|{x2x≥0−x2x<0
The graph, f(x) is shown in Fig. 10
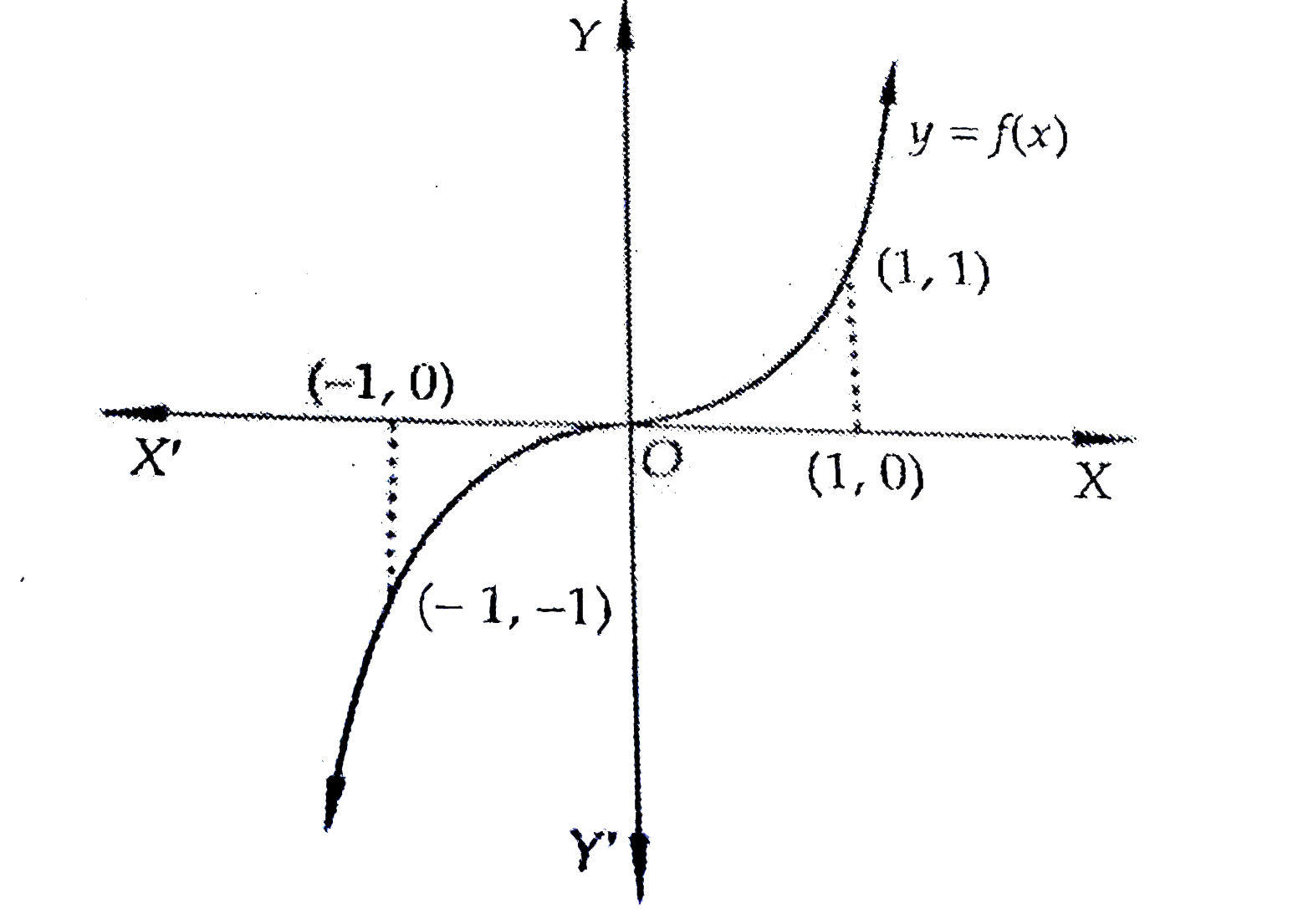
Clearly, f is a bijective
|
Topper's Solved these Questions
- FUNCTIONSBOOK - OBJECTIVE RD SHARMACHAPTER - FUNCTIONSEXERCISE - Section II - Assertion Reason Type
10 Videos
- FUNCTIONSBOOK - OBJECTIVE RD SHARMACHAPTER - FUNCTIONSEXERCISE - Exercise
48 Videos
- FUNCTIONSBOOK - OBJECTIVE RD SHARMACHAPTER - FUNCTIONSEXERCISE - Chapter Test
30 Videos
- DISCRETE PROBABILITY DISTRIBUTIONSBOOK - OBJECTIVE RD SHARMACHAPTER - DISCRETE PROBABILITY DISTRIBUTIONSEXERCISE - Exercise
40 Videos
- HYPERBOLABOOK - OBJECTIVE RD SHARMACHAPTER - HYPERBOLAEXERCISE - Chapter Test
29 Videos
Similar Questions
Knowledge Check
Let A={−1≤x≤1}andf:A→A such that f(x)=x|x| then f is:
Aa bijectionBinjective but not surjectiveCsurjective but not injectiveDneither injective nor surjectiveLet f(x)={−1−2≤x<0x2−10≤x≤2 and g(x)=|f(x)|+f(|x|). Then , in the interval (-2,2),g is
Anot differentiable at one pointBnot differentiable at two pointsCdifferentiable at all pointsDnot continuousLet f(x) be defined by
f(x)={sin2xif 0<x≤π/6ax+bif π/6<x≤1
The values of a and b such that f and f' are continuous, areAa=1,b=1/√2+π/6Ba=1/√2,b=1/√2Ca=1,b=√3/2−π/6Dnone of these
Let f(x) be defined on [-2,2] and is given by
f(x)={−1−2≤x≤0x−10<x≤2
and g(x)=f(|x|)+|f(x)|. Then g(x) is equal to
View SolutionIf f(x)=ax2+bx+c is such that |f(0)|≤1,|f(1)|≤1and|f(−1)|≤1, prove that |f(x)|≤5/4,∀x∈[−1,1]
View SolutionLet f(x) be defined on [-2,2[ such that f(x)={−1−2≤x≤0x−10≤x≤2 and g(x)=f(|x|)+|f(x)|. Then g(x) is differentiable in the interval.
View SolutionIf f:{x:−1≤x≤1}→{x:−1≤x≤1}, then which is/are bijective ?
View SolutionLet f be a function on R given by f(x) = x2 and let E = {x∈R:−1≤x≤0}andF={x∈R,0≤x≤1}
then which of the following is fase ?
View Solution
