Let BC be a fixed line segment in the plane . The locus of a point A such that the triangle ABC is isosceles , is (with finitely many possible exceptional points )

A

a line

B

a circle

C

the union of a circle and a line

D

the union of two circles and a line

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:D

Case (i) :
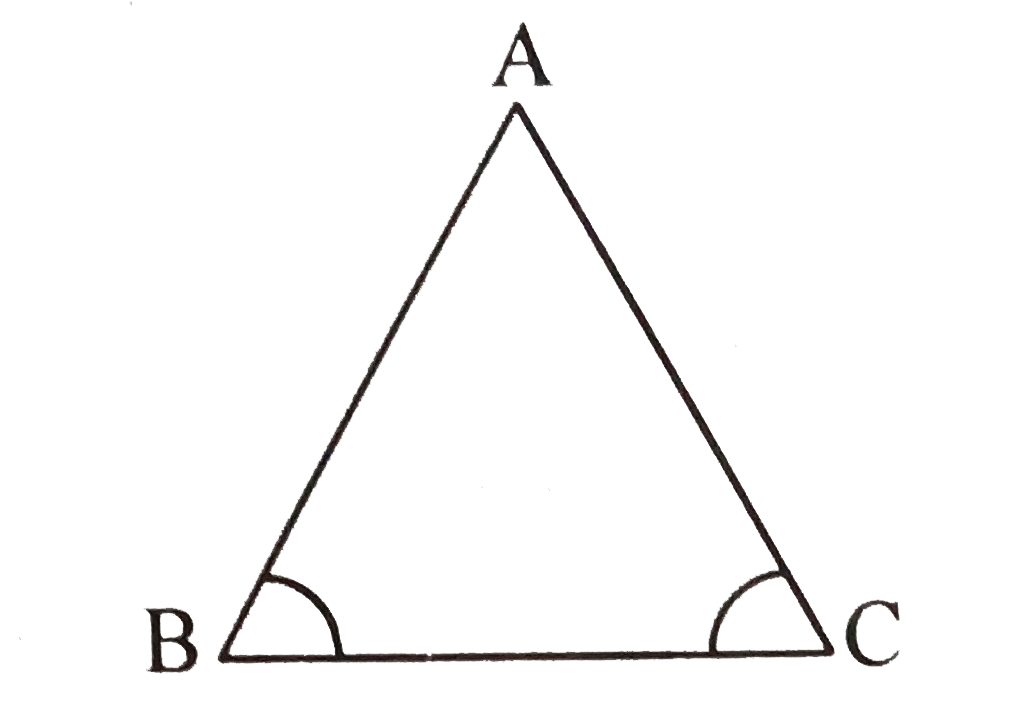
ocr_image
If ∠B=∠C
locus of A is ⊥ bisector of BC
So it is straight line
Case (ii) :
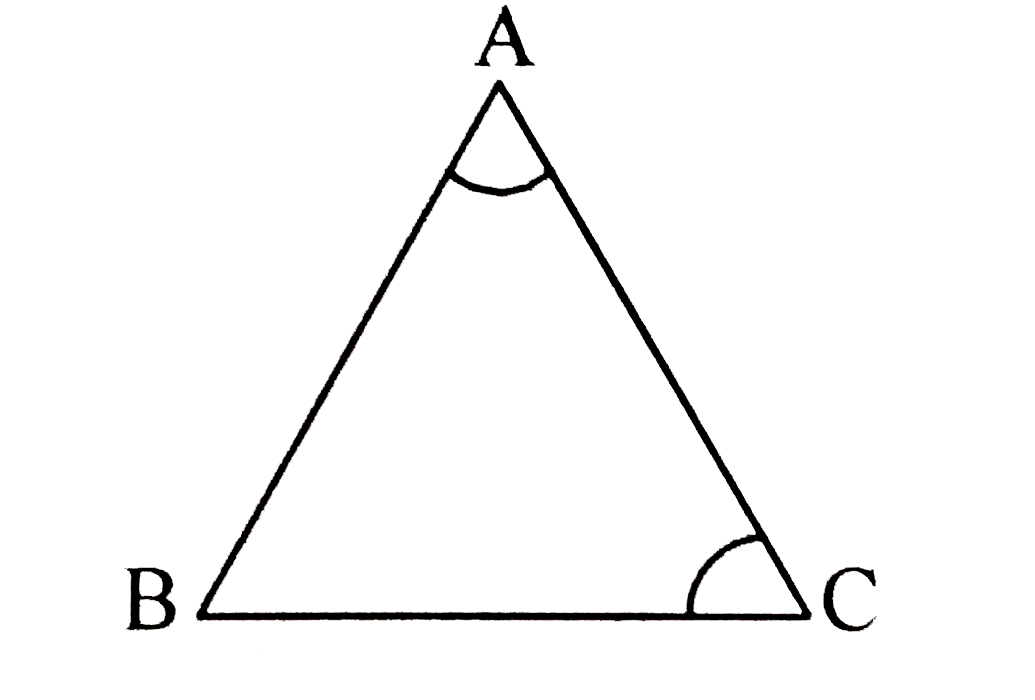
ocr_image
If ∠A=∠C
BC fixed B (a , 0 ) , C(0, a)
BC = AB
So , (x−a)2+y2=2a2
Circle
Case (iii) :
∠A=∠B
AC = BC
√h2+(k−a)2=√2a2
x2+(y−a)2=2a2
also a circle
So union of two circle and a line .

Updated on:21/07/2023
|
Class 12MATHSQUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Let A=(4,0),B=(0,12) be two points in the plane. The locus of a point C such that the area of triangle ABC is 18 sq. units is -

    A(y+3x+12)2=81
    B(y+3x+81)2=12
    C(y+3x−12)2=81
    D(y+3x−81)2=12

  • Suppose BC is a given line segment in the plane and T is a scalene triangle. The number of points A in the plane such that the triangle with vertices A,B,C (in some order) is similar to triangle T is

    A4
    B6
    C12
    D24

  • Let ABC be a triangle having its centroid at G. If S is any point in the plane of the triangle then SA + SB + SC is equal to

    ASG
    B2SG
    C3SG
    D0