[Tamil] Let E(1)={x in R :x ne 1 and (x)/(x-1) gt 0} and E(2)={x in E(

Let $f$ be a real-valued invertible function such that $f (\frac{- 32 x}{x - 2}) = 5 x - 2, x \neq 2 .$ Then value of $f^{- 1} (13)$ is________

02:12

View Solution

Let $a, b, c, d$ be positive integers such that ${(log)}_{a} b = \frac{3}{2} a n d {(log)}_{c} d = \frac{5}{4} .$ If $(a - c) = 9,$ then find the value of $(b - d) .$

04:41

View Solution

Number of integral value(s) of $λ$ for which vectors $x^{2} ˆ i - ˆ j + x ˆ k, (λ - 1) ˆ i - 2 λ ˆ j + ˆ k$ and $ˆ i - ˆ j + ˆ k$ , in the order from right-handed system $\forall x$ $\in$ R, is

View Solution

If log_(2)^(x)<=0 then log_((1)/(pi)){sin^(-1)((2x)/(1+x^(2)))+2Tan^(-1)x}=

02:00

View Solution

Consider f:(0,oo)rarr(-(pi)/(2),(pi)/(2)), defined as f(x)=tan^(-1)((log_(e)x)/((log_(e)x)^(2)+1))* The about function can be classified as

02:37

View Solution

Let $E_{1} = {x \in R : x \neq 1 and \frac{x}{x - 1} > 0} and E_{2} = {x \in E_{1} : {sin}^{- 1} ({log}_{e} (\frac{x}{x - 1})) is real number}$
(Here, the inverse trigonometric function ${sin}^{- 1} x$ assumes values in $[- \frac{π}{2}, \frac{π}{2}]$ .)
Let $f : E_{1} \to R$ be the function defined by $f (x) = {log}_{e} (\frac{x}{x - 1}) and g : E_{2} \to R$ be the function defined by $g (x) = {sin}^{- 1} ({log}_{e} (\frac{x}{x - 1})) .$
ocr_image
The correct option is

10:52

View Solution

Let $f (x) = {log}_{e} | x - 1 |, x \neq 1$ , then the value of $f' (\frac{1}{2})$ is

11:44

View Solution

If $f (x) = {cos}^{- 1} {\frac{1 - {({log}_{e} x)}^{2}}{1 + {({log}_{e} x)}^{2}}}$ , then f'( e )

View Solution

माना की $E_{1} = {x \in R : x \neq 1 और \frac{x}{x - 1} > 0}$ और $E_{2} = {x \in E_{1} {sin}^{- 1} ({log}_{e} (\frac{x}{x - 1})}$ एक वास्तविक संख्या (real number) है|
(यहाँ प्रतिलोम त्रिकोणमितीय फलां (inverse trignometric function) ${sin}^{- 1} x, [- \frac{π}{2}, \frac{π}{2}]$ में मान धारण करता है|
माना की फलन: $f : E_{1} \to R, f (x) = {log}_{e} (\frac{x}{x - 1})$ के द्वारा परिभाषित है
और फलन $f : E_{2} \to R, g (x) = {sin}^{- 1} l {log}_{e} (\frac{x}{x - 1})$ के द्वारा परिभाषित है|
ocr_image
दिए हुए विकल्पों में से सही विकल्प है

04:02

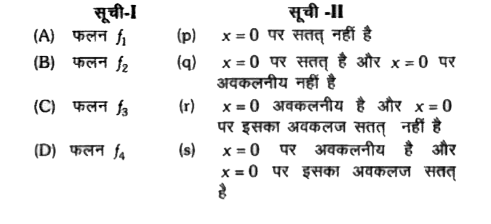
View Solution

माना कि फलन
$f_{1} : R \to R, f_{2} : (- \frac{π}{2}, \frac{π}{2}) \to R, f_{3} : (- 1, e^{\frac{π}{2}} - 2) \to R$ और $f_{4} : R \to R$ इस प्रकार परिभाषित है कि
(i) $f_{1} (x) = sin (\sqrt{1 - e^{- x^{2}}})$ ,
(ii) $f_{2} (x) = {\begin{matrix} \frac{| sin x |}{{tan}^{- 1} x} if & x \neq 0 1 & if x = 0 \end{matrix}$ जहां प्रतिलोम त्रिकोणमितीय फलन ${tan}^{- 1} x, (- \frac{π}{2}, \frac{π}{2})$ में मान धारण करता है
(iii) $f_{3} (x) = [sin ({log}_{e} (x + 2))]$ , जहां $t ε R$ के‌ लिए [t],t से छोटा या t के बराबर महत्तम पूर्णांक को दर्शाता है।
(iv) f_(4)(x)={(x^(2)sin(1/x),यदि x!=0),(x,यदिx=0):}
ocr_image

View Solution

The set of all real values of x for which $f (x) = {log}_{e} \sqrt{\frac{1 + x}{1 - x}} + {log}_{e} (\frac{1 + \sqrt{1 - x^{2}}}{x}) + {coth}^{- 1} x + {log}_{e} (\frac{1 + \sqrt{1 + x^{2}}}{x}) + {sinh}^{- 1} x$ is defined is