Let f:(2,∞)→X be defined by f(x)=4x−x2. Then f is invertible, if X=
A
[2,∞]
B
(−∞,2]
C
(−∞,4]
D
[4,∞)
Video Solution
Text Solution
The correct Answer is:C
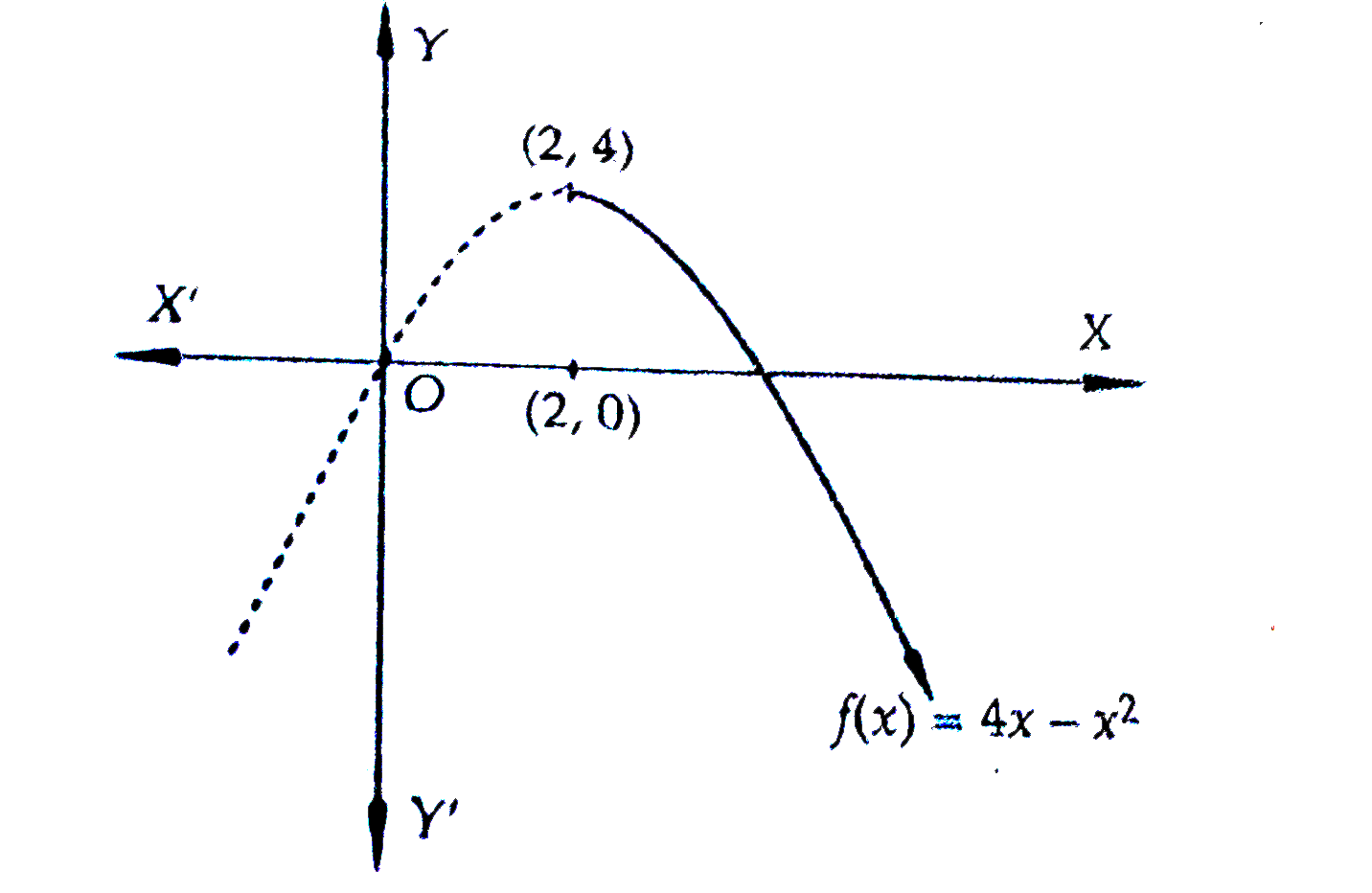
It is evident from the graph of f(x) that is represents an are of the parabola y=4x−x2 lying on the right side of the vertex (2,4). Clearly, range of f=(−∞,4)
Hence, X=(−∞,4)
|
Topper's Solved these Questions
- FUNCTIONSBOOK - OBJECTIVE RD SHARMACHAPTER - FUNCTIONSEXERCISE - Section II - Assertion Reason Type
10 Videos
- FUNCTIONSBOOK - OBJECTIVE RD SHARMACHAPTER - FUNCTIONSEXERCISE - Exercise
48 Videos
- FUNCTIONSBOOK - OBJECTIVE RD SHARMACHAPTER - FUNCTIONSEXERCISE - Chapter Test
30 Videos
- DISCRETE PROBABILITY DISTRIBUTIONSBOOK - OBJECTIVE RD SHARMACHAPTER - DISCRETE PROBABILITY DISTRIBUTIONSEXERCISE - Exercise
40 Videos
- HYPERBOLABOOK - OBJECTIVE RD SHARMACHAPTER - HYPERBOLAEXERCISE - Chapter Test
29 Videos
Similar Questions
Knowledge Check
Let f:(−∞,2]→(−∞,4] be a function defined by f(x)=4x−x2. Then, f−1(x) is
A2−√4−xB2+√4−xC2±√4−xDnot definedLet f:[4,∞)→[4,∞) be defined by f(x)=5x(x−4).Then f−1(x) is
A2−√4−logsxB2+√4+logsxC(15)xx+4Dnot defined
Let f:R→R be defined by f(x) = cos (5x+2). Is f invertible? Justify your answer.
View SolutionLet f:R→R be defined by f(x)=cos(5x+2). If f invertible ?
View SolutionLet f:R→R be defined by f(x)=(ex−e−x)/2. Is f(x) invertible? If so, find is inverse.
View SolutionLet f: R→R be defined by f(x)=3x+4,X∈R Is f invertible ? If so, give a formula for f−1.
View SolutionLet f: R→R be defined by f(x)=3x+4,X∈R Is f invertible ? If so, give a formula for f−1.
View SolutionLet f:R→R be defined by f(x)=cos(5x+2). Is f invertible? Justify your answer.
View Solution
