Question

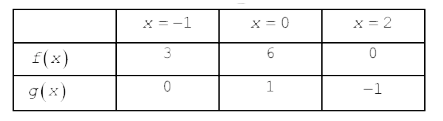
Let $f,g:[-1,2]\overrightarrow{R}$
be continuous functions which are twice differentiable on the interval $(-1,2)\stackrel{.}{}$
Let the values of $fandg$
at the points $-1,0and2$
be as given in the following table:
, $x=-1$
, $x=0$
, $x=2$

$f\left(x\right)$
, 3, 6, 0
$g\left(x\right)$
, 0, 1, $-1$

In each of the intervals $(-1,0)and(0,2)$
the function $(f-3g)\text{'}\text{'}$
never vanishes. Then the correct statement(s) is (are)
$f^{\prime }\left(x\right)-3g^{\prime }\left(x\right)=0$
has exactly three solutions in $(-1,0)\cup (0,2)\stackrel{.}{}$

$f^{\prime }\left(x\right)-3g^{\prime }\left(x\right)=0$
has exactly one solutions in $(-1,0)\stackrel{.}{}$

$f^{\prime }\left(x\right)-3g^{\prime }\left(x\right)=0$
has exactly one solutions in $(-1,2)\stackrel{.}{}$

$f^{\prime }\left(x\right)-3g^{\prime }\left(x\right)=0$
has exactly two solutions in $(-1,0)$
and exactly two solutions in $(0,2)\stackrel{.}{}$

Options

A. `f'(x)-3g,(x)=0` has exctly three solution in `(-1, 0) uu (0,2)`
B. `f(x)-3g'(x)`=0 has exactly one solution in (-1,0)
C. f'(x)-3g'(x)=0 has exactly one solution in (0,2)
D. f'(x)-3g'(x)=0 has exactly one solution in (-1,0) and exactly one solution in (0,2)

Answer

D

Explanation

Let `F(x)=f(x)-3g(x) "for all " x in [-1,2]` We have,
`F(-1)=f(-1)-3g(-1)=3-3xx0=3`
`F(0)=f(0)-3g(0)=6-3xx1=3`
`F(2)=f(2)-3g(2)=0-3xx(-1)=3`
By Rolle's theorem, F'(x) vanishes at least once in each of the interva [-1,0] and [0,2]
Now, `F,(x)=f(x)=-3 g(x)`
`rArr F'(x)=f''(x)-3g''(x)=(f''-3g'')(x)=(f-3g)''(x)`
It is given that `(f-3g)''(x)i.e.,F'' (x)` never vanishes. Therefore, `F''(x)gt0 or, F'' (x) lt0 "for all" x in (-1,0) uu(0,2)`
`rArr F'(x)` is either increasing or decreasing in (-1,0( and (0,2) .
`rArr F'(x)` 0 has exactly on solution in (-1,0) and one solution in (0,2)
`rArr f'(x)-3g(x)=0` has exctly one solution in (-1,0)n adn exactly one solution in (0,2)