Let f(x)=ax3+bx2+cx+1 has exterma at x=α,β such that αβ<0andf(α)f(β)<0 f . Then the equation f(x)=0 has (a)three equal real roots (b)one negative root if f(α) < 0andf(β) >0 (c)one positive root if f(α) < 0andf(β) >0 (d) none of these
three distinct real roots
one positive root if f(α)<0andf(β)>0
on negative root if f(α)>0 and (β)<0
all the above
The correct Answer is:D
We have ,
αβ<0
⇒αandβ are of opposite signs
Let α<0andβ>0
If is given that f(x) has extrema at x=α,β Therefore αandβ are two distinct real roots of f' (x)=0 .But we know that between two distinct roots a polynominal there is at least one real root of its derivative. Therefore,f(x) has three distinct real roots γ,μ and v (say) such that
γ<α<μ<β<v
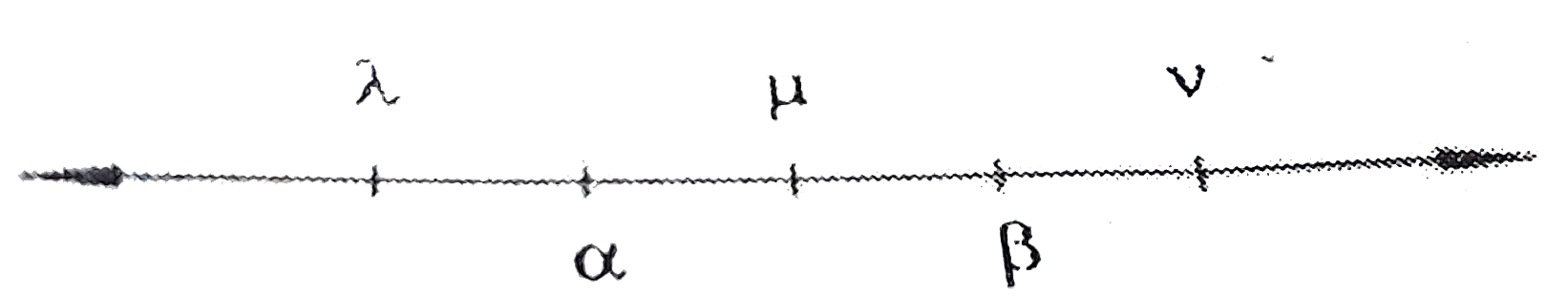
Thus,option (a)is correct.
If f(x)=0 has exactly one positive root, then it is evident from Fig .23 that v>0andγ,μ<0
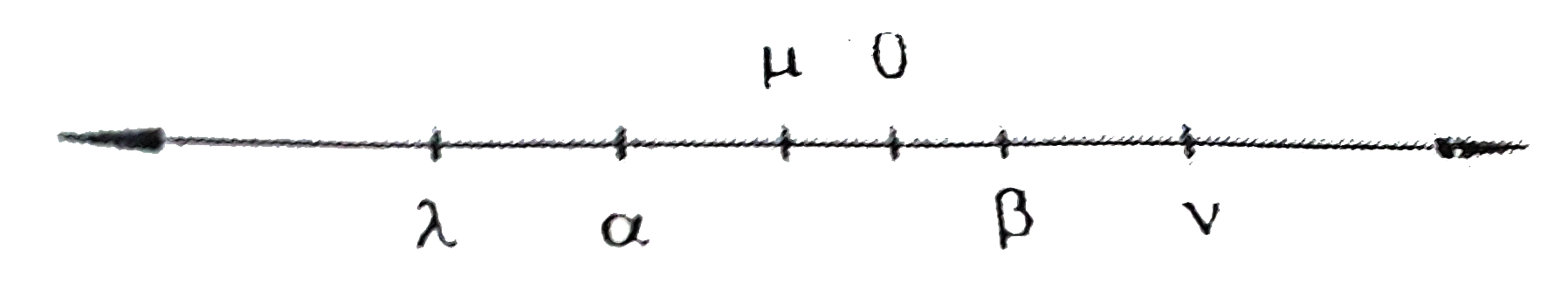
Therefore
α<μ<0
⇒f(α)f(0)<0 [∵μ lies between alpha and 0]
⇒f(α)<0 [∵f(0)=1<0]
⇒(α)>0 [∵f(α)f(β)<0] ltbtgt Thus option (b) is correct
If f(x) =0 has exactly one negative real root , then from Fig , 24 we have
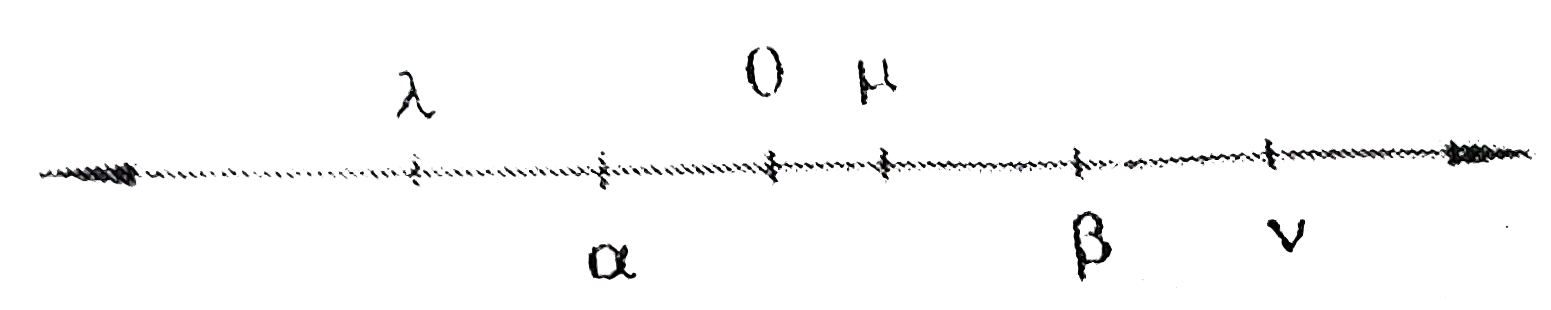
γ<0andμv>0
⇒0<μ<β
Topper's Solved these Questions
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Section II - Assertion Reason Type7videos
MAXIMA AND MINIMA
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Exercise47videos
MAXIMA AND MINIMA
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Chapter Test29videos
MAXIMA AND MINIMA
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MATHEMATICAL REASONING EXERCISE - Chapter Test20videos
MATHEMATICAL REASONING
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test21videos
MEASURES OF CENTRAL TENDENCY
Similar Questions
ax2+bx+c=0(a>0), has two roots αandβ such α<−2andβ>2, then
View Solutionax2+bx+c=0(a>0), has two roots αandβ such α<−2andβ>2, then
View Solutionax2+bx+c=0(a>0), has two roots αandβ such α<−2andβ>2, then
View SolutionIf (x + 2)(x + 3b) = c has roots α,β , then the roots of (x+α)(x+β)+c=0 are
View SolutionIf f is a real valued function given by f(x)=27x3+1x3andα,β are roots of 3x+1x=2. Then, f(α)=f(β)=−9 (b) f(α)=10 (c) f(β)=−10 (d) none of these
View SolutionIf α,β are the roots of x2+x+1=0 then αβ+βα=
View SolutionLet f(x)=ax5+bx4+cx3+dx2+ex, where a,b,c,d,e in R and f(x)=0 has a positive root. α. Then,
View SolutionLet f(x)=ax2+bx+c, g(x)=ax2+qx+r, where a, b, c,q, r∈R and a<0. If α, β are the roots of f(x)=0 and α+δ, β+δ are the roots of g(x)=0, then
View SolutionIf αβ(α<β) are two distinct roots of the equation. ax2+bx+c=0, then
View SolutionIn the quadratic equation ax2+bx+c=0, if Δ=b2−4acandα+β,α2+β2,α3+β3 are in GP. where α,β are the roots of ax2+bx+c=0, then
View Solution

