Let $P$ be any moving point on the circle $x^{2} + y^{2} - 2 x = 1 . A B$ be the chord of contact of this point w.r.t. the circle $x^{2} + y^{2} - 2 x = 0$ . The locus of the circumcenter of triangle $C A B (C$ being the center of the circle) is $2 x^{2} + 2 y^{2} - 4 x + 1 = 0$ $x^{2} + y^{2} - 4 x + 2 = 0$ $x^{2} + y^{2} - 4 x + 1 = 0$ $2 x^{2} + 2 y^{2} - 4 x + 3 = 0$

Text Solution

Verified by Experts

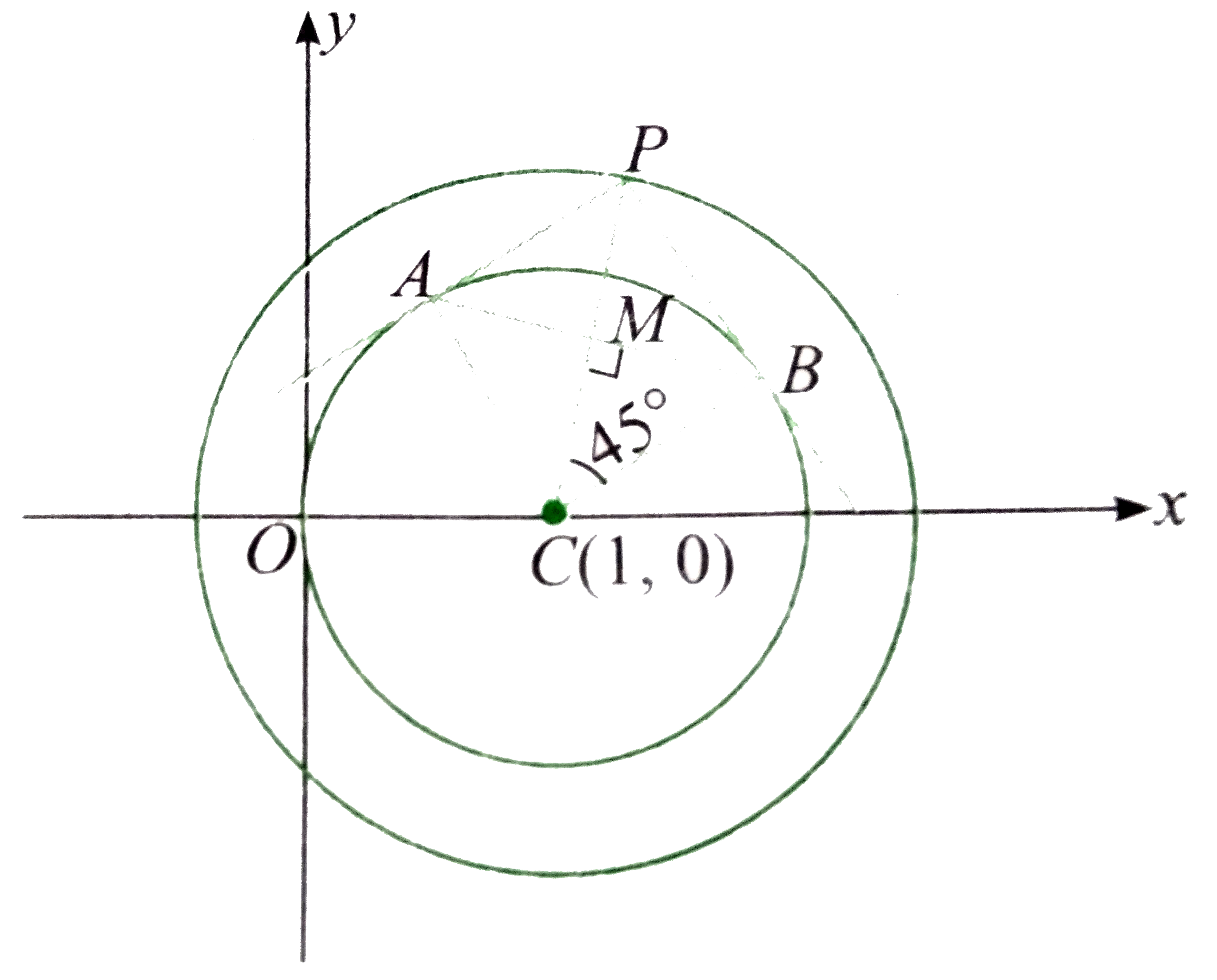
The two circles are
${(x - 1)}^{2} + y^{2} = 1$ (1)
${(x - 1)}^{2} + y^{2} = 2$ (2)
Clearly, the second circle is the director circle of the first circle.
$∴ ∠ A B = 90^{\circ}$
S, APBC is square.
Now, circumcentre of the right-angled triangle CAB will be midpoint of hypotenuse.
Let midpoint of AB be M(h,k).
Now, $C M = C B {sin 45}^{\circ} = \frac{1}{\sqrt{2}}$
So, ${(h - 1)}^{2} + k^{2} = \frac{1}{2}$
Hence, locus of M is ${(x - 1)}^{2} + y^{2} = \frac{1}{2}$

|

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

Knowledge Check

The length of the common chord of the circles $x^{2} + y^{2} + 2 x + 3 y + 1 = 0$ and $x^{2} + y^{2} + 4 x + 3 y + 2 = 0$ is
A $\frac{9}{2}$
B $2 \sqrt{2}$
C $3 \sqrt{2}$
D $\frac{3}{2}$

CENGAGE PUBLICATION-CIRCLE -Examples

Home
Ask Doubt
Profile