Let $S \equiv x^{2} + y^{2} + 2 g x + 2 f y + c =$ be a given circle. Find the locus of the foot of the perpendicular drawn from the origin upon any chord of S which subtends a right angle at the origin.

Text Solution

Verified by Experts

The equation of the given circle is
$x^{2} + y^{2} + 2 g x + 2 f y + c$ (1)
Let P(h,k) be the foot of perpendicular drawn from the origin to the chord LM of the circle S.
Slope, of $O P = \frac{k}{h}$
$∴$ Slope of $L M = - \frac{h}{k}$
Therefore, the equation of LM is
$y - k = - \frac{h}{k} (x - h)$
or $k y - k^{2} = - k x + h^{2}$
or $h x + k y = h^{2} = k^{2}$ (2)
Now, the combined equations of lines joining the points of intersection of (1) and (2) to the origin can be obtained by making (1) homogeneous with the helop of (2) as follows $,$
$x^{2} + y^{2} + (2 g x + 2 f y) (\frac{h x + k y}{h^{2} + k^{2}}) + c (\frac{h x_{k} y}{h^{2} + k^{2}}) = 0$
or ${(h^{2} + k^{2})}^{2} (x^{2} + y^{2}) + (h^{2} + k^{2}) (2 g x + 2 f y) (h x + k y) + c {(h x + k y)}^{2} = 0$ (3)

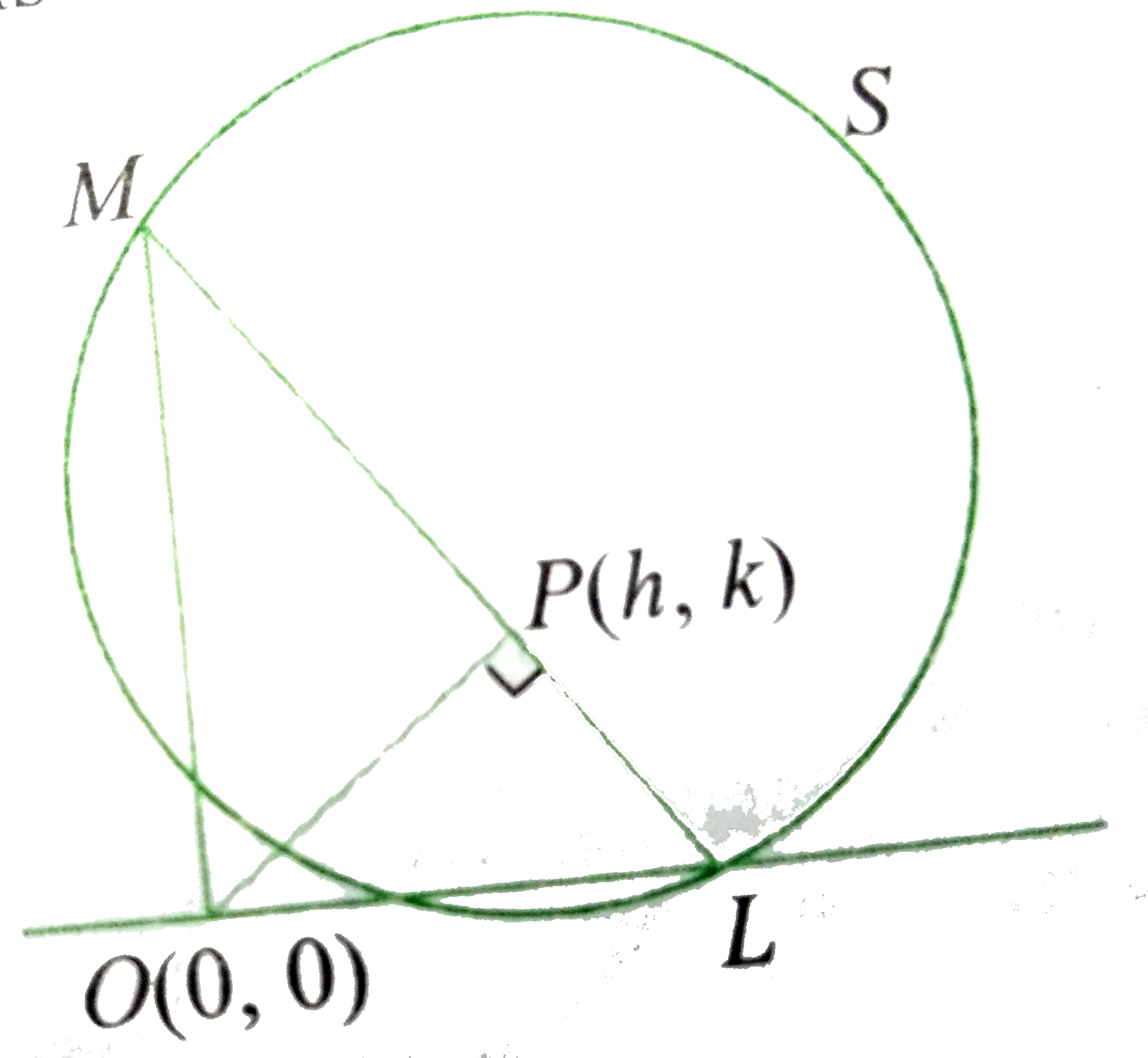
Equation (3) represents the combined equation of OL and OM.
But $∠ L O M = 90^{\circ}$ .
Therefore, from (3), we must have
Coeff. of $x^{2} +$ Coeff. of $y^{2} = 0$
or ${(h^{2} + k^{2})}^{2} + 2 g h (h^{2} + k^{2}) + c h^{2} + {(h^{2} + k^{2})}^{2} + 2 f k (h^{2} + k^{2}) + c k^{2} = 0$
or $h^{2} + k^{2} + g h + f k + \frac{c}{2} = 0$
Therefore, the locus of (h,k) is $x^{2} + y^{2} + g x + f y + \frac{c}{2} = 0$

The locus of the foot of the perpendicular drawn from the centre upon any tangent to the ellipse $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1$ , is
A ${(x^{2} + y^{2})}^{2} = a x^{2} + b y^{2}$
B $(x^{2} + y^{2}) = a^{2} x^{2} + b^{2} y^{2}$
C ${(x^{2} + y^{2})}^{2} = a^{2} x^{2} + b^{2} y^{2}$
DNone of the above
The locus of the midpoint of chord of the circle $x^{2} + y^{2} = 1$ which subtends a right angle at the origin is
A $x^{2} + y^{2} = \frac{1}{4}$
B $x^{2} + y^{2} = \frac{1}{2}$
C $x y = 0$
D $x^{2} - y^{2} = 0$