Let S1 be the sum of areas of the squares whose sides are parallel to coordinates axes
Let S2 be the sum of areas of the slanted squares as shown in the figure. Then S1/S2 is
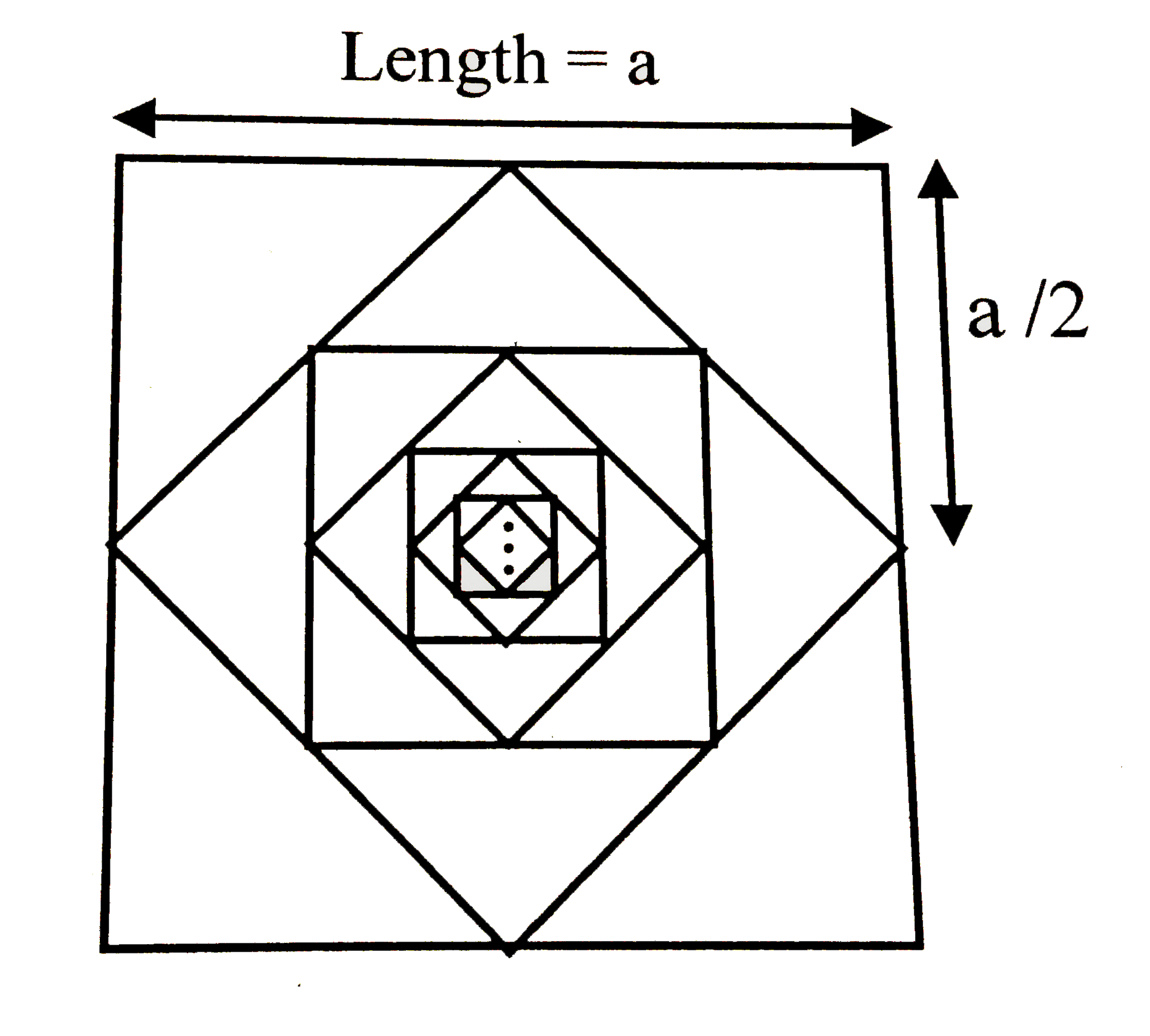
2
√2
1
1√2
The correct Answer is:a
S1=a2+a24+a216+......∞=a21−14=4a23
S2a22+a28+a232+......∞=a221−14=4a26
S1S2=2
Topper's Solved these Questions
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - Part 1 Mathematics
45 Videos
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - exercise
18 Videos
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - Part 2 Mathematics
15 Videos
- KVPY 2021BOOK - KVPY PREVIOUS YEARCHAPTER - KVPY 2021EXERCISE - PART II MATHEMATICS
4 Videos
Similar Questions
Knowledge Check
The sum of the squares of three distinct real
numbers which are in GP is S2 , if their sum is αS, thenA1<α2<3B13<α2<1C1<α<3D13<α<1Let A be the area of a square whose each side is 10 cm. Let B be the area of the square whose diagonals are 14 cm each. Then (A-B) is equal to
A0B1C2D43 point O(0,0),P(a,a2),Q(−b,b2)(a>0,b>0) are on the parabola y=x2. Let S1 be the area bounded by the line PQ and parabola let S2 be the area of the ΔOPQ, the minimum value of S1/S2 is
A2/3B5/3C2D73
Let S1 be the sum of first 2n terms of an arithmetic progression. Let S2 be the sum first 4n terms of the same arithmeti progression. If (S2−S1) is 1000, then the sum of the first 6n term of the arithmetic progression is equal to :
View SolutionThe sum of the areas of two squares is 157m2 . If the sum of their perimeters is 68 m , find the sides of the two squares .
View SolutionThe parabolas y2=4xandx2=4y divide the square region bounded by the lines x=4,y=4 and the coordinate axes. If S1,S2,S3 are the areas of these parts numbered from top to bottom, respectively, then S1:S2≡1:1 (b) S2:S3≡1:2 S1:S3≡1:1 (d) S1:(S1+S2)=1:2
View SolutionLet each side of smallest square of chess board in one unit in length. Find the sum of area of all possible squares whose side parallel to side of chess board.
View SolutionLet S1 be the sum of the first n terms of the A.P 8,12,16,... and let S2 be the sum of the first n terms of the A.P 17,19,21,... assume n≠0 then S1=S2 for
View Solution
