Make a conjuecture What do you think is true about the relationship between the number of vertices, edges and faces of a polyhedron ?
To solve the question regarding the conjecture about the relationship between the number of vertices, edges, and faces of a polyhedron, we can follow these steps:
1. Understand the Definitions:
- Vertices (V): The points where the edges meet.
- Edges (E): The line segments between the vertices.
- Faces (F): The flat surfaces that make up the polyhedron.
2. State Euler's Formula:
- Euler's formula for polyhedra states that:
V−E+F=2
- This means that the number of vertices minus the number of edges plus the number of faces equals 2.
3. Choose a Polyhedron:
- Let's take a square prism as an example.
- A square prism has:
- Vertices (V): 8 (4 from the top square and 4 from the bottom square)
- Edges (E): 12 (4 edges from the top square, 4 edges from the bottom square, and 4 vertical edges connecting the top and bottom squares)
- Faces (F): 6 (2 square faces and 4 rectangular lateral faces)
4. Calculate Using Euler's Formula:
- Substitute the values into Euler's formula:
V=8,E=12,F=6
- Plugging these values into the formula gives:
8−12+6=2
- This confirms that the formula holds true for the square prism.
5. Conjecture:
- Based on the example of the square prism and Euler's formula, we can conjecture that:
- For any polyhedron, the relationship between the number of vertices, edges, and faces will always satisfy Euler's formula:
V−E+F=2
- Vertices (V): The points where the edges meet.
- Edges (E): The line segments between the vertices.
- Faces (F): The flat surfaces that make up the polyhedron.
- Euler's formula for polyhedra states that:
V−E+F=2
- This means that the number of vertices minus the number of edges plus the number of faces equals 2.
- Let's take a square prism as an example.
- A square prism has:
- Vertices (V): 8 (4 from the top square and 4 from the bottom square)
- Edges (E): 12 (4 edges from the top square, 4 edges from the bottom square, and 4 vertical edges connecting the top and bottom squares)
- Faces (F): 6 (2 square faces and 4 rectangular lateral faces)
- Substitute the values into Euler's formula:
V=8,E=12,F=6
- Plugging these values into the formula gives:
8−12+6=2
- This confirms that the formula holds true for the square prism.
- Based on the example of the square prism and Euler's formula, we can conjecture that:
- For any polyhedron, the relationship between the number of vertices, edges, and faces will always satisfy Euler's formula:
V−E+F=2
Topper's Solved these Questions
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - THINK AND DISCUSS
18 Videos
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - EXERCISE
91 Videos
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - EXERCISE
91 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
Similar Questions
Knowledge Check
The number of vertices, edges and faces respectively of a cuboid are
A8, 6, 12B12, 8, 6C8, 12, 6D12, 6,8
What do you think a telebook is?
View SolutionWhat do you think a telebook is?
View SolutionWhat do you think is special about the composition of the poem?
View SolutionComplete the table for the number of vartices V, for edges E and for faces F each of the polyhedrons you made.
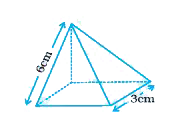
View SolutionDetermine the number of edges, vertices and faces in the fig.
View SolutionWhat do you think was Jo’s problem?
View SolutionWhat do you think are the limitations of nationalism?
View Solution
