Match the following lists and then choose the correct code.
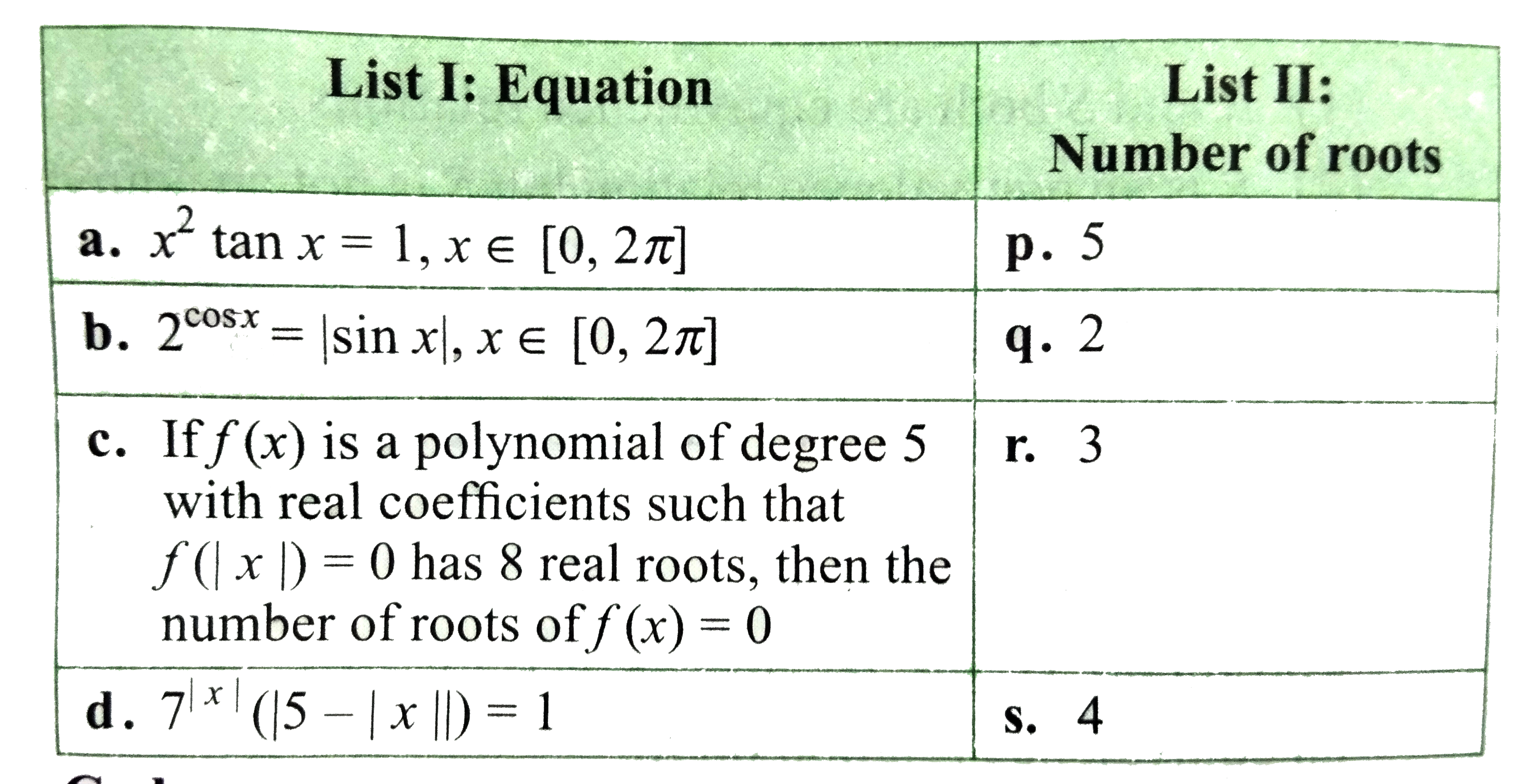
A
r,s,p,q
B
s,p,r,q
C
q,s,p,r
D
p,r,s,q
Text Solution
The correct Answer is:3
a. Let the length of the common chord be 2a. Then,
√9−a2+√16−a2=5
or √16−a2=5−√9−a2
or 16−a2=25+9−a2−10√9−a2
or 10√9−a2=18
or 100(9−a2)=324
i.e., 100a2=576
or a=√576100=2410
∴2a=245=k5or =24
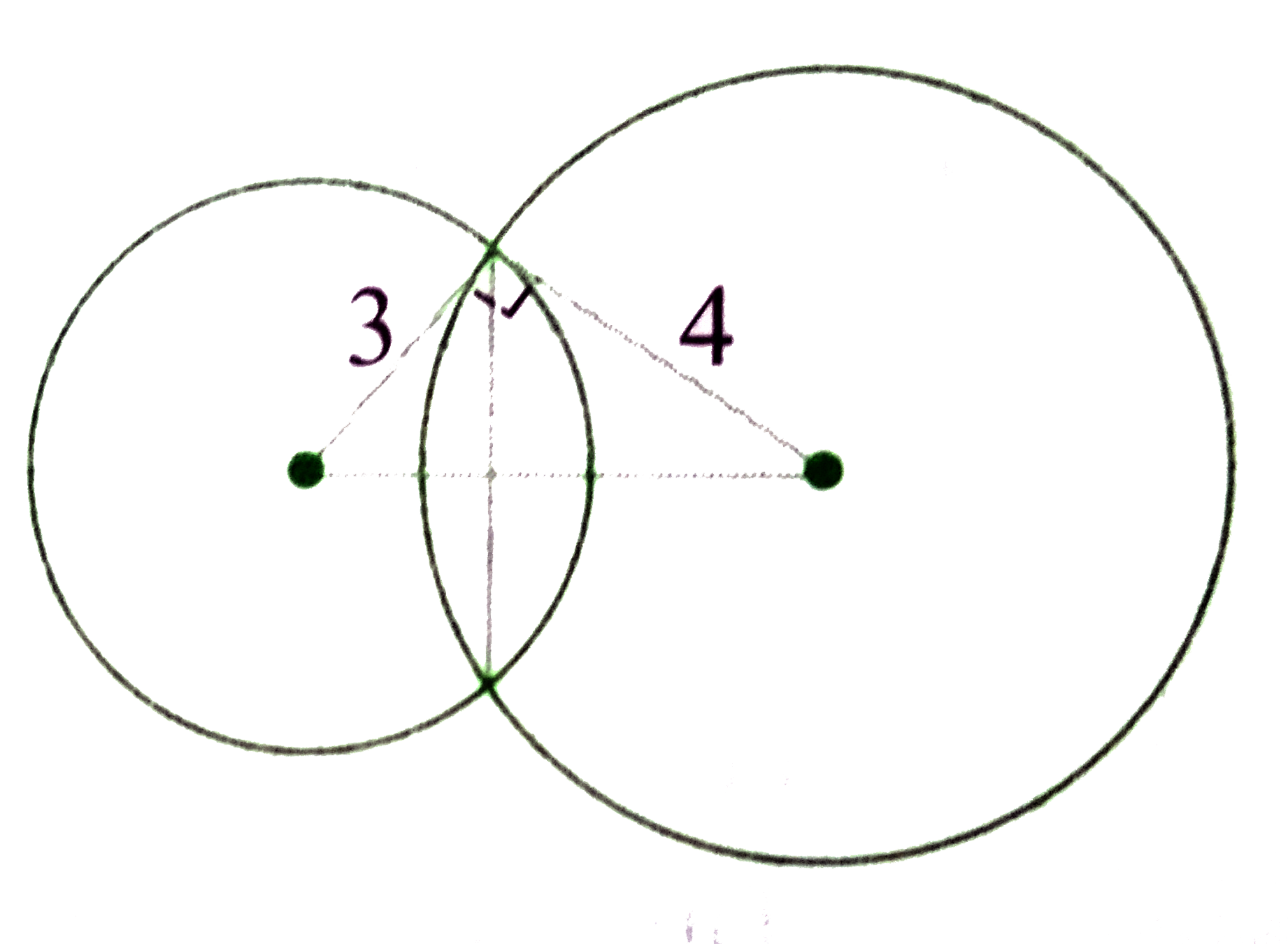
b. The equation of common chord is 6x+4y+p+q=0.
The common chord passes through the center (−2,−6) of the circle x2+y2+4x+12y+p=0 . Therefore,
p+q=36
c. The equation of the circle is 2x2+2y2−2√2x−y=0
Let (α,0) be the midpoint of a chord . Then the equation of the chord is
2αx−√2(x+α)−12(y+0)=2α2−2√2α
Since it passes through the point (√2,1/2) , we have
2√2α−√2(√2+α)−14=2α2−2√2α
i.e., 8α2−12√2α+9=0
i.e., (2√2α−3)2=0
i.e., α=32√2
Therefore, the number of chords is 1.
d. Midpoint of AB ≡(1,4).
The equation perpendicular bisector of AB is x=1
A diameter of the circle is 4y=x+7
Therefore, the center of the circle is (1,2)
Hence, the sides of the rectangle are 8 and 4. Therefore, area =32
|
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - NUMERICAL VALUE TYPE
21 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - ARCHIVES (JEE MAIN)
1 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - For Problems
43 Videos
- BINOMIAL THEOREMBOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
Similar Questions
Knowledge Check
Match the following lists and and then choose the correct code.

 AabcdprqqBabcdqprsCabcdspqrDabcdrsqp
AabcdprqqBabcdqprsCabcdspqrDabcdrsqpMatch the following two lists and choose the correct answer from the codes given below:
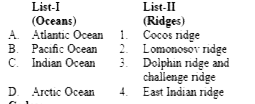 AA-2,B-4,C-1,D-3BA-3,B-1,C-4,D-2CA-1,B-2,C-3,D-4DA-4,B-3,C-2,D-1
AA-2,B-4,C-1,D-3BA-3,B-1,C-4,D-2CA-1,B-2,C-3,D-4DA-4,B-3,C-2,D-1Match the following two lists and choose the correct answer from the codes given below:
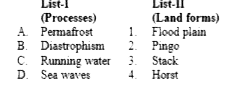 AA-2,B-4,C-1,D-3BA-2,B-1,C-4,D-3CA-1,B-2,C-3,D-4DA-4,B-3,C-2,D-1
AA-2,B-4,C-1,D-3BA-2,B-1,C-4,D-3CA-1,B-2,C-3,D-4DA-4,B-3,C-2,D-1
Match the following columns and choose the correct code.
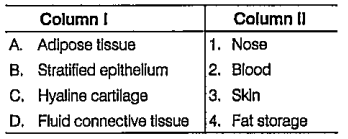
View SolutionList ‘A’ consists of the dynasties and List ‘B’ consists of their capital cities. Match the lists and choose the correct answer from the codes given below :

View SolutionMatch the following column and choose the correct code
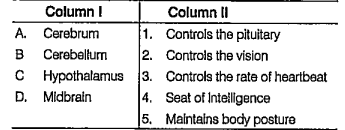
View SolutionMatch the relation for derivatives given in List II with the relation given in List I and then choose the correct code.

View SolutionMatch List I with List II and choose the correct code
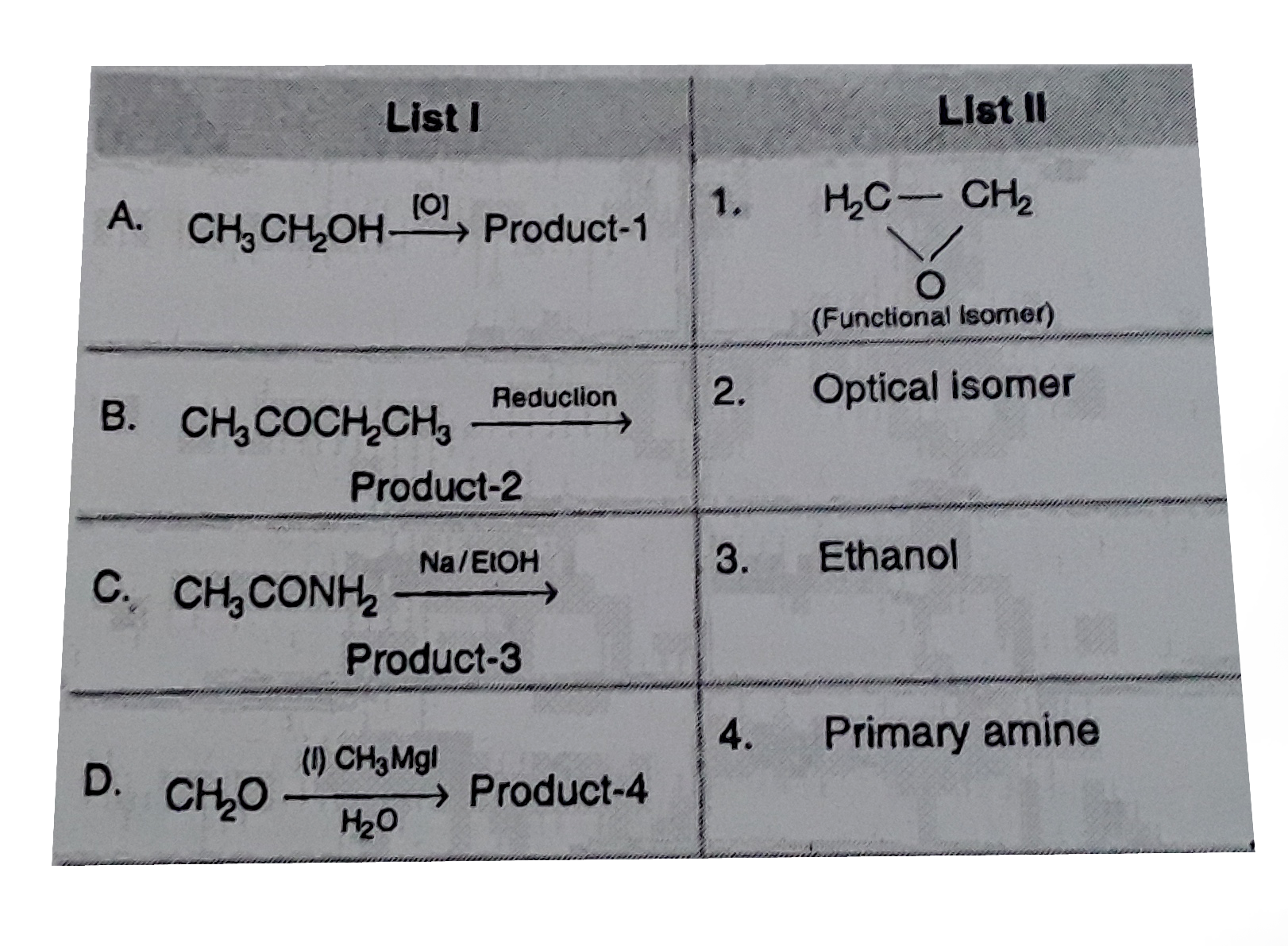
View Solution


