Match the following lists:

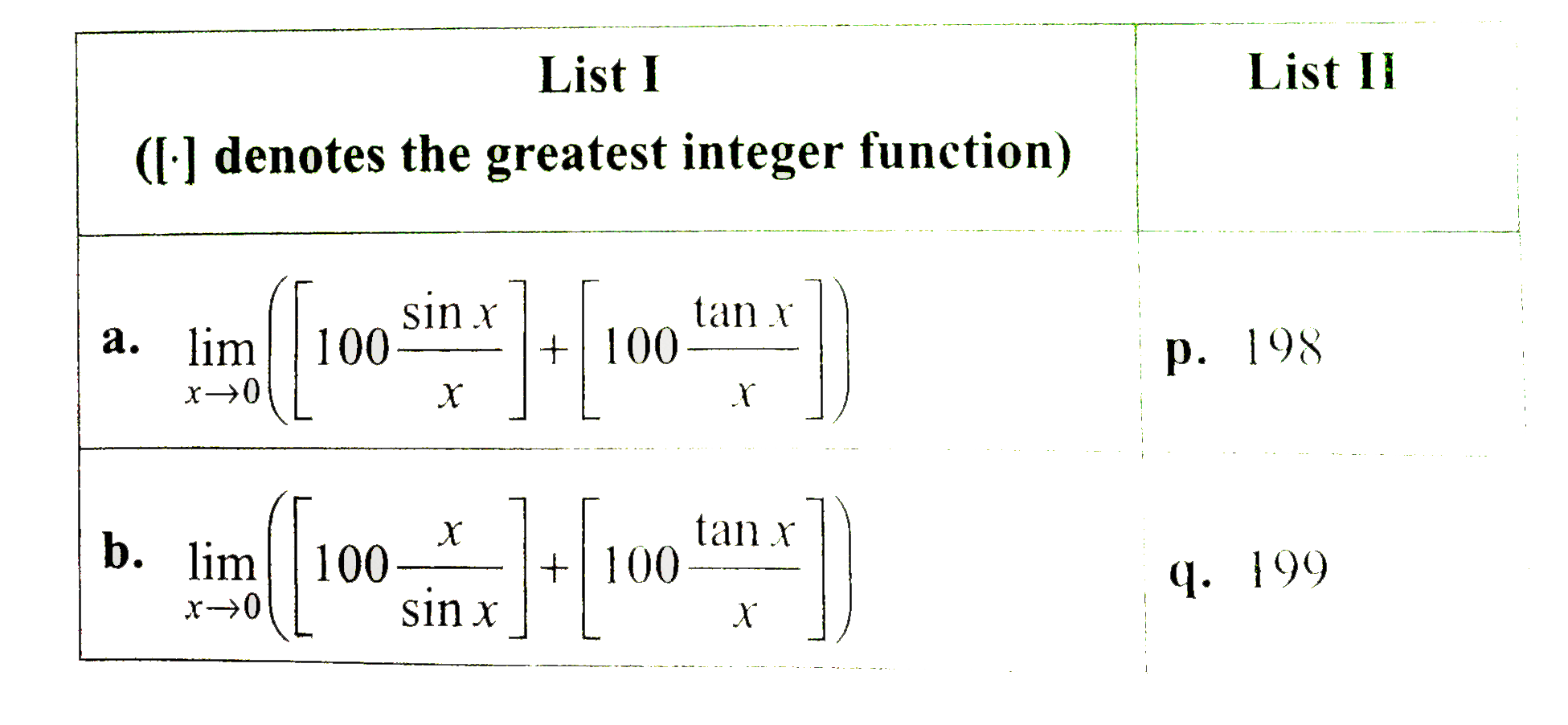
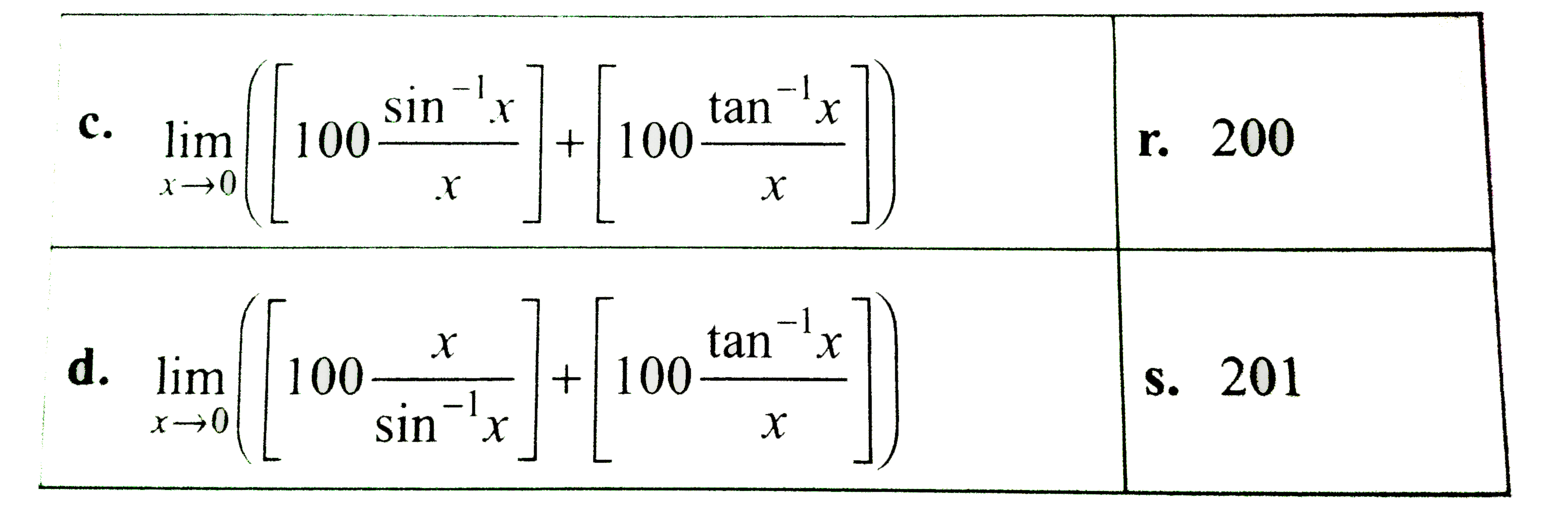
Text Solution

Verified by Experts

The correct Answer is: $a \to q; b \to r; c \to q; d \to p .$

We know that $lim x \to 0 \frac{sin x}{x} = 1$ (but a value is smaller than 1)
or $[lim x \to 0 100 \frac{sin x}{x}] = 99$
and $[lim x \to 0 100 \frac{x}{sin x}] = 100$
Also, $lim x \to 0 \frac{{sin}^{- 1} x}{x} = 1$ (but a value is more than 1)
or $[lim x \to 0 100 \frac{{sin}^{- 1} x}{x}] = 100$
and $[lim x \to 0 100 \frac{x}{{sin}^{- 1} x}] = 99$
$lim x \to 0 \frac{tan x}{x} = 1$ (but a value is bigger than 1)
or $[lim x \to 0 100 \frac{tan x}{x}] = 100$
and $[lim x \to 0 100 \frac{{tan}^{- 1} x}{x}] = 99$
Hence,
a. $lim x \to 0 ([100 \frac{sin x}{x}] + [100 \frac{tan x}{x}]) = 199$
b. $lim x \to 0 ([100 \frac{x}{sin x}] + [100 \frac{tan x}{x}]) = 200$
c. $lim x \to 0 ([100 \frac{{sin}^{- 1} x}{x}] + [100 \frac{{tan}^{- 1} x}{x}]) = 199$
d. $lim x \to 0 ([100 \frac{x}{{sin}^{- 1} x}] + [100 \frac{{tan}^{- 1} x}{x}]) = 198$

Updated on:21/07/2023

|

Class 12 MATHS LIMITS AND DERIVATIVES

Topper's Solved these Questions

CENGAGE-LIMITS-Exercise (Matrix)

Home
Ask Doubt
Profile